I have a system, consisting of one differential equation and one algebraic equation
req = (1 - xD)/τD - δxD xD ν;
ν = νmax (2/(1 + Exp[(-2 (u - u0))/kv]) - 1);
eqh = u == Istim + gE ν (xD - 0.5);
τD = 2; δxD = 0.01; νmax = 100; u0 = 150; kv = 20; \
Istim = 126; gE = 5;
eqs1 = xD'[t] == (req /. {xD -> xD[t], u -> u[t]});
eqs2 = eqh /. {xD -> xD[t], u -> u[t]}
ics = xD[0] == 0.99;
I try to solve it with the next command:
eqsol = NDSolve[{eqs1, eqs2, ics}, {xD[t], u[t]}, {t, 0, 1}];
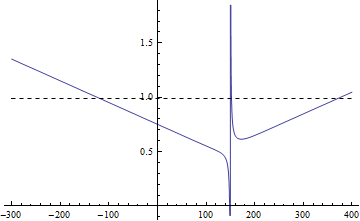
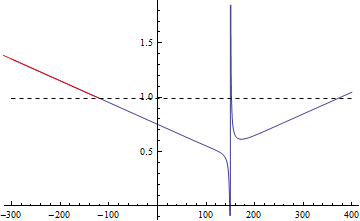
Then I want to make sure that this is correct solution, so I plot both variable xD[t] and u[t] and expect to find them on the curve defined by implicit equation from the system.
ParametricPlot[Evaluate[{u[t], xD[t]} /. eqsol], {t, 0, 1},
PlotRange -> Full]
I also can plot that curve by expressing the variable xD[t] as a function of the variable u: xD=xD(u).
xDimp = Solve[eqh, xD];
Plot[Evaluate[xD /. xDimp], {u, u0, 300}]
But I found that these curves don't coincide with each other. I suspect that the integration was executed wrong, but no errors were displayed during the integration. By observing the apt derivative I investigated that the function u(t) can be explicitly found from the implicit equation only for xD>0.54. This solution satisfies me. Due to this constraint I set the initial value as xD=0.99. But shall I define this constraint explicitly? Or else I don't know where the error can be.