0
$\begingroup$

I tried to define a partial differential operator using this code

x ∈ Reals && y ∈ Reals
μ ∈ Reals && ν ∈ Reals
z := x + I y

landau := (Laplacian[#, {x, y}] + 2*(μ + I ν) (z*D[#, z]) - 
    2*(μ - I ν) (Conjugate[z]*D[#, Conjugate[z]]) + 
    2 I ν - (μ^2 + ν^2)*(x^2 + y^2)) &

When I type

landau[f]

I got an error saying

General::ivar: "x+I y is not a valid variable." General::ivar: "Conjugate[x]-I\ Conjugate[y] is not a valid variable."

I think Mathematica don't support directly the usage of the standard operators

Cauchy operators

Is there a way to work directly withe complex coordinates, or do I have always to express differentiation in real coordinates. I will appreciate any other comment concerning my code.

Improve this question
$\endgroup$
3
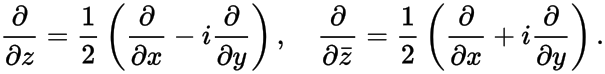
  • $\begingroup$ i believe you are correct about the differentiation, but also not your first "declaration" of the symbol "types" doesn't do anything, hence Conjugate[z] returns Conjugate[x] + I Conjugate[y] because Conjugate doesn't know x,y are real. You need to do $Assumptions={Element[x,Reals],..}, and then the assumptions are only applied by Simplify and the like. $\endgroup$
    – george2079
    Commented Apr 13, 2017 at 19:18
  • $\begingroup$ @george2079 Thank you, Can you help me to redefine D manually such that it supports the operators given above. Any insight will be helpful. $\endgroup$
    – Aymane Fihadi
    Commented Apr 14, 2017 at 10:35
  • $\begingroup$ @george2079 I posted that in separate question mathematica.stackexchange.com/questions/143583/… $\endgroup$
    – Aymane Fihadi
    Commented Apr 14, 2017 at 11:44

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.