How do we solve N-Rooks variation using primes?

Question

Using a $p_n $x $p_n$ matrix, how can we solve the N-Rooks problem to find a prime in every row and column?

Table[MatrixForm[Table[If[PrimeQ[n], "P", "."], {m, 0, Prime[o]^2 - Prime[o], 
Prime[o]}, {n, m + 1, m + Prime[o]}]], {o, 1, 8}]  

Here is the $11 $x$11$ matrix with the possible prime positions for the queens:

Note: single primes are always in the $p$-th column.

This is one possible solution (done by hand):

Edit Changed the title and link as Paxinum suggested.
OEIS A215637 has these counts of multiple solutions through $10th$ prime:
$$ 1, 1, 1, 2, 7, 72, 2144, 2641, 1345721, 2191254096$$

Answer 1

A very simple one, not very elegant :

f[o_] := Module[{mat, sol, vars, const, output}, 
  mat = Table[If[PrimeQ[n], Unique["p"], 0], {m, 0, Prime[o]^2 - Prime[o], 
    Prime[o]}, {n, m + 1, m + Prime[o]}];
  vars = Cases[Flatten[mat], _?(Not[NumericQ[#]] &)] ;
  const = Join[{Last[First[mat]] == 1}, Total[#] == 1 & /@ mat, 
    Total[#] == 1 & /@ Transpose[mat], 
    Thread[GreaterEqual[vars, 0]]];
  sol = FindInstance[const, vars, Integers];
  output = (mat /. First[sol])
]

f[8]/.{0 -> "."} //MatrixForm

Answer 2

Another possibility, at least for relatively small matrices, is to take the determinant (strictly speaking it is the permanent that is required, I suppose).

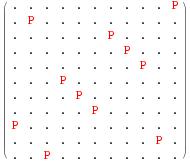
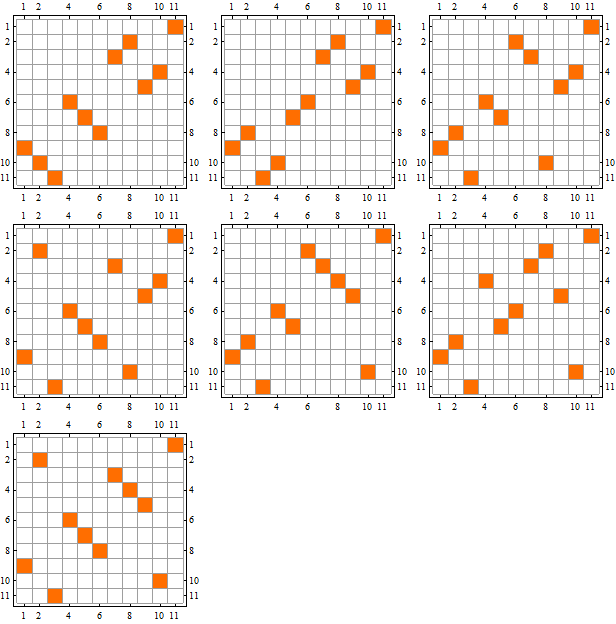
For example, for an $11 \times 11$ matrix (o=5), I find there are 7 solutions.

primePositions5 = 
  Position[With[{o = 5}, 
    Table[If[PrimeQ[n], 1, 0], {m, 0, Prime[o]^2 - Prime[o], 
      Prime[o]}, {n, m + 1, m + Prime[o]}]], 1];

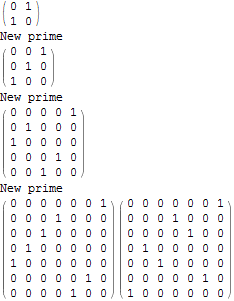
mylist = List @@ (Det@
     SparseArray[## -> Subscript[a, ##] & /@ 
       primePositions5] /. {-x_ -> x})

gives the following:

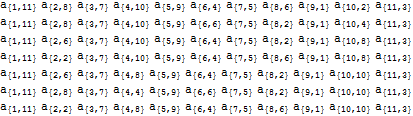
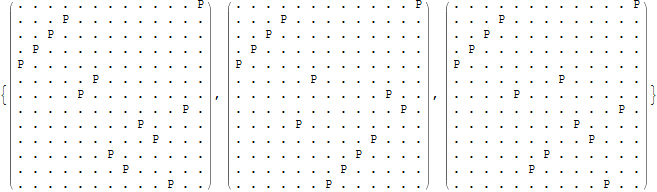
Matrix plots of all seven solutions:

MatrixPlot[
   Normal@SparseArray[(List @@ #) /. 
      Subscript[a, {x_, y_}] -> {x, y} -> 1], Mesh -> All, 
   ImageSize -> 200] & /@ mylist

I'll give then as a grid:

I reckon it needs to be emphasized that the Mathematica's Det command is slow.

With o=7 which gives a $17 \times 17$ matrix, I obtain 2144 solutions. For 0 =8 ($19 \times 19$), the figure is 2641. I could not go beyond this with the computer I am using (with Mathematica 7, as it so happens).

For o=4 ($7 \times 7$), I get two solutions:

Update for Mathematica 11

In Mma 11, we can use the Permanent function

myListAlt = List @@ (SparseArray[## -> Subscript[a, ##] & /@  primePositions5] // 
 Permanent // Expand)

The behaviour of Det seems to have changed somewhat since this question was posted.
I now need to Expand the result of the Det function:

mylist = List @@ (Expand@
 Det@SparseArray[## -> Subscript[a, ##] & /@ 
    primePositions5] /. {-x_ -> x})

and

mylist == myListAlt

True

Answer 3

This is neither elegant nor smart nor memory efficient. It is a brute force method to get all solutions of a given size

isGood[m_] := Sort@m === reye@Length@m;
i : reye[l_] := i = Reverse@IdentityMatrix@l;

getAllSolutions[n_?PrimeQ] := With[{id = IdentityMatrix@n},
     Pick[id, #, 1] & /@ Boole@PrimeQ@Partition[Range[n^2], n] // 
     Tuples]~Select~isGood;

So

Row[MatrixForm /@ #] & /@ 
  Composition[getAllSolutions, Prime]~Array~4 // 
 Column@Riffle[#, "New prime"] &

Gives

EDIT

I imagined that a solution along the lines of @bgatessucks 's great answer, but with booleans, would be more efficient and appropriate. However, while this is true for sizes below 13 (an order of magnitude faster in my tests), for some reason it suddenly becomes terribly slow afterwards.

v2[n_?PrimeQ, nsols_Integer: 1] := Module[{mat, vars},
  {mat, {vars}} = 
   Reap[PrimeQ@Partition[Range[n^2], n] /. True :> Sow@Unique["p"]];
  SatisfiabilityInstances[
    And @@ BooleanCountingFunction[{1}, n] @@@ 
      Join[mat, Transpose@mat], vars, nsols] /. 
   res_ :> (mat /. (Thread[vars -> #] & /@ res) /. {False -> ".", 
       True -> "P"})
  ]

Now

MatrixForm /@ v2[Prime@6, 3]

Gives