This question is based on the old song, The Twelve Days of Christmas
In a comment made on a recent question, Yves Klett remarked, "no seasonal questions this year?" This reminded me of one I solved six years ago. It's very easy, but has the virtue of giving a pretty expression for the general case.
Can you get Mathematica to tell you how many gifts True Love give over the 12 days of Christmas? Better yet, can you get a general expression for how many he would he give over a gift-giving period of n days?
I'm not going to post my answer unless no one posts an equivalent answer in the next 24 hours. I will be very surprised if that happens.
Update
My interpretation of the song is on each day True Love repeats each gift from the previous day and adds a new gift with multiplicity equal to the day index. That means 364 gifts for 12 days. I'm looking for the general formula for $n$ days. It's still an easy problem, but the general formula is rather nice.


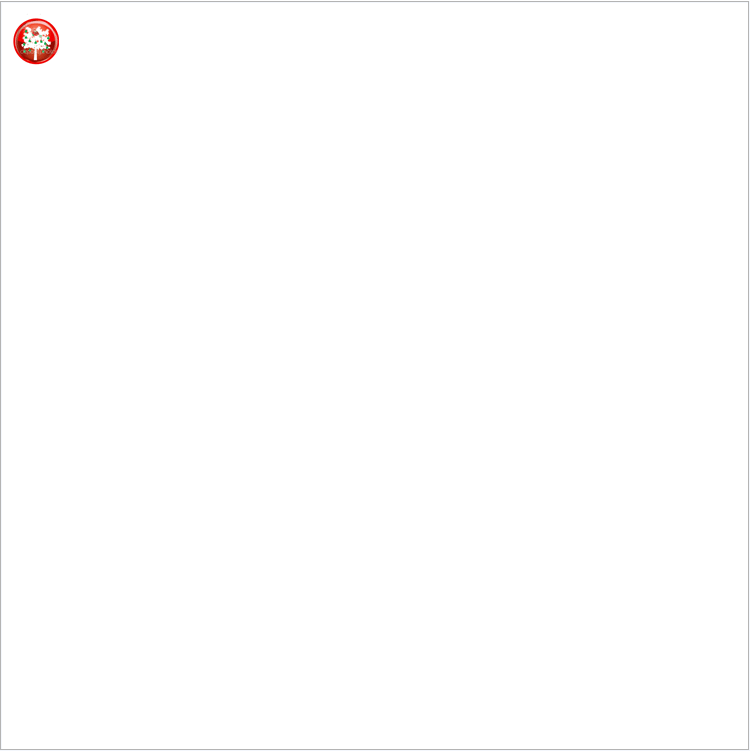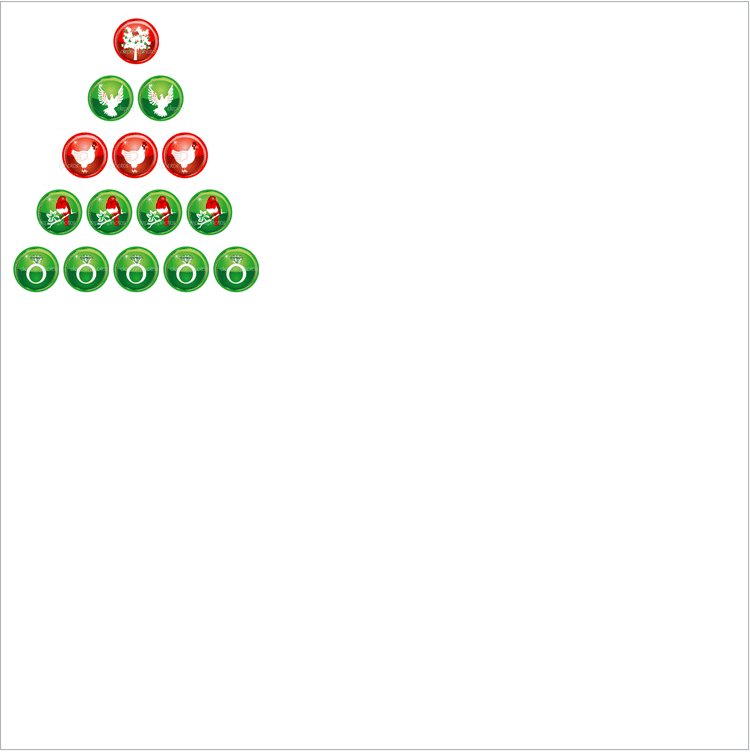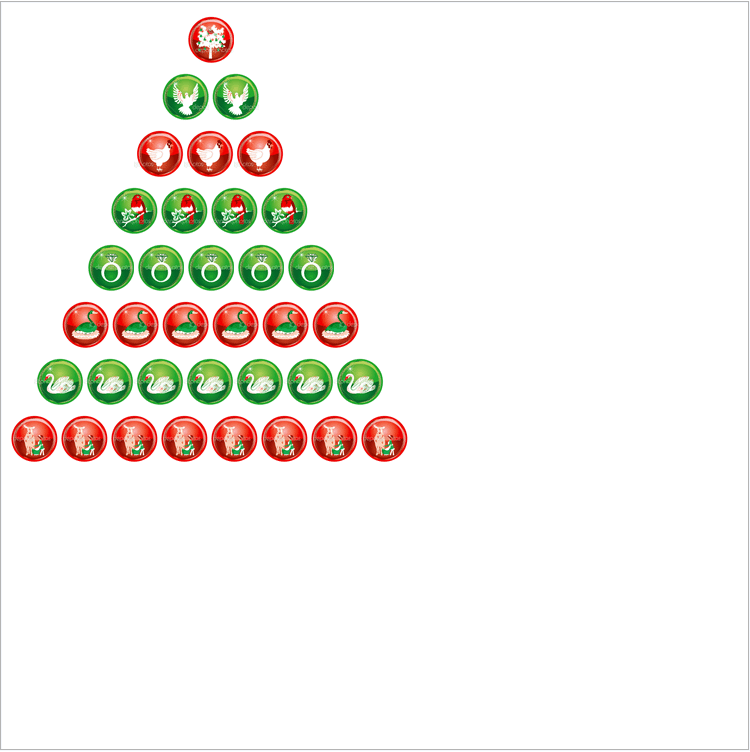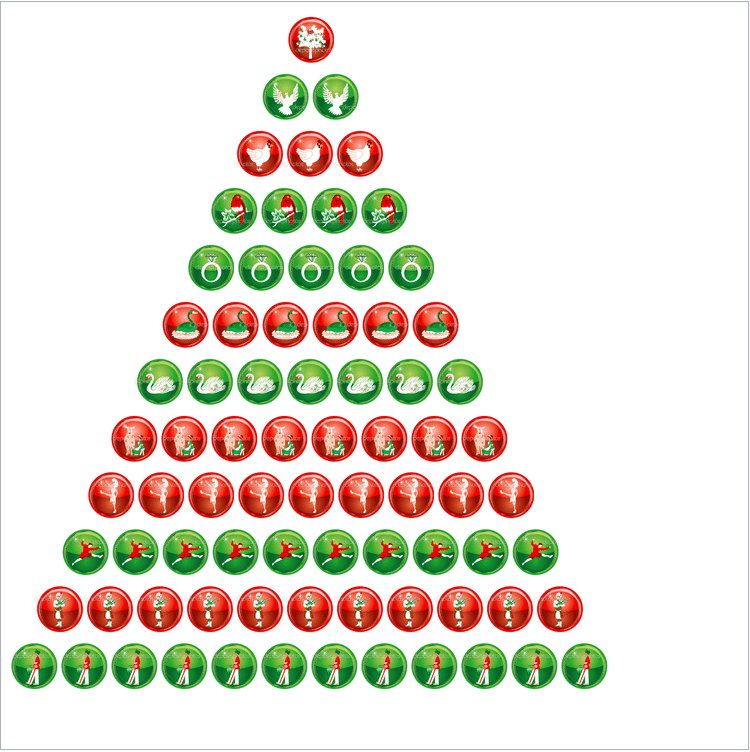

Sum[j, {i, n}, {j, i}]$\endgroup$(1/6)*n*(1 + n)*(2 + n)$\endgroup$