What is the algorithm to find the Up-sets of this set?

Question

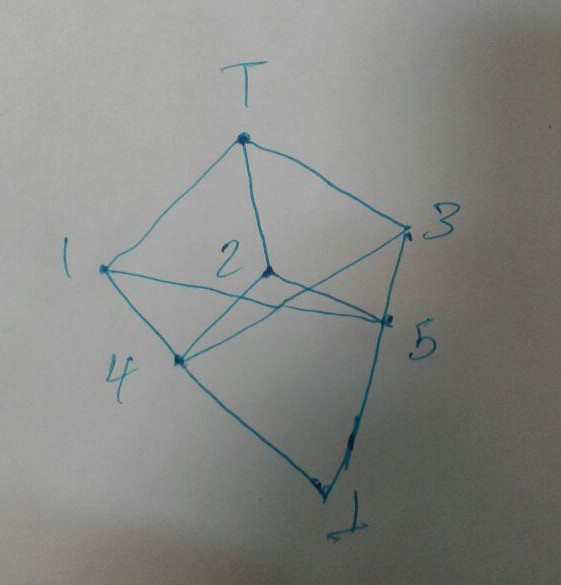
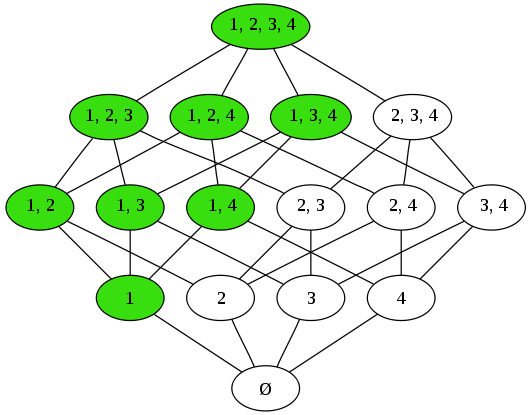
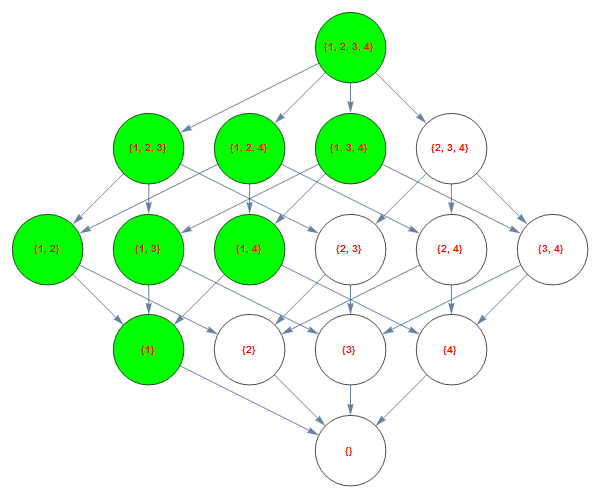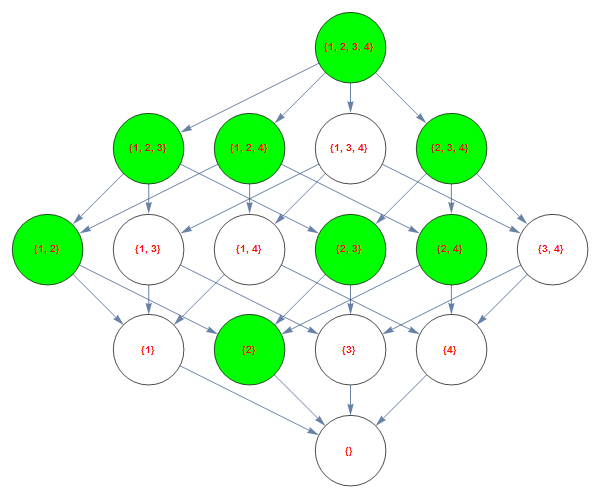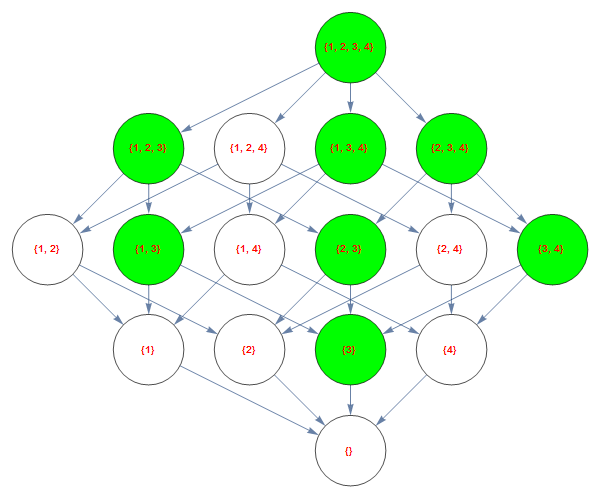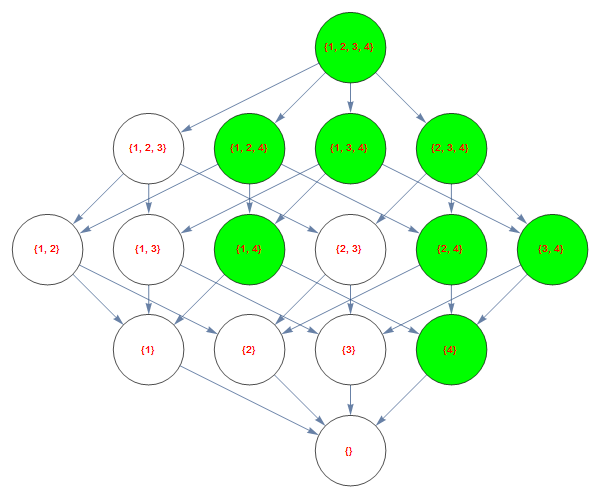
In my thesis, I need to find all Up-sets of the set in the picture below using the order provided in the picture. I tried to code this problem with Mathematica but I can't find a nice and optimal algorithm. A definition of Up-set is given in this link:https://en.wikipedia.org/wiki/Upper_set. For instance in the first picture, green set is an up-set. because "for any element in this set such as x, all element that such as y, such that x<= y are is this set". I need to coding this definition to find all up-set for an arbitrary set with an arbitrary order.

Answer 1

I'm not sure whether I fully interpreted your initial drawing and description correctly, but would the following work for you?

Your set as a Graph:

g = Graph[{"T", 1, 2, 3, 4, 5, "⊥"}, 
          {"T" -> 1, "T" -> 2, "T" -> 3, 4 -> 1, 4 -> 2, 5 -> 2, 4 -> 3, 
            5 -> 3, 5 -> 1, 4 -> "⊥", 5 -> "⊥"}, 
          VertexLabels -> Placed["Name", Center], 
          VertexLabelStyle -> Directive[FontSize -> 32], 
          VertexCoordinates -> {{245, 446}, {103, 316}, {268, 313}, {409, 354}, 
                                {177, 227}, {383, 264}, {323, 93}}
    ]

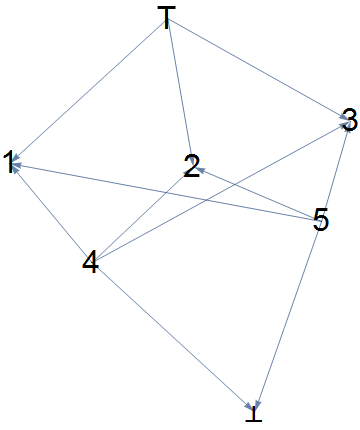
With this representation VertexComponent[g, #] & gives you the UpSet of the vertex you apply it on. So the lists of all UpSets is given by:

VertexComponent[g, #] & /@ VertexList[g]
(* {{"T"}, {1, "T", 4, 5}, {2, "T", 4, 5}, {3, "T", 4, 5}, {4}, {5}, {"⊥", 4, 5}} *)

Answer 2

set = Range[4]
subsets = Subsets[set];
rg = RelationGraph[SubsetQ[#1, #2] && Length[#1] == Length[#2] + 1 &, 
  subsets, VertexSize -> 0.7, VertexLabels -> Placed["Name", Center], 
  VertexLabelStyle -> Directive[Red, Bold], VertexStyle -> White]
upset[n_] := 
 Module[{sel = Select[VertexList[rg], MemberQ[#, n] &], 
   v = VertexList[rg], e = EdgeList[rg], com, h},
  com = Complement[v, sel];
  h = Thread[sel -> Green]~Join~Thread[com -> White];
  Graph[v, e, VertexCoordinates -> GraphEmbedding[rg], 
   VertexStyle -> h, VertexSize -> 0.7, 
   VertexLabels -> Placed["Name", Center], 
   VertexLabelStyle -> Directive[Red, Bold], ImageSize -> 600]
  ]

So finding upsets 1,2,3,4: