For example:
a = Range[20];
a = ArrayReshape[a, {4, 5}]
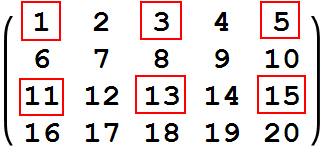
I want to take elements from a with interval = 2 :
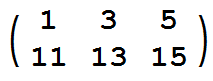
Take[a, {1, -1, 2}] fails to do this.
It would be better if it works for 3D or higher-dimensional large matrix.
$\begingroup$
$\endgroup$
3
1 Answer
$\begingroup$
$\endgroup$
3
That is precisely what Downsample is for:
a = Range[20];
a = ArrayReshape[a, {4, 5}];
Downsample[a, 2]
(* {{1, 3, 5}, {11, 13, 15}} *)
-
$\begingroup$ That's neat: I've learned a new function today! Would it be correct to say, however, that its functionality can be reproduced entirely by the use of Part, like @2012rchampion showed in the comment to the original question? For instance
a[[;; ;; 2, ;; ;; 3]] == Downsample[a, {2, 3} ]. Actually, usingPartseems to me slightly more flexible, because different offsets can be specified for each direction (e.g.a[[ hoff ;; ;; 2, voff ;; ;; 3 ]], but maybe I misunderstandDownsample. TheDownsamplesyntax is more readable than thePartspecification though. $\endgroup$– MarcoBCommented May 2, 2015 at 4:17 -
$\begingroup$ @Marc A quick test confirms that
offsetcan be a list:Downsample[Partition[Range[20], 5], {2, 3}, {1, 2}]gives the expected result. $\endgroup$ Commented May 2, 2015 at 20:01 -
$\begingroup$ @2012rcampion I see. Thanks for checking! $\endgroup$– MarcoBCommented May 3, 2015 at 13:38
Take[Partition[Range[20], 5], {1, -1, 2}, {1, -1, 2}]works, tho. Probably more generally:myTake[list_?ArrayQ] := Take[list, Sequence @@ ConstantArray[{1, -1, 2}, ArrayDepth[list]]]. (I don't have Mathematica on hand for testing.) $\endgroup$a[[;; ;;2, ;; ;; 2]](look upPartandSpan) $\endgroup$