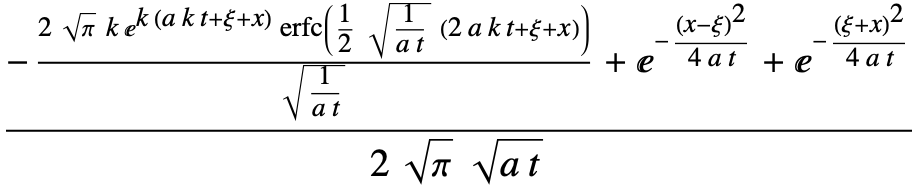How to use Mathematica to obtain the Green function for the third boundary value problem of the parabolic equation?

Question

I see that in the official documentation, there are only instructions for the first or second boundary value problems.

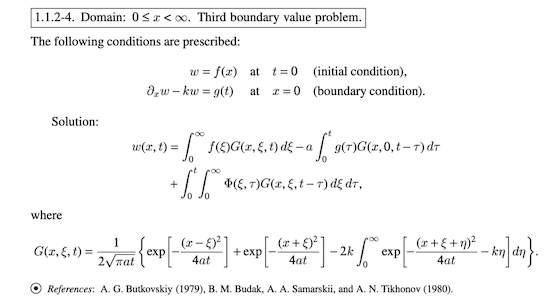
$\frac{\partial w}{\partial t}=a \frac{\partial^2 w}{\partial x^2}+\Phi(x, t)$ with Domain: $0 \leq x<\infty$. Third boundary value problem. The following conditions are prescribed: $$ \begin{array}{rlll} &w=f(x) &\text { at } t=0 \text { (initial condition), } \\ &\partial_x w-k w =g(t) \quad &\text { at } x=0 \text { (boundary condition). } \end{array} $$

Although the textbook provides the Green Function for this problem, I still want to know if Mathematica can solve this problem on its own?

For information on this issue in the textbook, please refer to the link: https://ibb.co/HV2JnS2

Answer 1

Not an answer but a comment. One can use mathematica to check that the solution provided from the PDF works.

Let us define

G[x_, \[Xi]_, t_] = 
 1/2/Sqrt[Pi  a  t] (Exp[-(x - \[Xi])^2/4/a/t] + 
    Exp[-(x + \[Xi])^2/4/a/t] - 
    2  k  Integrate[
      Exp[-(x + \[Xi] + \[Eta])^2/4/a/t - k  \[Eta] ], {\[Eta], 0, 
       Infinity}, GenerateConditions -> False])

We check that it satisfies the boundary conditions.

D[G[x, \[Xi], t], t] - a  D[G[x, \[Xi], t], x, x] // FullSimplify

(* 0 *)

D[G[x, \[Xi], t], x] - k  G[x, \[Xi], t] /. x -> 0 // FullSimplify

(*  0  *)

Now the full solution with a left hand side should be

ws[x_, t_] = 
 Integrate[f[\[Xi]] G[x, \[Xi], t], {\[Xi], 0, Infinity}] - 
  a  Integrate[g[\[Tau]] G[x, 0, t - \[Tau]], {\[Tau], 0, t}] + 
  Inactive@
   Integrate[\[CapitalPhi][x, \[Tau]] G[x, \[Xi], 
      t - \[Tau]], {\[Tau], 0, t},
    {\[Xi], 0, Infinity}]

Let us define the PDE it is supposed to satisfy

eqn = D[G[x, \[Xi], t], t] - 
   a  D[G[x, \[Xi], t], x, x] - \[CapitalPhi][x, t];

Indeed

eqn /. w -> Function[{x, t}, ws[x, t] // Rest // Evaluate] // Simplify

(* -[CapitalPhi](x,t) *)

Which may look incorrect at first but just reflects that the first term, if active, would actually return \[CapitalPhi](x,t) so that it cancels out to zero.

As a complement you might want to look at this answer