Let us suppose we have a coefficient vetor:
coeff = {a0, a1, b1, a2, b2, ..., an, bn}
with a total of (2*n + 1) elements. Those coefficients are applied in a Fourier series, such as:
I need to calculate both Jacobian (J) and Hessian (H) matrices from the coefficients, in the form:
Transpose[J] =
and
H =
both evaluated in a range of points x = {1, ... M}. Could anyone help me build the matrices J and H?

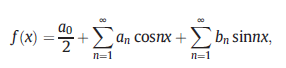
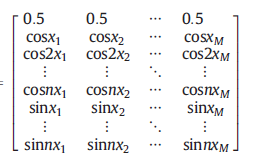
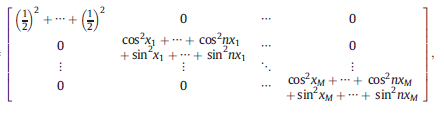
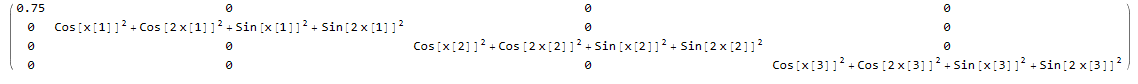
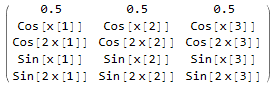
Transpose[J]to look like{0.5, Cos[1],...,Cos[n],Sin[1],...,Sin[n]}? $\endgroup$