If $ f(x)=x+6, h(x) = 6x-2$ and $g\circ f = h$, how can I solve for $g(x)$? I know how to do it on paper, but I wonder if Mathematica can do it in a general way. This doesn't work:
f[x_] := x + 6;
h[x_] := 6 x - 2;
Solve[ (g @* f )[x] == h[x], g]
$\begingroup$
$\endgroup$
2
1 Answer
$\begingroup$
$\endgroup$
Solve basically works on the level of symbolic algebra, so break down the composition in terms of substitution:
f[x_] := x + 6;
h[x_] := 6 x - 2;
g[u_] = g[u] /. First@Solve[{g[u] == h[x], u == f[x]}, g[u], {x}]
(* 2 (-19 + 3 u) *)
ClearAll[g];
g[f[x]] == h[x] // Simplify
(* True *)
Restriction: As @thorimur's comment implies, f must be invertible; further Mathematica has to be able to somehow solve for the inverse. Note in the above Solve command, f does not actually appear itself, just its value, so that solving for the inverse is merely a simple algebra problem. Yet, Mathematica can use InverseFunction:
Clear[f, g]
g[u_] = g[u] /. First@Solve[{g[u] == h[x], u == f[x]}, g[u], {x}]
Solve::ifun: Inverse functions are being used by Solve, so some solutions may not be found; use Reduce for complete solution information.
answered Jul 3, 2022 at 0:46
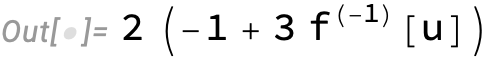
InverseFunctionin some way to express $h \circ f^{-1}$, or do something clever by somehow turning this into a differential equation forg. That would be cool. Of course both of these approaches have restrictions, and I'd be happy to see a more general approach. $\endgroup$Composition[Function[x, 6 x - 2], InverseFunction[Function[x, x + 6]]][x]$\endgroup$