As I noted in the comments, it seems unlikely that an analytic closed-form solution to this integral exists. However, if all you need is a numerical integral, then you can use the Region method to specify the region of integration.
PR[k_] := 2.1*10^-9 (k/0.05)^.04
k = 10^5;
1/12 NIntegrate[((4 v^2 - (1 + v^2 - u^2)^2)/(4 v u))^2 (3/( 4 u^3 v^3))^2 (u^2 + v^2 - 3)^2 ((-4 u v + (u^2 + v^2 - 3) Log[(3 - (u + v)^2)/(3 - (u - v)^2)])^2 + \[Pi]^2 (u^2 + v^2 - 3)^2 HeavisideTheta[v + u - Sqrt[3]]) PR[k u] PR[k v],
{u, v} \[Element] ImplicitRegion[Abs[1 - v] < u < Abs[1 + v], {u, v}]]
The method of specifying ranges of integration for u and v does not work in this case because the bounds with respect to u depend on the value of v, and NIntegrate does not allow the bounds of integration to depend on other variables when specified in this form. The use of ImplicitRegion gets around this restriction.
Alternately, if one defines $x = u + v$ and $y = u - v$, then the region of integration becomes $x \geq 1$ and $-1\leq y \leq 1$. Under the appropriate substitutions in the integrand, one could easily integrate this over the range {x, 1, Infinity} and {y, -1, 1}. Note that you will have to insert a Jacobian into the integral to account for the different volume element in these coordinates (I believe it works out to be 1/2 in this case.)
This said, I suspect that your integral may have an error. Specifically, the logarithm function looks strange; there are points in your range of integration for which its argument is positive, but also points for which its argument is negative. This yields a complex result in the end, and Mathematica is not confident in its results due to the resulting divergence of the integrand. Perhaps you need an absolute value on the argument?
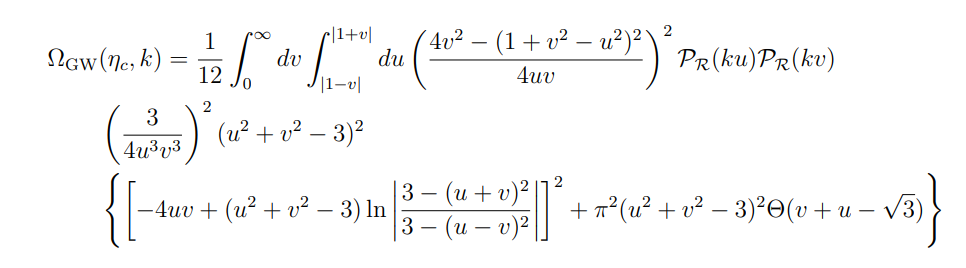


NIntegrateinstead? $\endgroup$NInegratedoes not supportAbs[x]in the limit of integrations. For exampleNIntegrate[Sin[x], {x, Abs[1 - x], Abs[1 + x]}]gives error. I never seen integral with such limits myself before. $\endgroup$Integrateit is not a good idea to use non-exact numbers. ` 2.1*10^-9 (k/0.05)^.04` you can change these to exact numbers. $\endgroup$NIntegrate[Sin[x y], {x, -10, 10}, {y, Abs[1 - x], Abs[1 + x]}], which works fine. Your example does not work because the limits of integration are non-numerical, not because they containAbs. $\endgroup$