I am trying to fit some data
data = {{100, 114}, {200, 1342}, {300, 36604}, {400, 44279}, {500,
10243}, {600, 4103}, {700, 3080}, {800, 2636}, {900, 2254}, {1000,
1909}, {1100, 1821}, {1200, 1620}, {1300, 1436}, {1400,
1319}, {1500, 1175}, {1600, 1158}, {1700, 1094}, {1800,
975}, {1900, 884}, {2000, 865}, {2100, 806}, {2200, 721}, {2300,
630}, {2400, 634}, {2500, 650}, {2600, 530}, {2700, 498}, {2800,
465}, {2900, 462}, {3000, 400}, {3100, 442}, {3200, 376}, {3300,
364}, {3400, 362}, {3500, 305}, {3600, 292}, {3700, 313}, {3800,
292}, {3900, 240}, {4000, 243}, {4100, 281}, {4200, 230}, {4300,
231}, {4400, 229}, {4500, 227}, {4600, 208}, {4700, 215}, {4800,
182}, {4900, 176}, {5000, 191}, {5100, 187}, {5200, 177}, {5300,
170}, {5400, 170}, {5500, 157}, {5600, 135}, {5700, 120}, {5800,
154}, {5900, 162}, {6000, 118}, {6100, 131}, {6200, 116}, {6300,
142}, {6400, 142}, {6500, 157}, {6600, 135}, {6700, 151}, {6800,
172}, {6900, 128}, {7000, 126}, {7100, 117}, {7200, 113}, {7300,
127}, {7400, 120}, {7500, 114}, {7600, 132}, {7700, 133}, {7800,
140}, {7900, 107}, {8000, 135}, {8100, 163}, {8200, 117}, {8300,
118}, {8400, 103}, {8500, 111}, {8600, 107}, {8700, 126}, {8800,
89}, {8900, 109}, {9000, 107}, {9100, 127}, {9200, 101}, {9300,
101}, {9400, 107}, {9500, 116}, {9600, 111}, {9700, 109}, {9800,
110}, {9900, 111}, {10000, 97}, {10100, 88}, {10200, 107}, {10300,
110}, {10400, 104}, {10500, 120}, {10600, 95}, {10700,
100}, {10800, 108}, {10900, 96}, {11000, 103}, {11100,
116}, {11200, 127}, {11300, 152}, {11400, 98}, {11500,
95}, {11600, 93}, {11700, 112}, {11800, 151}, {11900,
132}, {12000, 129}, {12100, 116}, {12200, 128}, {12300,
148}, {12400, 110}, {12500, 127}, {12600, 159}, {12700,
140}, {12800, 98}, {12900, 95}, {13000, 98}};
dataPlot = ListPlot[data, PlotRange -> All]
using a piecewise function consisting of a Gaussian function for $t < 600$ and an exponential function for $t \geq 600$. I've used FindFit and plotted the function on top of the data:
f2[t_, t0_, \[Sigma]_, t1_, \[Tau]_] :=
Piecewise[{{A Exp[-((t - t0)/(Sqrt[2] \[Sigma]))^2],
t < 600}, {B Exp[-(t - t1)/\[Tau]], t >= 600}}]
FindFit[data,
f2[t, t0, \[Sigma],
t1, \[Tau]], {{t0, 400}, {\[Sigma], 100}, {t1, 600}, {\[Tau],
1000}, {A, 45000}, {B, 4000}}, t,
NormFunction -> (Norm[#1, Infinity] & )]
f2Fit[t_] :=
Piecewise[{{45000*Exp[-((t - 360.003)/(Sqrt[2] 66.0556))^2],
t < 600}, {4000*Exp[-(t - 600)/1000], t >= 600}}]
fitPlot =
Plot[f2Fit[t], {t, 0, 13000}, PlotRange -> All,
PlotStyle -> {Red, Thick}];
Show[{dataPlot, fitPlot}]
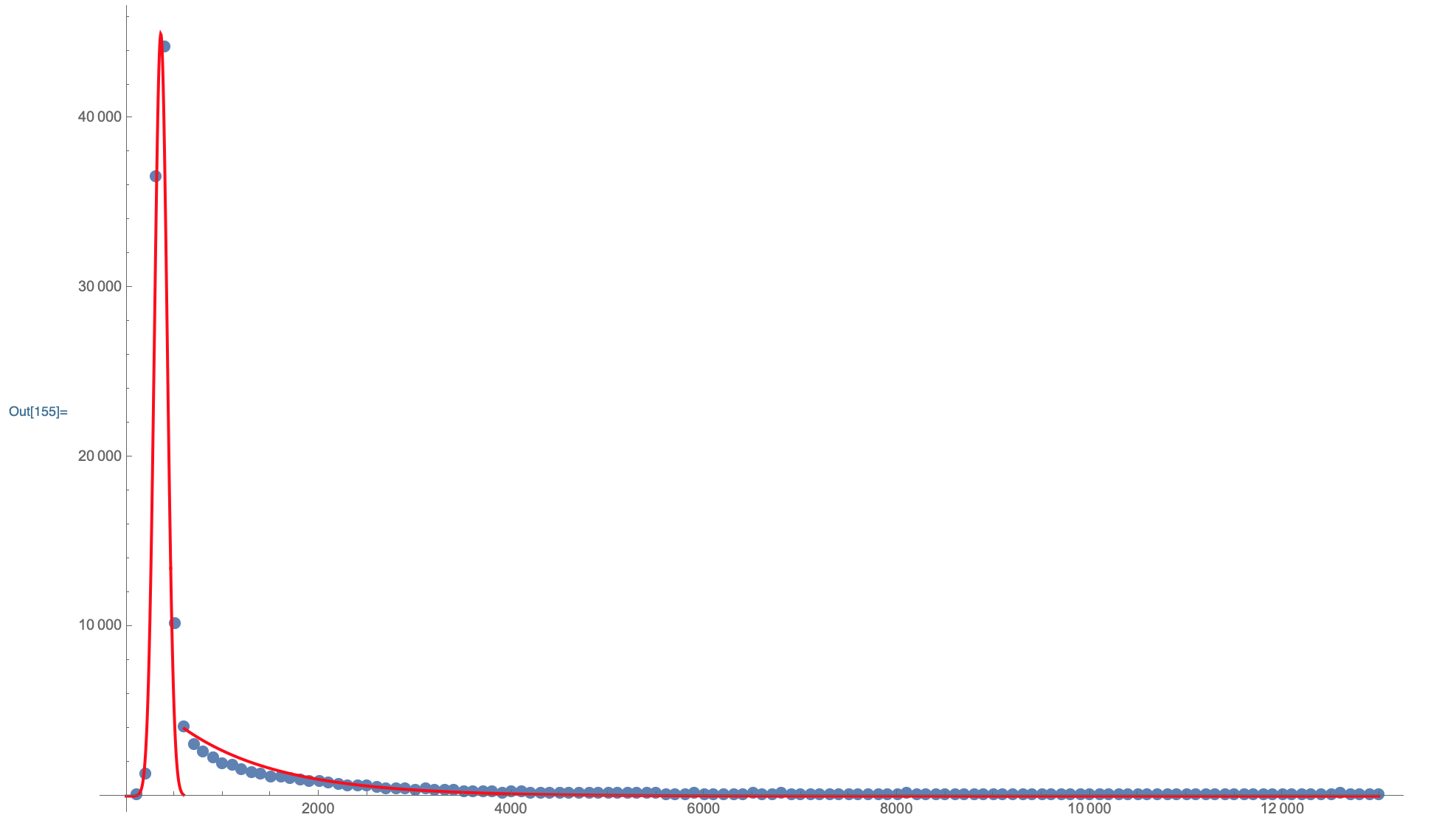 but I am having some trouble getting the function to be continuous. I have tried tweaking parameters of the fit but this is very time consuming and doesn't work well. Is there a way I can force the FindFit function to generate a continuous piecewise function? Or is there another routine I can use to generate such a function?
but I am having some trouble getting the function to be continuous. I have tried tweaking parameters of the fit but this is very time consuming and doesn't work well. Is there a way I can force the FindFit function to generate a continuous piecewise function? Or is there another routine I can use to generate such a function?




Reduceon that equality suggests that using $\tau=-\frac{2 \sigma ^2 (t_1-600)}{2 \sigma ^2 \log \left(\frac{B}{A}\right)+t_0^2-1200 t_0+360000}$ would do it. $\endgroup$