0
$\begingroup$

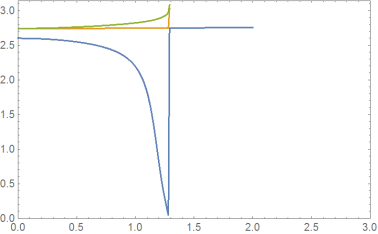
I have three lists (appropriately padded), giving me three curves, however the curves are not smooth/continuous and with gaps. I tried to make the grid finer, but still I can not get around this problem. This looks like a similar situation but it seems that it doesn't work for my problem. The data is

{{2.60012, 2.6001, 2.60004, 2.59994, 2.5998, 2.59962, 2.5994, 2.59914,
   2.59885, 2.59851, 2.59813, 2.59771, 2.59725, 2.59675, 2.59621, 
  2.59563, 2.59501, 2.59435, 2.59365, 2.59291, 2.59213, 2.59131, 
  2.59045, 2.58954, 2.5886, 2.58761, 2.58658, 2.58551, 2.5844, 
  2.58324, 2.58204, 2.5808, 2.57951, 2.57817, 2.57679, 2.57537, 
  2.57389, 2.57237, 2.5708, 2.56918, 2.56751, 2.56579, 2.56402, 
  2.56219, 2.56031, 2.55837, 2.55637, 2.55431, 2.55219, 2.55, 2.54775,
   2.54544, 2.54305, 2.54059, 2.53806, 2.53545, 2.53276, 2.52999, 
  2.52713, 2.52418, 2.52114, 2.51799, 2.51475, 2.5114, 2.50794, 
  2.50436, 2.50065, 2.49682, 2.49286, 2.48874, 2.48448, 2.48006, 
  2.47548, 2.47071, 2.46575, 2.4606, 2.45523, 2.44964, 2.4438, 
  2.43771, 2.43134, 2.42467, 2.41769, 2.41037, 2.40268, 2.39459, 
  2.38608, 2.3771, 2.36761, 2.35758, 2.34694, 2.33565, 2.32363, 
  2.31082, 2.29714, 2.28248, 2.26675, 2.24982, 2.23155, 2.21177, 
  2.19031, 2.16693, 2.14138, 2.11336, 2.08254, 2.04849, 2.01075, 
  1.96877, 1.92191, 1.86945, 1.81061, 1.74454, 1.67039, 1.58744, 
  1.49519, 1.39361, 1.28336, 1.16595, 1.04378, 0.919873, 0.79749, 
  0.679534, 0.568156, 0.464555, 0.369009, 0.281, 0.199296, 0.121749, 
  0.04388, 2.74842, 2.74852, 2.74862, 2.74872, 2.74882, 2.74892, 
  2.74902, 2.74912, 2.74922, 2.74932, 2.74942, 2.74952, 2.74962, 
  2.74972, 2.74982, 2.74992, 2.75003, 2.75013, 2.75023, 2.75033, 
  2.75043, 2.75053, 2.75063, 2.75073, 2.75084, 2.75094, 2.75104, 
  2.75114, 2.75124, 2.75134, 2.75144, 2.75154, 2.75164, 2.75174, 
  2.75183, 2.75193, 2.75203, 2.75213, 2.75222, 2.75232, 2.75242, 
  2.75251, 2.75261, 2.7527, 2.7528, 2.75289, 2.75298, 2.75308, 
  2.75317, 2.75326, 2.75335, 2.75344, 2.75353, 2.75361, 2.7537, 
  2.75379, 2.75387, 2.75396, 2.75404, 2.75413, 2.75421, 2.75429, 
  2.75437, 2.75445, 2.75453, 2.75461, 2.75469, 2.75476, 2.75484, 
  2.75491, 2.75498, 2.75506}, {Null, 2.74159, 2.74159, 2.7416, 2.7416,
   2.7416, 2.74161, 2.74161, 2.74162, 2.74163, 2.74163, 2.74164, 
  2.74165, 2.74166, 2.74167, 2.74169, 2.7417, 2.74171, 2.74173, 
  2.74174, 2.74176, 2.74178, 2.74179, 2.74181, 2.74183, 2.74185, 
  2.74187, 2.7419, 2.74192, 2.74194, 2.74197, 2.74199, 2.74202, 
  2.74205, 2.74207, 2.7421, 2.74213, 2.74216, 2.74219, 2.74223, 
  2.74226, 2.74229, 2.74233, 2.74236, 2.7424, 2.74244, 2.74248, 
  2.74251, 2.74255, 2.74259, 2.74264, 2.74268, 2.74272, 2.74277, 
  2.74281, 2.74286, 2.7429, 2.74295, 2.743, 2.74305, 2.7431, 2.74315, 
  2.7432, 2.74325, 2.74331, 2.74336, 2.74342, 2.74347, 2.74353, 
  2.74359, 2.74365, 2.7437, 2.74376, 2.74383, 2.74389, 2.74395, 
  2.74401, 2.74408, 2.74414, 2.74421, 2.74428, 2.74434, 2.74441, 
  2.74448, 2.74455, 2.74462, 2.74469, 2.74477, 2.74484, 2.74491, 
  2.74499, 2.74506, 2.74514, 2.74522, 2.74529, 2.74537, 2.74545, 
  2.74553, 2.74561, 2.74569, 2.74578, 2.74586, 2.74594, 2.74603, 
  2.74611, 2.7462, 2.74628, 2.74637, 2.74646, 2.74655, 2.74664, 
  2.74672, 2.74681, 2.74691, 2.747, 2.74709, 2.74718, 2.74727, 
  2.74737, 2.74746, 2.74755, 2.74765, 2.74774, 2.74784, 2.74794, 
  2.74803, 2.74813, 2.74823, 2.74833, 3.0303, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null}, {Null, 2.7416, 2.74163, 2.74168, 2.74174, 2.74183, 2.74193, 
  2.74206, 2.7422, 2.74236, 2.74254, 2.74273, 2.74295, 2.74318, 
  2.74343, 2.7437, 2.74399, 2.74429, 2.74461, 2.74494, 2.7453, 
  2.74567, 2.74605, 2.74645, 2.74687, 2.7473, 2.74775, 2.74821, 
  2.74869, 2.74918, 2.74969, 2.75021, 2.75074, 2.75129, 2.75185, 
  2.75243, 2.75301, 2.75362, 2.75423, 2.75486, 2.7555, 2.75615, 
  2.75681, 2.75749, 2.75818, 2.75888, 2.7596, 2.76032, 2.76106, 
  2.76181, 2.76258, 2.76335, 2.76414, 2.76494, 2.76575, 2.76658, 
  2.76741, 2.76826, 2.76913, 2.77, 2.77089, 2.77179, 2.7727, 2.77363, 
  2.77458, 2.77553, 2.7765, 2.77749, 2.77849, 2.77951, 2.78054, 
  2.78159, 2.78265, 2.78373, 2.78483, 2.78595, 2.78709, 2.78824, 
  2.78942, 2.79062, 2.79184, 2.79308, 2.79434, 2.79563, 2.79695, 
  2.79829, 2.79966, 2.80105, 2.80248, 2.80394, 2.80543, 2.80696, 
  2.80852, 2.81012, 2.81176, 2.81345, 2.81518, 2.81696, 2.81879, 
  2.82067, 2.82261, 2.82462, 2.82669, 2.82883, 2.83105, 2.83335, 
  2.83573, 2.83822, 2.84081, 2.84351, 2.84634, 2.84931, 2.85243, 
  2.85572, 2.8592, 2.8629, 2.86683, 2.87105, 2.87559, 2.88051, 
  2.88587, 2.89177, 2.89834, 2.90575, 2.91426, 2.92427, 2.9365, 
  2.95232, 2.9754, 3.08189, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, Null, 
  Null, Null, Null, Null, Null, Null, Null, Null, Null}}

with

ListLinePlot[data, DataRange -> {0, 2}, 
 PlotRange -> {{0, 3}, {0, Pi}}, Frame -> True]
Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Not an answer

Your plot

ListLinePlot[data, DataRange -> {0, 2},PlotRange -> {{0, 3}, {0, Pi}}, Frame -> True]

looks fine in MMA v11.0.1

enter image description here

What are the smoothness & gap issues you're asking for?

Perhaps an answer

If you try to remove Null (thereby I'm assuming that the points Transpose[data] belong together)

DeleteCases[Transpose[data], {___, Null, ___}] // Transpose;
ListLinePlot[%, DataRange -> {0, 2}, PlotRange -> {{0, 3}, {0, Pi}},Frame -> True]

enter image description here

Improve this answer
$\endgroup$
5
  • $\begingroup$ Yes, the sharp points, around the vertical blue branch, where it meets the orange and orange meets the green, should be actually smooth curves, however, even if I make the x-axis grid very fine, upto 0.0001, I still get the same pattern.. $\endgroup$
    – AtoZ
    Commented Aug 6, 2019 at 8:47
  • $\begingroup$ Then it means that I won't need to use padding for the lists? $\endgroup$
    – AtoZ
    Commented Aug 6, 2019 at 8:58
  • 1
    $\begingroup$ What means"padding for the lists" ? Only the last "point" in DeleteCases[Transpose[data], {___, Null, ___}] // Transpose; makes the curves unsmooth. $\endgroup$
    – Ulrich Neumann
    Commented Aug 6, 2019 at 9:03
  • $\begingroup$ I suspect that even though I don't use Joined-->True but I think that the problem is due the data points are joined automatically? $\endgroup$
    – AtoZ
    Commented Aug 6, 2019 at 11:57
  • 1
    $\begingroup$ That's because you use ListLinePlot, only in ListPlot the option Joined->True is necessary. Remove the last point DeleteCases[Transpose[data], {___, Null, ___}] //Most // Transpose; and you'll get three smooth curves! $\endgroup$
    – Ulrich Neumann
    Commented Aug 6, 2019 at 12:15

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.