Take the following symbolic sum:
Sum[(-1)^n/Factorial[2*n]*(Pi/2)^(2*n)*n, {n, 1, Infinity}]
Mathematica answers me: $- \frac{\pi}{4}$
It is great, I have an exact expression for this complicated sum. But I would like to know how mathematica knows this result.
Is there a way to ask him which function he used to compute it ?
You can use a Wolfram|Alpha query and then look at the Series Representation results.
In Mathematica, the easiest way to do this is start your input with == and it will change it to a spikey input symbol.
==Sum[(-1)^n/Factorial[2*n]*(Pi/2)^(2*n)*n, {n, 1, Infinity}]
Result:
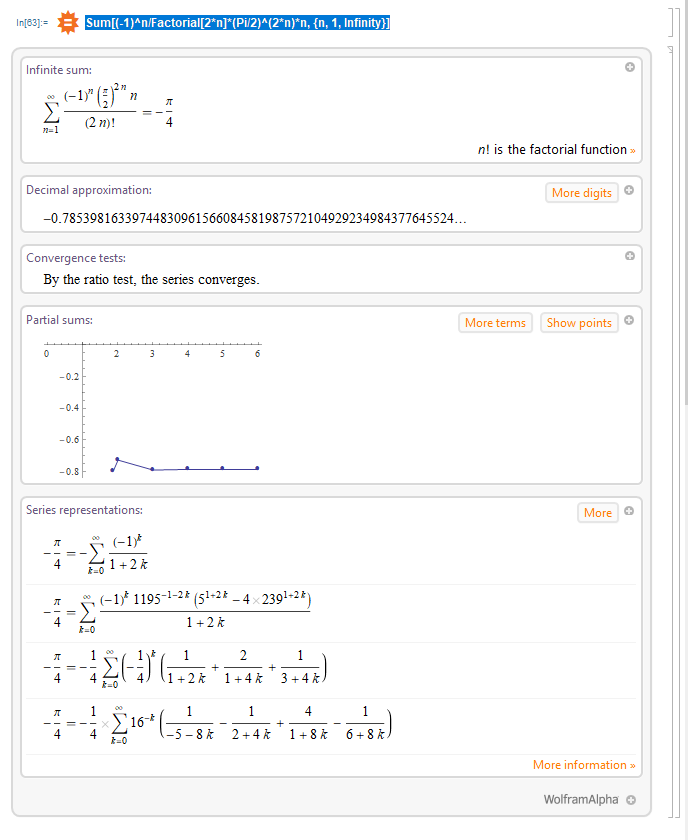
In general, symbolic sums are evaluated by using a combination of internal methods and it is usually difficult to give insight into the evaluation process.
However, this sum is evaluated by using a representation in terms of HypergeometricPFQ (strictly speaking, this is a Hypergeometric0F1).
This may be seen by using the (undocumented) Method option setting "InactivePFQ" as shown below.
In[1]:= Sum[(-1)^n/Factorial[2*n]*(Pi/2)^(2*n)*n, {n, 1, Infinity},
Method -> "InactivePFQ"] // InputForm
Out[1]//InputForm=
-(Pi^2*Inactive[HypergeometricPFQ][{}, {3/2}, -Pi^2/16])/8
In[2]:= Activate[%] // FullSimplify // InputForm
Out[2]//InputForm=
-Pi/4
In[3]:= N[%]
Out[3]= -0.785398
In[4]:= NSum[(-1)^n/Factorial[2*n]*(Pi/2)^(2*n)*n, {n, 1, Infinity}]
Out[4]= -0.785398
The representation in terms of HypergeometricPFQ can be understood by defining a sequence corresponding to the first argument of Sum as follows (the sequence has been shifted so that it starts at 0, rather than 1).
a[n_] = (-1)^n/Factorial[2*n]*(Pi/2)^(2*n)*n /. {n -> n + 1};
The factor of-Pi^2/8 in the answer from Sum is the zeroth term of this sequence.
In[6]:= a[0] // InputForm
Out[6]//InputForm=
-Pi^2/8
The arguments of HypergeometricPFQ in this case can be understood by computing the ratio of the adjacent terms of a[n].
In[7]:= DiscreteRatio[a[n], n] // InputForm
Out[7]//InputForm=
-Pi^2/(8*(1 + n)*(3 + 2*n))
In[8]:=
Simplify[% == -(Pi^2/16)*(1/((n + 1)*(n + 3/2)))]
Out[8]= True
The factor of (n+1) in the denominator of the above comes from the definition of Hypergoemetric0F1 and can be ignored.
Hope this helps in understanding the result from Sum.
