Sol is the evaluation at $t=100$ of the solution of a nonlinear system of three differential equations with initial values at $a$, $b$, $c$. This is obtained with the function ParametricNDSolveValue.
sol = ParametricNDSolveValue[
{x'[t] == -y[t],
y'[t] == -z[t],
z'[t] == -0.915 x[t]+(1-0.915 x[t]^2) y[t]-z[t],
x[0] == a, y[0] == b, z[0] == c},
{x[100], y[100], z[100]}, {t, 0, 100}, {a, b, c}
]
The point $\{xeq,yeq,zeq\}=\{0,0,0\}$ is one of the stable equilibriums of the alleged system. I would like to obtain the stable region of the this equilibrium so for that I'm trying the following: calculate the distance from a point evaluated with Sol to the equilibrium and then plot all these points. The points I evaluate is the points of the unit cube obtained with a step of 0.1 in each of the coordinates:
Table[EuclideanDistance[sol[a, b, c], {xeq, yeq, zeq}], {a,0, 1,0.1}, {b, 0, 1, 0.1},{c,0,1,0.1}]
This provides me with the distance. The problems are two:
1) I don't know how to obtain back the point $\{a,b,c\}$ (the argument of Sol) for the distances that are less than, say, 0.1 from the equilibrium.
2) I actually have only tried with only one or two variables in a small range, say,
Table[EuclideanDistance[sol[a, b, 0.1], {xeq, yeq, zeq}], {a,0.4, 0.6,0.1}, {b, 0.7, 0.9, 0.1}]
because I usually get the following
General: 1/4.60387*10^307 is too small to represent as a normalized machine number; precision may be lost.
which makes the evaluation last forever not showing any outcome.
How can I overcome these issues?

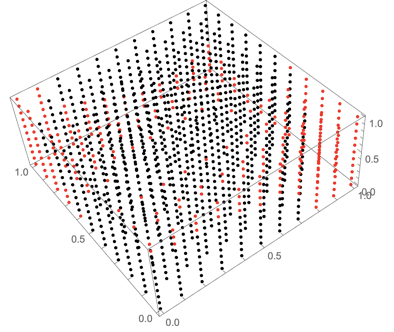
solin your question. $\endgroup${xeq, yeq, zeq}? $\endgroup$