Automatically Trimming Noisy Experimental Data and Extrapolating It

Question

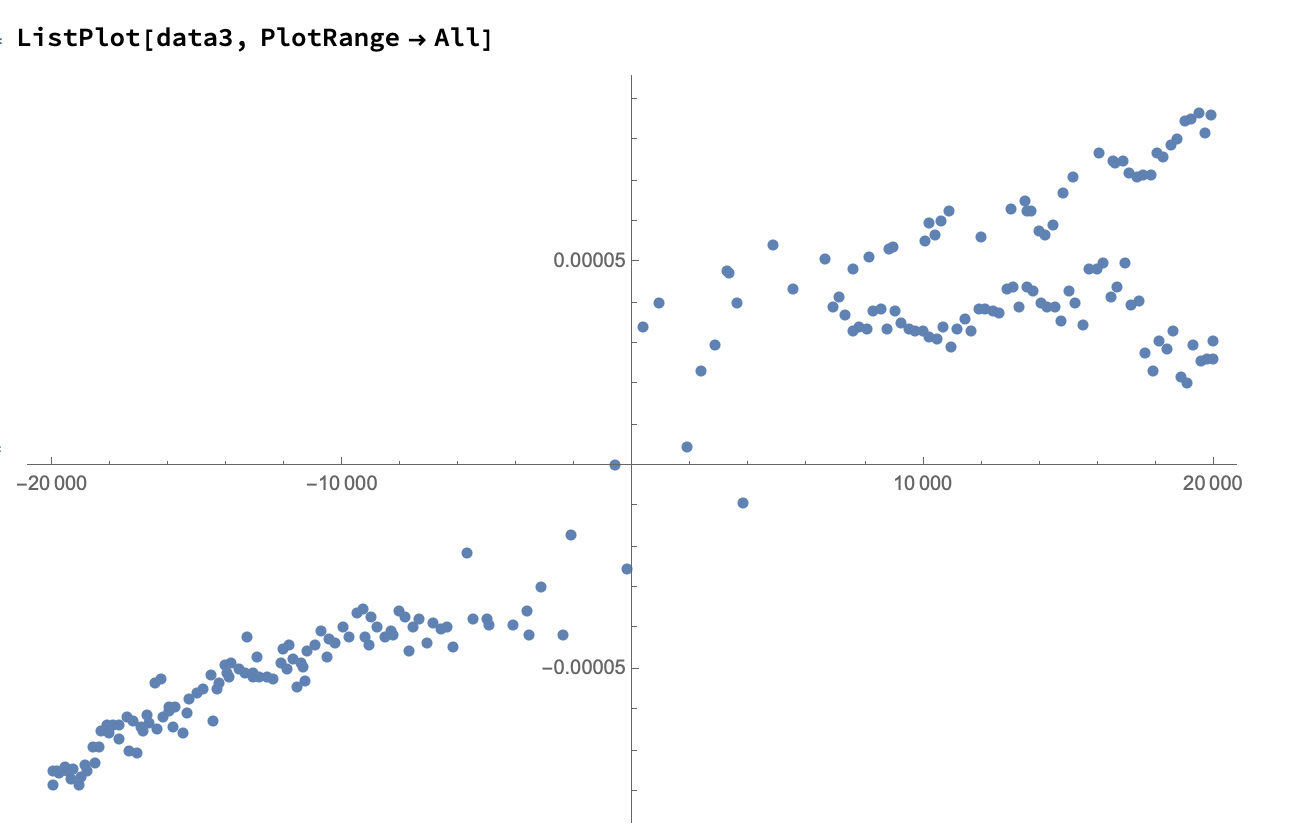
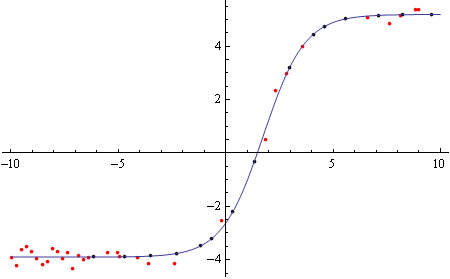
I have experimental data which looks something like this

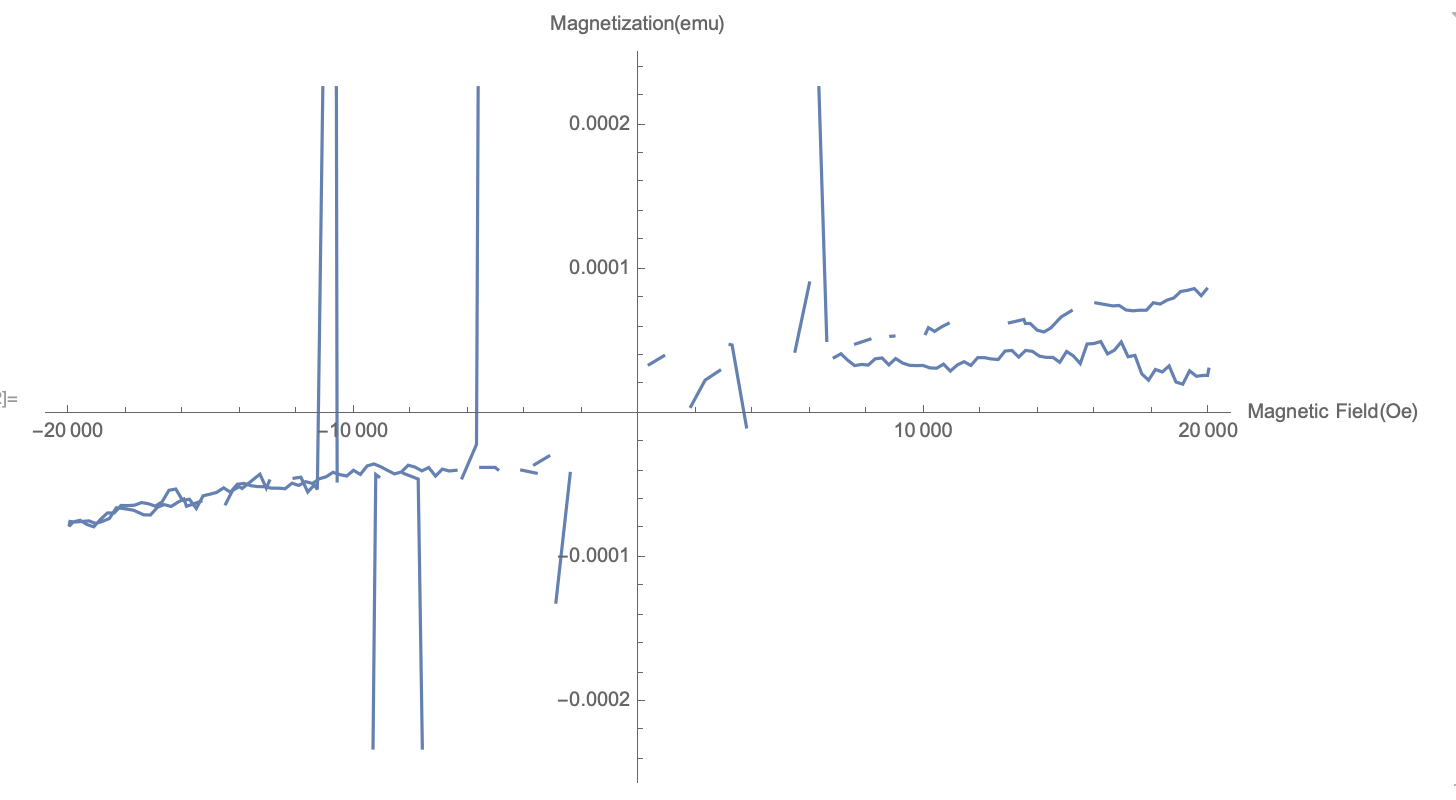
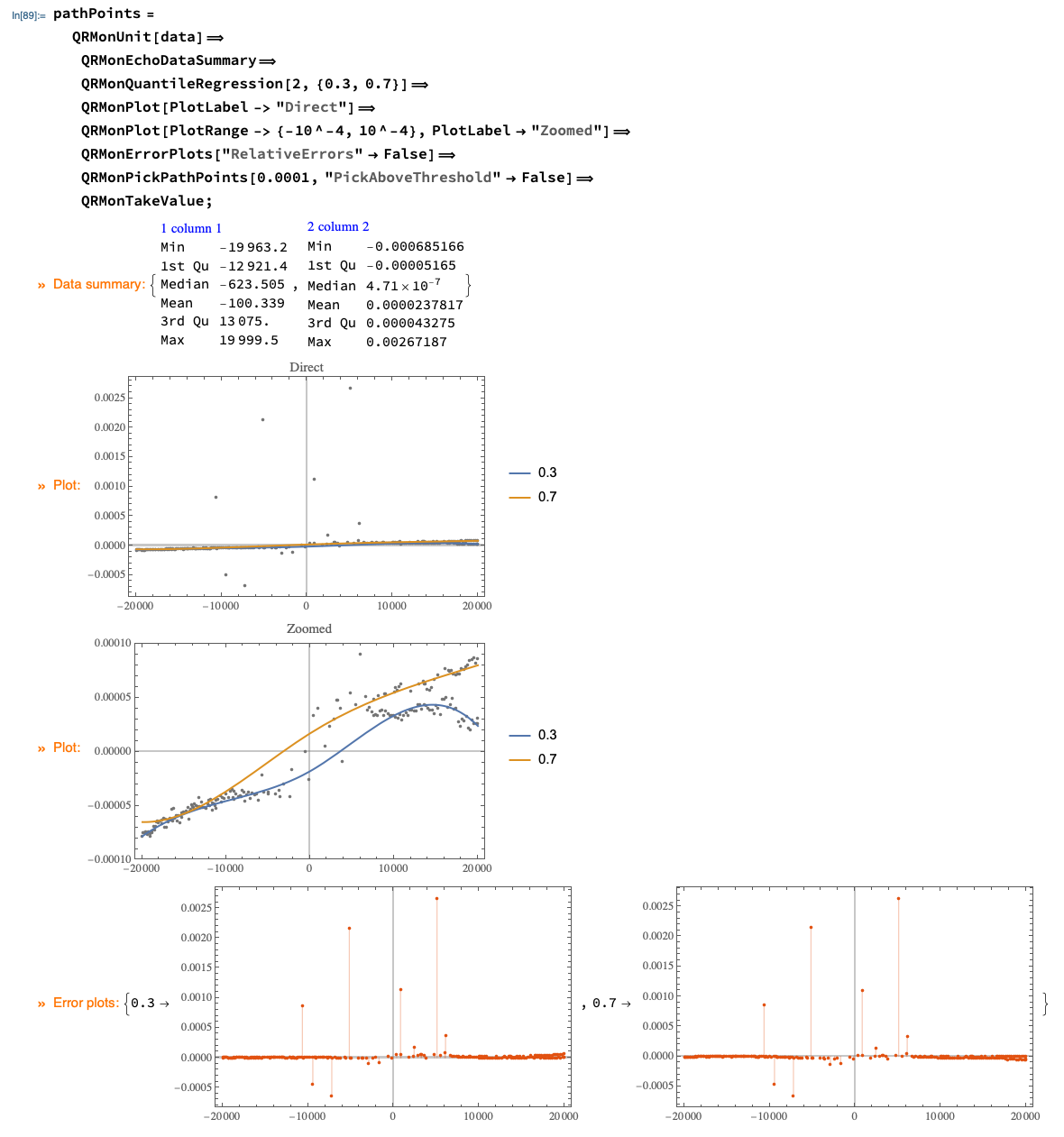
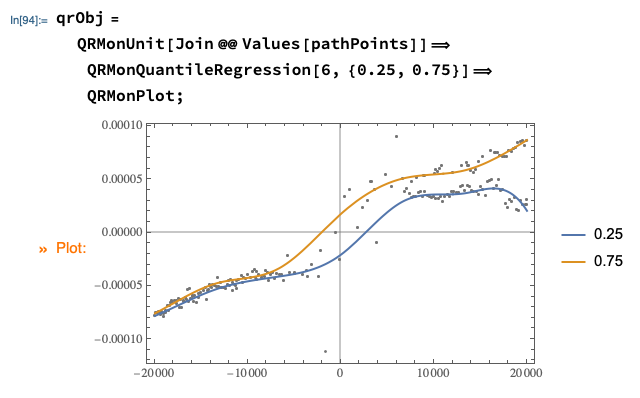
I first want to remove the outlier points which are at quite high value such as around x= -10,000 etc, how to automatically trim these points.

Then around x=0, I have missing data points and I want to extrapolate the existing curve to get a new version. How should I do it?

If anyone is interested here is my data:

Magneticfield = {19999.5, 19958.5, 19804.9, 19567., 19327.5, 19088.7, 18849.6, \
18609.8, 18370.3, 18129.3, 17888.9, 17650., 17410.4, 17170.6, \
16930.9, 16691.3, 16451.7, 16211.9, 15973., 15734., 15494., 15254.1, \
15014.6, 14774.4, 14534.1, 14294.4, 14055.5, 13815.7, 13575.9, \
13336.9, 13096.7, 12856.8, 12617.1, 12377.2, 12137.3, 11897.6, \
11658., 11418.5, 11179., 10939.4, 10699.4, 10459.7, 10220.1, 9980.23, \
9741.27, 9502.3, 9261.83, 9021.18, 8782.03, 8543.24, 8303.09, \
8062.75, 7822.94, 7582.47, 7342.6, 7104.21, 6865.2, 6626.64, 5999.22, \
5493.19, 5398.44, 4798.75, 4292.4, 3789.79, 3284.89, 3215.92, \
3027.13, 2410.08, 1895.67, 1391.51, 885.82, 379.671, -115.872, \
-623.505, -1125.88, -1634.51, -2137.94, -2639.87, -3141.62, -3642.53, \
-4153.16, -4658.18, -5166.93, -5676.18, -6181.8, -6192.78, -6709.73, \
-7219.03, -7722.2, -8228.04, -8412.4, -9101.69, -9208.82, -9436.59, \
-10058.5, -10562.5, -10657.5, -11257.4, -11365.9, -11593.3, -11832.9, \
-12072.6, -12309.9, -12939.8, -13046.3, -13271.3, -13893.5, -14002.1, \
-14230.1, -14469.8, -14709.9, -15339.3, -15844., -15937., -16148.2, \
-16387.8, -16627.7, -16867.4, -17106.3, -17345.6, -17705.2, -18065.2, \
-18306.4, -18546.3, -18785.1, -19025., -19264.9, -19504.7, -19744.8, \
-19932.6, -19963.2, -19813.5, -19576.4, -19336.8, -19097.2, -18857.5, \
-18617.9, -18379., -18138.8, -17898.7, -17659.8, -17419.9, -17180.1, \
-16940.3, -16700.5, -16461., -16221.3, -15982.3, -15742.4, -15501.9, \
-15263., -15024.1, -14783.9, -14543.7, -14304., -14064.1, -13824.3, \
-13585.3, -13346.6, -13106.6, -12866.2, -12626.5, -12386.7, -12146.9, \
-11907.1, -11667.1, -11426.8, -11186.9, -10947.4, -10708.3, -10469.1, \
-10228.7, -9989.11, -9750.04, -9510.35, -9270.66, -9030.64, -8790.87, \
-8552.03, -8312.43, -8072.06, -7831.46, -7591.77, -7352.59, -7112.63, \
-6873.59, -6634.13, -6393.24, -6154.2, -5527.38, -5026., -4929.63, \
-4713.55, -4091.62, -3585.43, -3493.45, -2891.49, -2390.03, -2292.88, \
-1693.41, -1188.46, -682.805, -179.91, 319.04, 823.518, 1328.78, \
1839.95, 2339.51, 2849.49, 2963.87, 3578.31, 4082.59, 4586.96, \
5092.08, 5597.75, 6089.97, 6605.45, 7109.6, 7616.6, 8118.46, 8228.8, \
8851.97, 8960.51, 9565.92, 10072.3, 10170.3, 10385.8, 10624.7, \
10864.6, 11491.5, 11997.1, 12505.6, 13009.7, 13515.9, 13569., \
13740.6, 13980.5, 14220.3, 14460.9, 14821., 15180.2, 15420.2, \
16040.6, 16547.8, 16642.7, 16857., 17096.8, 17336.6, 17575.8, \
17814.8, 18055.7, 18296., 18535.2, 18774.9, 19014.7, 19254.5, \
19494.2, 19734.2, 19926.9};

Magnetization = {0.0000308, 0.0000265, 0.0000265, 0.0000259, 0.0000297, 0.0000204, \
0.0000219, 0.0000331, 0.0000289, 0.0000306, 0.0000232, 0.0000276, \
0.0000404, 0.0000394, 0.0000497, 0.000044, 0.0000414, 0.00005, \
0.0000486, 0.0000483, 0.0000347, 0.00004, 0.0000431, 0.0000356, \
0.000039, 0.000039, 0.0000399, 0.0000432, 0.0000438, 0.0000392, \
0.0000438, 0.0000434, 0.0000375, 0.0000379, 0.0000388, 0.0000388, \
0.0000334, 0.000036, 0.0000337, 0.0000295, 0.0000343, 0.0000315, \
0.0000317, 0.0000334, 0.0000333, 0.0000335, 0.0000351, 0.0000382, \
0.0000338, 0.0000386, 0.000038, 0.0000337, 0.0000341, 0.0000333, \
0.000037, 0.0000415, 0.0000389, "", 0.0000906, 0.0000433, "", \
0.0000543, "", -9.22*10^-6, 0.0000476, 0.0000479, "", 0.0001704, "", \
"", 0.00004, 0.000034, "", 
 4.71*10^-7, "", "", -0.0000169, "", -0.0000295, -0.0000354, "", "", \
0.00213533, -0.0000214, -0.0000446, "", "", -0.000685166, -0.0000454, \
-0.0000415, "", -0.0000438, -0.0000422, -0.000492149, "", -0.0000467, \
0.00081401, -0.0000526, -0.0000495, -0.0000544, -0.0000441, \
-0.0000448, "", -0.0000467, -0.0000517, -0.000042, -0.0000519, \
-0.0000507, -0.0000533, -0.0000626, "", -0.0000608, -0.0000643, \
-0.0000592, -0.0000615, -0.0000644, -0.0000631, -0.000065, \
-0.0000703, -0.0000702, -0.000067, -0.0000658, -0.0000653, \
-0.0000727, -0.0000747, -0.0000762, -0.0000744, -0.0000748, \
-0.0000752, -0.0000748, -0.0000782, -0.0000751, -0.000074, \
-0.0000769, -0.0000785, -0.0000733, -0.0000689, -0.0000689, \
-0.0000637, -0.0000638, -0.0000635, -0.0000617, -0.0000625, \
-0.0000642, -0.0000612, -0.0000532, -0.0000523, -0.0000602, \
-0.0000594, -0.0000658, -0.000057, -0.0000559, -0.0000546, \
-0.0000515, -0.0000545, -0.000049, -0.0000484, -0.00005, -0.0000506, \
-0.0000507, -0.0000517, -0.0000517, -0.0000521, -0.0000483, \
-0.0000498, -0.0000472, -0.0000485, -0.0000452, -0.0000438, \
-0.0000407, -0.0000423, -0.0000433, -0.0000393, -0.0000422, \
-0.0000363, -0.0000349, -0.0000369, -0.0000394, -0.0000418, \
-0.0000405, -0.0000358, -0.0000371, -0.0000397, -0.0000374, \
-0.0000433, -0.0000385, -0.0000398, -0.0000393, "", -0.0000373, \
-0.0000373, -0.0000388, "", -0.0000392, -0.0000413, "", -0.000130764, \
-0.0000415, "", -0.000111659, "", "", -0.0000255, "", 0.00111743, "", 
 4.96*10^-6, 0.0000232, 0.0000299, "", 0.00004, "", "", 0.00267187, \
"", 0.000377004, 0.0000508, "", 0.0000483, 0.0000516, "", 0.0000536, \
0.0000538, "", 0.0000555, 0.0000596, 0.000057, 0.0000601, 0.0000625, \
"", 0.0000562, "", 0.000063, 0.0000653, 0.0000625, 0.0000625, \
0.0000579, 0.0000566, 0.0000594, 0.000067, 0.0000713, "", 0.0000768, \
0.000075, 0.0000747, 0.000075, 0.0000719, 0.0000713, 0.0000717, \
0.0000717, 0.0000769, 0.000076, 0.0000787, 0.0000802, 0.0000847, \
0.0000855, 0.0000867, 0.0000818, 0.0000864}

Final plot should be hysteresis like this:

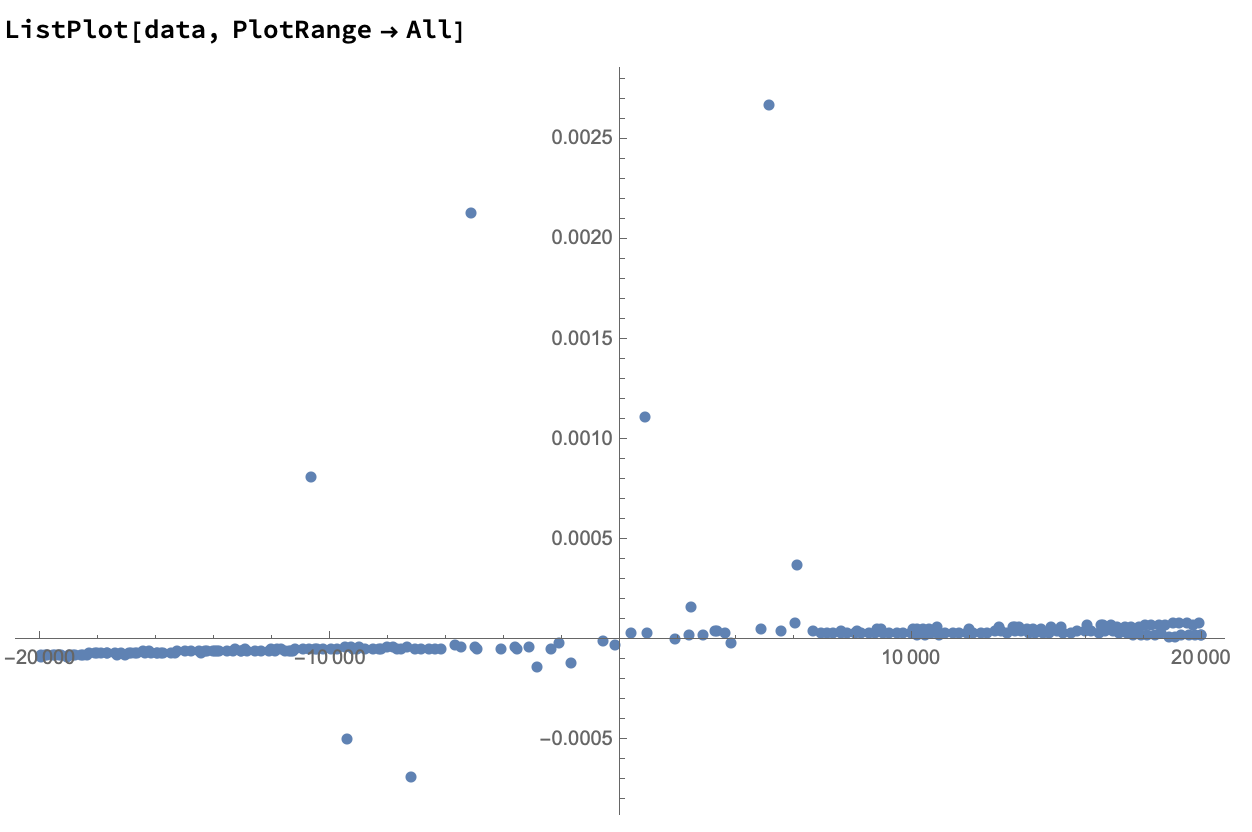
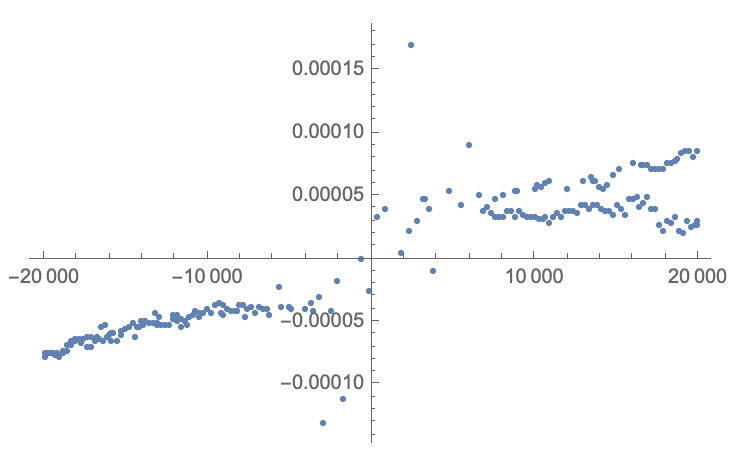
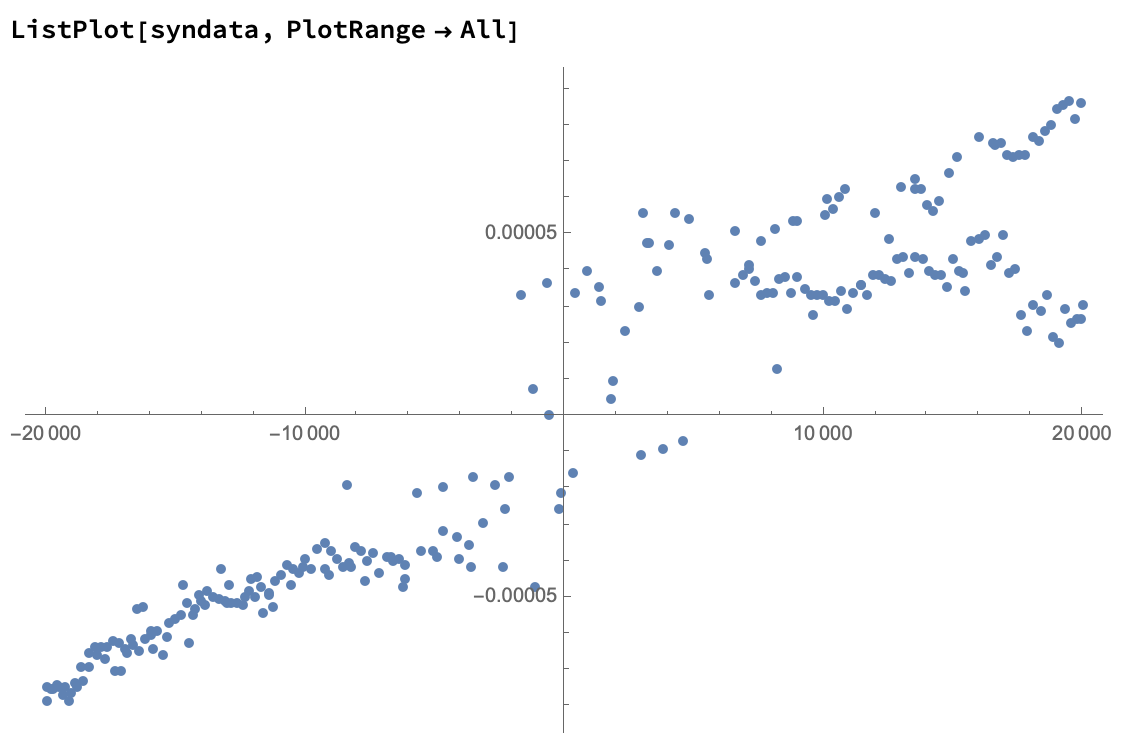
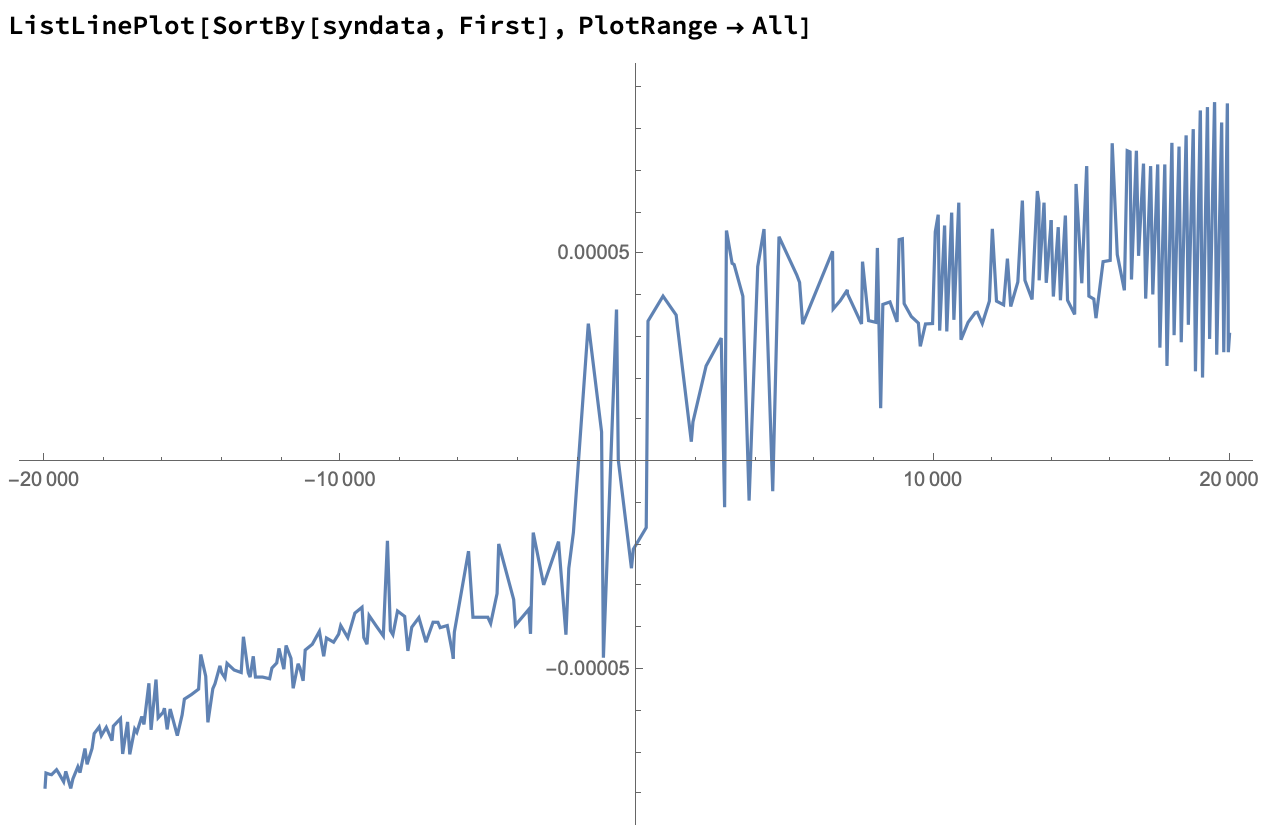
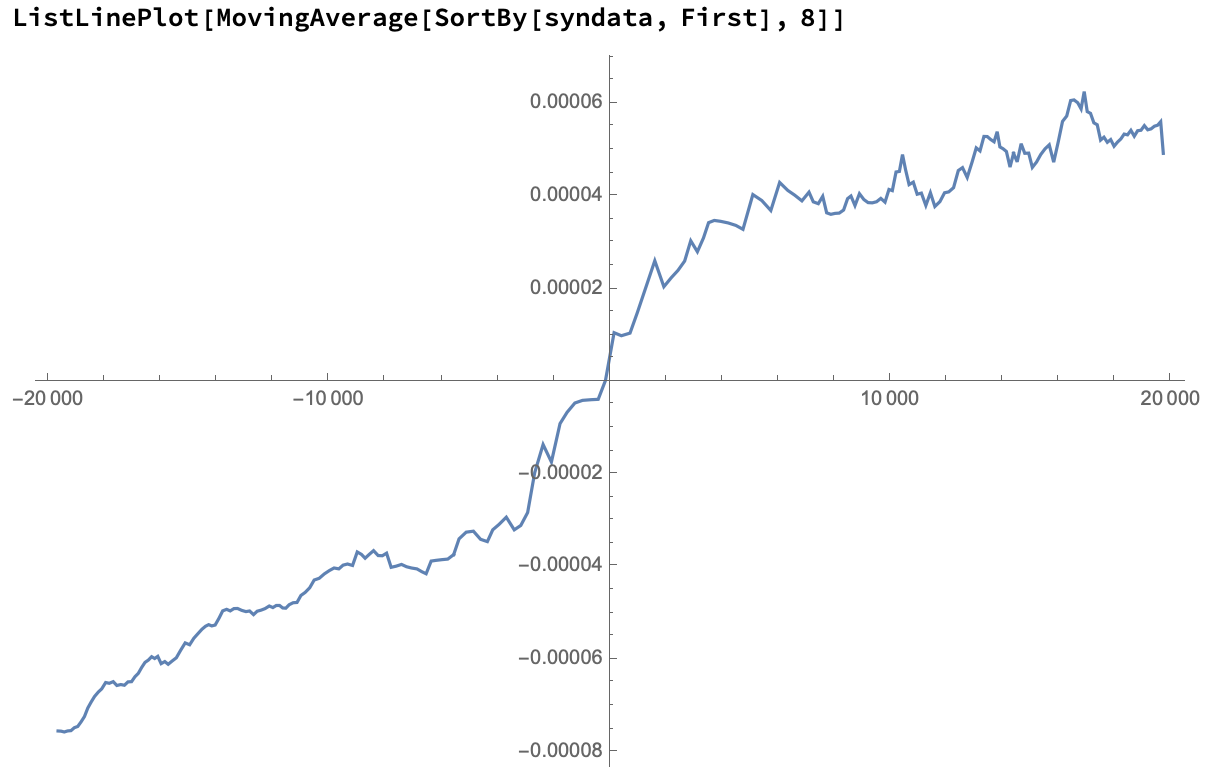
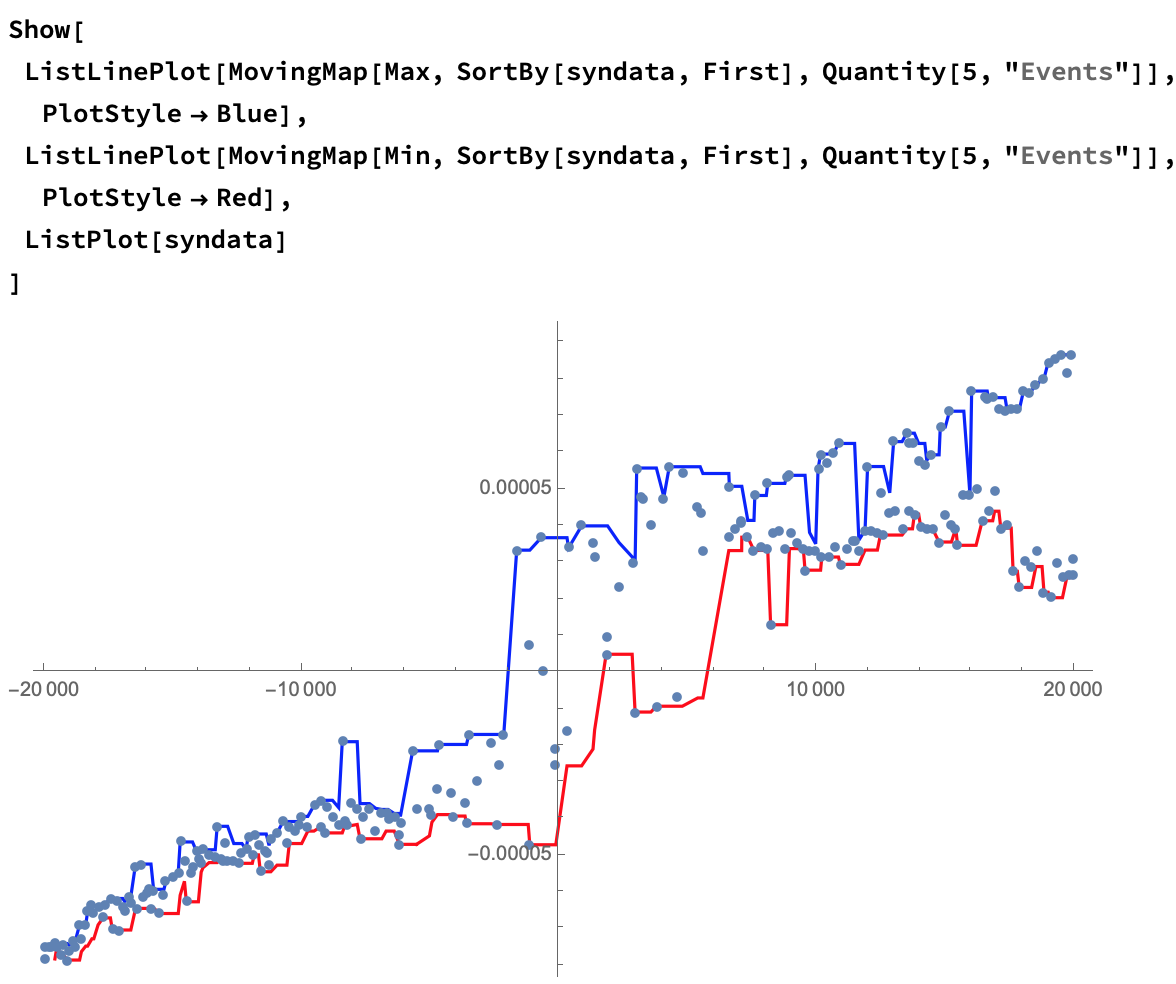
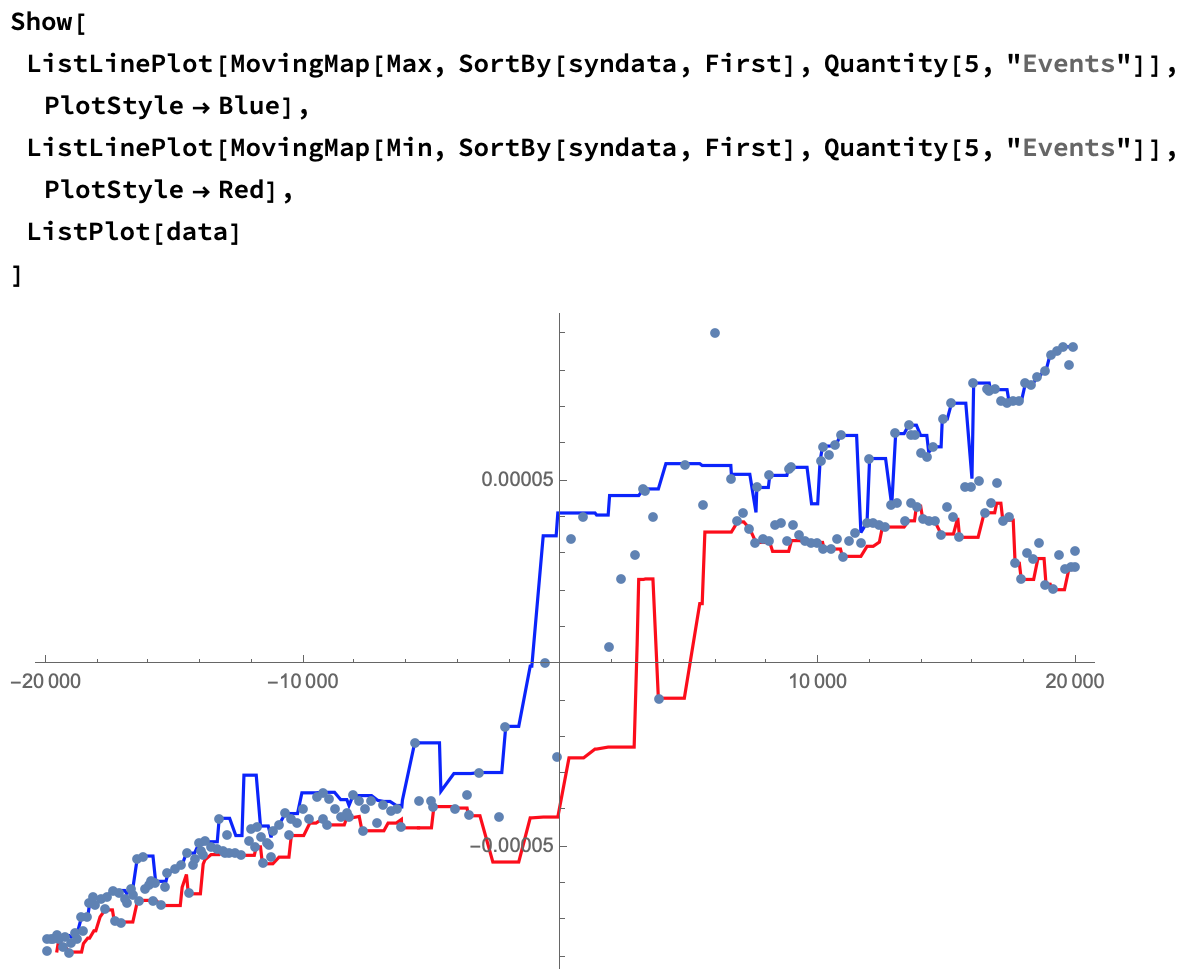
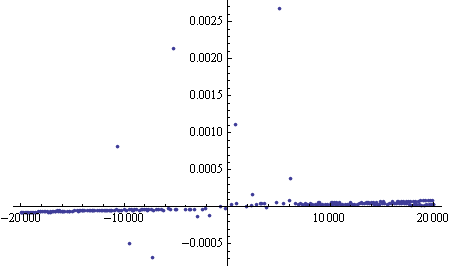
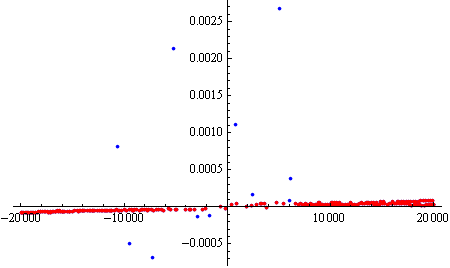
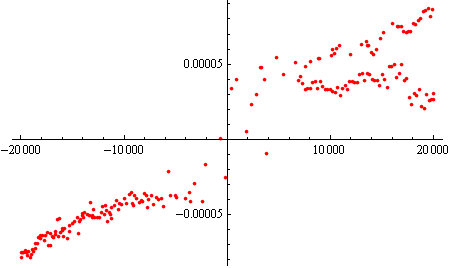
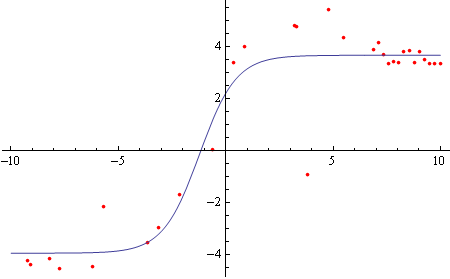
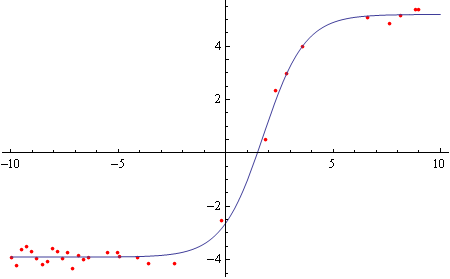
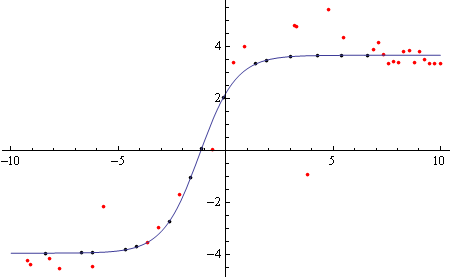
You can format inline code and code blocks by selecting the code and clicking the {} button above the edit window. The edit window help button ? is useful for learning how to format your questions and answers. You may also find this meta Q&A helpful — Michael E2, Commented May 21, 2019 at 2:54
Why does Magnetization have a bunch of values that are ""? Quotation marks indicate strings, and strings cannot be plotted. — MassDefect, Commented May 21, 2019 at 5:12
How were the hysteresis loops measured? I would hesitate to remove outliers to fit a new curve in without knowing more about the methods used and the errors involved, as the outliers may very well be 'real' information, and the hysteresis loops may be real. Hysteresis loops, whilst can be naively described as a Sigmoid function, can be more complicated than that: physics.stackexchange.com/questions/60194/… or ieeexplore.ieee.org/document/7133863 — Letshin, Commented Jun 7, 2019 at 11:09

Carl Lange · Accepted Answer · 2019-05-21 07:39:46Z

We can do the first part rather easily using FindAnomalies or DeleteAnomalies, and the second can be attempted using SynthesizeMissingValues. All these functions are new in version 12.

First, let's remind ourselves of your initial data.

We do a first run of DeleteAnomalies using a high AcceptanceThreshold, in order to remove the really blatant outliers. I also replace "" with Missing[], as it seems that's what's intended, and "" will cause the automatic anomaly detection to work less well, since the data is not all numeric.

data = data /. "" -> Missing[]

data2 = DeleteAnomalies[data, AcceptanceThreshold -> .2]

Now we are left with "smaller" anomalies:

ListPlot[data2, PlotRange->All]

We can now run DeleteAnomalies again, with a lower AcceptanceThreshold (in this case the default of 0.001), to get the more "local" anomalies:

data3 = DeleteAnomalies[data2];

And now we are left with what looks like very clean data:

Now, let's see how SynthesizeMissingValues will do on this data, to find your missing values. (Remember that we replaced "" with Missing[] above - this is required)

syndata = SynthesizeMissingValues[data3, PerformanceGoal -> "Quality", TimeGoal -> Quantity[60, "Seconds"]]

And now let's have a look at the outcome:

ListPlot[syndata, PlotRange->All]

Let's sort the points and plot them on a line:

We can approximate results by taking a moving average of these points:

Since you're looking for a hysteresis, we can approximate that using a moving Min and Max:

Show[
 ListLinePlot[
  MovingMap[Max, SortBy[syndata, First], Quantity[5, "Events"]], 
  PlotStyle -> Blue],
 ListLinePlot[
  MovingMap[Min, SortBy[syndata, First], Quantity[5, "Events"]], 
  PlotStyle -> Red],
 ListPlot[syndata]
 ]

And to show only your initial data:

Some things to consider:

FindAnomalies, DeleteAnomalies, and SynthesizeMissingValues all use approximation methods, and may give different results every time you run them.
Consider varying the windows for MovingMap.

Would it be better to use SynthesizeMissingValues separately on the two halves of the data, to maintain the hysteresis? In the original unsorted data, it appears that the first half is the "top" of the loop and the second half is the "bottom". — MelaGo, Commented May 21, 2019 at 22:08
That sounds like a great idea @MelaGo! I would encourage you to try it out. There's certainly a lot of room for experimentation here. — Carl Lange, Commented May 21, 2019 at 22:11
My version of MMA doesn't support SynthesizeMissingValues, but I updated my answer with an attempt using curve fitting. — MelaGo, Commented May 21, 2019 at 23:48
Thanks! Not sure it's a good idea to construct data that way though... — MelaGo, Commented May 21, 2019 at 23:57

MelaGo · Accepted Answer · 2019-05-21 23:54:08Z

First, removing outliers:

Make {x,y} pairs and remove points with missing y-values:

data = Transpose[{Magneticfield, Magnetization}];
data2 = DeleteCases[data, {_, ""}];
ListPlot[data2, PlotRange -> All]

In this case, the outliers are so far off that most of them can be removed with simple min-max thresholds.

dataculled = Select[data2, #[[2]] > -0.0001 && #[[2]] < 0.00009 &];
ListPlot[{data2, dataculled}, PlotStyle -> {Blue, Red}, PlotRange -> All]

And now if you zoom in a bit, it does actually resemble a hysteresis loop.

ListPlot[dataculled, PlotStyle -> Red, PlotRange -> All]

Updated to add something about guessing missing values, with the caveat that adding data points this way may not be appropriate or ethical, depending on your experiment.

Unfortunately my old version of MMA doesn't support SynthesizeMissingValues. Instead, I attempted manual fitting using a sigmoidal curve:

model = bottom + (top - bottom)/(1 + 10^((h50 - x) hs));

First looking at the "top" part of the hysteresis loop, and restricting data to domain [-10000,10000]:

tophalf = Select[dataculled[[;; Round[Length[dataculled]/2]]], 10000 > #[[1]] > -10000 &];
topfit = FindFit[{.001, 100000} # & /@ tophalf, model, {bottom, top, h50, hs}, x]
Show[
  ListPlot[{.001, 100000} # & /@ tophalf, PlotStyle -> Red, PlotRange -> All],    
  Plot[model /. topfit, {x, -10, 10}]]
(* For a reason I don't exactly understand, I was unable to fit using the raw data, so I transformed {x,y} by {0.001,100000}. *)

Same for the "bottom" part of the loop:

bottomhalf = Select[dataculled[[Round[Length[dataculled]/2] ;;]], 10000 > #[[1]] > -10000 &];
bottomfit = FindFit[{.001, 100000} # & /@ bottomhalf, model, {bottom, top, h50, hs}, x]
Show[
  ListPlot[{.001, 100000} # & /@ bottomhalf, PlotStyle -> Red, PlotRange -> All], 
  Plot[model /. bottomfit, {x, -10, 10}]]

Now to calculate missing values based on the curve fits.

Finding x-values with missing y-values:

topmissing = Select[Cases[data[[;; Round[Length[data]/2]]], {_, ""}], 10000 > #[[1]] > -10000 &][[All, 1]];

Plugging those x-values into the model:

topsynth = Table[{m, (model /. topfit /. x -> 0.001 m)/100000}, {m,topmissing}];
Show[ListPlot[{{.001, 100000} # & /@ tophalf, {.001, 100000} # & /@ topsynth}, PlotStyle -> {Red, Black}, PlotRange -> All], 
 Plot[model /. topfit, {x, -10, 10}]]

And same for the bottom part:

bottommissing = Select[Cases[data[[Round[Length[data]/2] ;;]], {_, ""}], 10000 > #[[1]] > -10000 &][[All, 1]];
bottomsynth = Table[{m, (model /. bottomfit /. x -> 0.001 m)/100000}, {m, bottommissing}];
Show[ListPlot[{{.001, 100000} # & /@ bottomhalf, {.001, 100000} # & /@
     bottomsynth}, PlotStyle -> {Red, Black}, PlotRange -> All], 
 Plot[model /. bottomfit, {x, -10, 10}]]

Finally merging the old and new values:

fulltop = Join[dataculled[[;; Round[Length[dataculled]/2]]], topsynth];
fullbottom = Join[dataculled[[Round[Length[dataculled]/2] ;;]], bottomsynth];
Show[
 ListPlot[{fulltop, fullbottom}, PlotRange -> {-0.0001, 0.0001}],
 Plot[{(model /. topfit /. x -> 0.001 xx)/100000, (model /. bottomfit /. x -> 0.001 xx)/100000}, {xx, -10000, 10000}]]

Anton Antonov · Accepted Answer · 2019-05-23 20:24:27Z

I like the approach taken in Carl Lange's answer -- built-in functions are used.

That said, in previous answers, like this one, there is a clear streamlined workflow using the software monad QRMon.

Summarize the data.
Do a Quantile Regression (QR) fit.
- Using appropriate QR parameters and two probability values.
Pick points close to the fitted curves.
- Using an appropriate threshold.
- The absolute error plots can help with selecting the threshold.
Plot the picked points.
If the results are not satisfactory go to 2.
The outliers are removed at this point -- they were not picked.
Do a QR fit over the picked points and plot the fitted functions.
- Using appropriate QR parameters and two probability values.
If the results are not satisfactory goto 7.
Get the regression functions and apply them over points of interest.

Code

(For the computation results see the output section below.)

data = Transpose[{Magneticfield, Magnetization}];
data = Select[data, VectorQ[#, NumericQ] &];


Import["https://raw.githubusercontent.com/antononcube/\
MathematicaForPrediction/master/MonadicProgramming/\
MonadicQuantileRegression.m"]

(* Steps 1-5 *)
pathPoints =
  QRMonUnit[data]⟹
   QRMonEchoDataSummary⟹
   QRMonQuantileRegression[2, {0.3, 0.7}]⟹
   QRMonPlot[PlotLabel -> "Direct"]⟹
   QRMonPlot[PlotRange -> {-10^-4, 10^-4}, PlotLabel -> "Zoomed"]⟹
   QRMonErrorPlots["RelativeErrors" -> False]⟹
   QRMonPickPathPoints[0.0001, "PickAboveThreshold" -> False]⟹
   QRMonTakeValue;

(* Steps 7 and 8 *)
qrObj =
  QRMonUnit[Join @@ Values[pathPoints]]⟹
   QRMonQuantileRegression[6, {0.25, 0.75}]⟹
   QRMonPlot;

(* Step 9 *)
qFuncs = qrObj⟹QRMonTakeRegressionFunctions;

Map[#[0] &, qFuncs]

(* <|0.25 -> -0.0000208432, 0.75 -> 0.0000174134|> *)

Stack Exchange Network

Automatically Trimming Noisy Experimental Data and Extrapolating It

3 Answers 3

Code

Output

Steps 1-5

Steps 7 and 8

Your Answer

Not the answer you're looking for? Browse other questions tagged
plotting
data
or ask your own question.

Linked

Hot Network Questions

Automatically Trimming Noisy Experimental Data and Extrapolating It

3 Answers 3

Code

Output

Steps 1-5

Steps 7 and 8

Your Answer

Sign up or log in

Post as a guest

Not the answer you're looking for? Browse other questions tagged plottingdata or ask your own question.

Linked

Related

Hot Network Questions

Not the answer you're looking for? Browse other questions tagged
plotting
data
or ask your own question.