2
$\begingroup$

I am trying to simulate the growth of cell populations through a system of coupled PDEs. There are six equations in total but Mathematica takes a long time to solve the system (+6 hours running) and I do not know when it will stop or end.

The code of my system of equations is the following:

H[\[Psi]_, \[Sigma]_] := Which[\[Psi] <= 0, 1, \[Psi] > \[Sigma], 0, True, 1 
- \[Psi]/\[Sigma]]

\[CapitalSigma][Y_, psi0_] := ((Y - psi0)/(1 - Y))*((2 - psi0)/(1 - psi0)) 

gaman = 0.746;  (*reproduccion celulas sanas*)
gamat = 0.97;    (*reproducion celulas tumorales*)
psi0 = 0.75;      (*razon de volumen libre de estres*)
psin = 0.6;        (*valor umbral que detiene el crecimiento c. sanas*)
psit = 0.8;        (*valor umbral que detiene el crecimiento c. \tumolaes*)
deltat = 0.03;  (*rapidez muerte celulas tumorales*)
deltan = 0.1;    (*rapidez muerte celulas sanas*)
mun = 0.1;           (*rapidez reproduccion MEC por celulas sanas*)
mut = 0.05;        (*rapidez reproduccion MEC por celulas tumorales*)
nu = 0.000016; (*coeficiente de degradacion de la MEC por enzimas*)
pin = 6000000;(*produccion de enzimas que degradan la MEC por c. \sanas*)
pit = 3000000;(*produccion de enzimas que degradan la MEC por c.\tumorales*)
tau = 0.005;         (*tiempo de vida medio de las enzimas*)
fo = 0.25;          (*suministro de oxigeno*)
fg = 0.16;          (*suministro de glucosa*)
betan = 1.2;      (*tasa de absorcion de nutrientes celulas \sanas*)
betat = 1.5;      (*tasa de absorcion de nutrientes celulas \tumorales*)

\[Sigma] = 0.2;          
\[CapitalKappa]n = 0.1;
\[CapitalKappa]t = 0.3;
\[Kappa] = 0.00000734;
Diff = 1.0;

eqns = { 
       D[fin[t, x, y], t] == fin[t, x, y]*\[CapitalKappa]n*(D[fin[t, x,y]*\
       [CapitalSigma][
       fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0], x, x] + 

       D[fin[t, x, y]*\[CapitalSigma][fin[t, x, y] + fit[t, x, y] + mat[t, 
       x, y], psi0], y,y]) gaman*fin[t, x, y]*H[(fin[t, x, y] + fit[t, x, y] 
       + mat[t, x, y]) - psin, \[Sigma]] - (1 - oxi[t, x, y])*deltan*fin[t, 
       x, y],

       D[fit[t, x, y], t] == fit[t, x,y]*\[CapitalKappa]t*(D[fit[t, x, y]*\
       [CapitalSigma][fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0], x, 
       x] + D[fit[t, x, y]*\[CapitalSigma][fin[t, x, y] + fit[t, x, y] + 
       mat[t, x, y], psi0], y, y]) + gamat*fit[t, x, y]*H[(fin[t, x, y] + 
       fit[t, x, y] + mat[t, x, y]) - psit, \[Sigma]] - (1 - glu[t, x, 
       y])*deltat*fit[t, x, y],


       D[mat[t, x, y], t] == mun*fin[t, x, y]*\[CapitalSigma][fin[t, x, y] + 
       fit[t, x, y] + mat[t, x, y], psi0] + mut*fit[t, x, y]*\[CapitalSigma]
       [fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0] - nu*enz[t, x, 
       y]*mat[t, x, y],


       D[enz[t, x, y],t] == \[Kappa]*(D[enz[t, x, y], x, x] + D[enz[t, x, 
       y], y, y]) + pin*fin[t, x, y]*\[CapitalSigma][fin[t, x, y] + fit[t, 
       x, y] + mat[t, x, y], psi0] + pit*fit[t, x, y]*\[CapitalSigma][
       fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0] - enz[t, x, y]/tau,


       D[oxi[t, x, y], t] == Diff*(D[oxi[t, x, y], x, x] + D[oxi[t, x, y],y, 
       y]) - betan*fin[t, x, y]*oxi[t, x, y] + fo,


       D[glu[t, x, y], t] == Diff*(D[glu[t, x, y], x, x] + D[glu[t, x, y],y, 
       y]) - betat*fit[t, x, y]*glu[t, x, y] + fg};

IC = {fin[0, x, y] == 0.45, fit[0, x, y] == 0.005, 
mat[0, x, y] == 0.2, enz[0, x, y] == 0.3, oxi[0, x, y] == 0.25, 
glu[0, x, y] == 0.16};
BC = {
Derivative[0, 1, 0][fin][t, 0, y] == 0,
Derivative[0, 1, 0][fin][t, 1, y] == 0,
Derivative[0, 0, 1][fin][t, x, 0] == 0,
Derivative[0, 0, 1][fin][t, x, 1] == 0,

Derivative[0, 1, 0][fit][t, 0, y] == 0,
Derivative[0, 1, 0][fit][t, 1, y] == 0,
Derivative[0, 0, 1][fit][t, x, 0] == 0,
Derivative[0, 0, 1][fit][t, x, 1] == 0,

Derivative[0, 1, 0][mat][t, 0, y] == 0,
Derivative[0, 1, 0][mat][t, 1, y] == 0,
Derivative[0, 0, 1][mat][t, x, 0] == 0,
Derivative[0, 0, 1][mat][t, x, 1] == 0,

Derivative[0, 1, 0][enz][t, 0, y] == 0,
Derivative[0, 1, 0][enz][t, 1, y] == 0,
Derivative[0, 0, 1][enz][t, x, 0] == 0,
Derivative[0, 0, 1][enz][t, x, 1] == 0,

Derivative[0, 1, 0][oxi][t, 0, y] == 0,
Derivative[0, 1, 0][oxi][t, 1, y] == 0,
Derivative[0, 0, 1][oxi][t, x, 0] == 0,
Derivative[0, 0, 1][oxi][t, x, 1] == 0,

Derivative[0, 1, 0][glu][t, 0, y] == 0,
Derivative[0, 1, 0][glu][t, 1, y] == 0,
Derivative[0, 0, 1][glu][t, x, 0] == 0,
Derivative[0, 0, 1][glu][t, x, 1] == 0};

solu = NDSolve[{eqns, IC, BC}, {fin, fit, mat, enz, oxi, glu}, {x, 0, 
1}, {y, 0, 1}, {t, 0, 80}];

I want to see how cell populations grow in 2D but this it take long time to evaluate the system. My boundary conditions are arbitrary so i don't know if they are correct.

I attach an image of my system of equations that I try to solve:

enter image description here

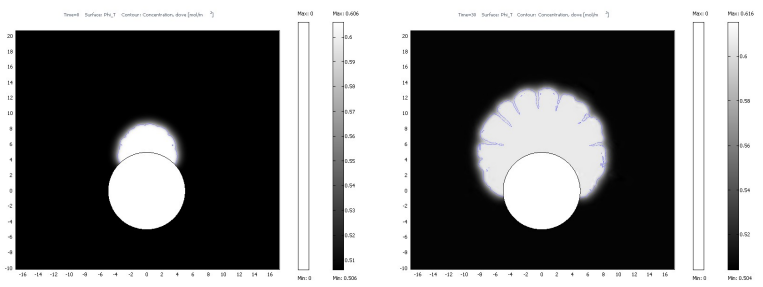
UPDATE I change my BC and my Mathematica finally solve the system quickly but when using DensityPlot to see how behaves in spacetime is not what I really expected.

Really what i hope is see how evolve the volume ratios occupied by two types cells represented by $\phi_n$ and $\phi_t$ (in the image, in the code are "fin" and "fit") into a mesh. In the mesh i want to represent a blood vessel (a circle in a middle of the mesh, for example) and from there to see how the initial group of cells evolve or expand in the confined space of the mesh.

I update my code and I put it below:

H[\[Psi]_, \[Sigma]_] := Which[\[Psi] <= 0, 1, \[Psi] > \[Sigma], 0, True, 1 
- \[Psi]/\[Sigma]]

\[CapitalSigma][Y_, psi0_] := ((Y - psi0)/(1 - Y))*((2 - psi0)/(1 - psi0)) 

gaman = 0.746;  
gamat = 0.97;   
psi0 = 0.75;    
psin = 0.6;     
psit = 0.8;        
deltat = 0.03;  
deltan = 0.1;   
mun = 0.1;      
mut = 0.05;     
nu = 0.000016; 
pin = 6000000;
pit = 3000000;
tau = 0.005;   
fo = 0.25;     
fg = 0.16;     
betan = 1.2;   
betat = 1.5;      
\[Sigma] = 0.2; 
\[CapitalKappa]n = 0.1;
\[CapitalKappa]t = 0.3;
\[Kappa] = 0.00000734;
Diff = 1;
L = 5;
T = 80;



eqns = {
   D[fin[t, x, y], t] == 
    fin[t, x, 
       y]*\[CapitalKappa]n*(D[
         fin[t, x, y]*\[CapitalSigma][
           fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0], x, x] + 
        D[fin[t, x, y]*\[CapitalSigma][
           fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0], y, y]) +
      fin[t, x, y]*gaman*fin[t, x, y]*
      H[(fin[t, x, y] + fit[t, x, y] + mat[t, x, y]) - 
        psin, \[Sigma]] - deltan*fin[t, x, y],


   D[fit[t, x, y], t] == 
    fit[t, x, 
       y]*\[CapitalKappa]t*(D[
         fit[t, x, y]*\[CapitalSigma][
           fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0], x, x] + 
        D[fit[t, x, y]*\[CapitalSigma][
           fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0], y, y]) +
      fit[t, x, y]*gamat*fit[t, x, y]*
      H[(fin[t, x, y] + fit[t, x, y] + mat[t, x, y]) - 
        psit, \[Sigma]] - deltat*fit[t, x, y],


   D[mat[t, x, y], t] == 
    mun*fin[t, x, y]*\[CapitalSigma][
       fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0] + 
     mut*fit[t, x, y]*\[CapitalSigma][
       fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0] - 
     nu*enz[t, x, y]*mat[t, x, y],


   D[enz[t, x, y], 
     t] == \[Kappa]*(D[enz[t, x, y], x, x] + D[enz[t, x, y], y, y]) + 
     pin*fin[t, x, y]*\[CapitalSigma][
       fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0] + 
     pit*fit[t, x, y]*\[CapitalSigma][
       fin[t, x, y] + fit[t, x, y] + mat[t, x, y], psi0] - 
     enz[t, x, y]/tau,


   D[oxi[t, x, y], t] == 
    Diff*(D[oxi[t, x, y], x, x] + D[oxi[t, x, y], y, y]) - 
     betan*fin[t, x, y]*oxi[t, x, y] + fo,


   D[glu[t, x, y], t] == 
    Diff*(D[glu[t, x, y], x, x] + D[glu[t, x, y], y, y]) - 
     betat*fit[t, x, y]*glu[t, x, y] + fg};



IC = {
   fin[0, x, y] == 0.45,
   fit[0, x, y] == 0.05,
   mat[0, x, y] == 0.2,
   enz[0, x, y] == 0.3,
   oxi[0, x, y] == 0.25,
   glu[0, x, y] == 0.16};
   BC = {
   fin[t, -L, y] == fin[t, L, y],
   fin[t, x, -L] == fin[t, x, L],
   fit[t, -L, y] == fit[t, L, y],
   fit[t, x, -L] == fit[t, x, L],
   mat[t, -L, y] == mat[t, L, y],
   mat[t, x, -L] == mat[t, x, L],
   enz[t, -L, y] == enz[t, L, y],
   enz[t, x, -L] == enz[t, x, L],
   oxi[t, -L, y] == oxi[t, L, y],
   oxi[t, x, -L] == oxi[t, x, L],
   glu[t, -L, y] == glu[t, L, y],
   glu[t, x, -L] == glu[t, x, L]
   };

pdes = Flatten@{eqns, IC, BC};

sol1 = NDSolve[
  pdes, {fin, fit, mat, enz, oxi, glu}, {t, 0, T}, {x, -L, L}, {y, -L,
    L}, PrecisionGoal -> 2, 
  Method -> {"MethodOfLines", 
    "SpatialDiscretization" -> {"TensorProductGrid", 
      "MaxPoints" -> 100}}]

Plot[Evaluate[fin[t, 1, 1] /. sol1], {t, 0, 80}, PlotRange -> All]


Plot[Evaluate[mat[t, 1, 1] /. sol1], {t, 0, 80}, PlotRange -> All]


Table[DensityPlot[
  Evaluate[glu[t, x, y] /. sol1], {x, -L, L}, {y, -L, L}, 
  ColorFunction -> "SunsetColors", PlotLegends -> Automatic], {t, 0, 
  80, 10}]

Growth of the fibrosis in a homogeneous tissue surrounding a bone. (another system similar to mine)

Is it possible? Could you please help me?

Any suggestions are very appreciated.

Improve this question
$\endgroup$
7
  • $\begingroup$ Have you tried solving it in 1D first, to see if that even works? $\endgroup$
    – Chris K
    Commented Dec 7, 2017 at 2:49
  • $\begingroup$ I changed the boundary conditions and could already solve the system. It takes approximately five minutes to solve. Thanks you all. $\endgroup$
    – Rodrigo López
    Commented Dec 8, 2017 at 4:49
  • $\begingroup$ I was looking at your profile and I realized that working with reaction-diffusion equations and biological populations. I working with cell populations and i have some questions about my system. I already solved the problem with BC but now I need to solve how those cell populations evolve over time arround a blood vessel (represented graphically as a circle) into a mesh. I used DensityPlot for to seehow the system of equations behaves in space and time but it is not what I expected and i don't know how represent a blood vessel (a circle, for example) into a mesh or in DensityPlot. $\endgroup$
    – Rodrigo López
    Commented Dec 11, 2017 at 22:34
  • $\begingroup$ I update my post $\endgroup$
    – Rodrigo López
    Commented Dec 11, 2017 at 23:24
  • $\begingroup$ Hi Rodrigo - most of my problems don't have a complex geometry underneath but I'll look at your edit. $\endgroup$
    – Chris K
    Commented Dec 11, 2017 at 23:54

1 Answer 1

Reset to default
2
$\begingroup$

To see how far you have progressed you can use something like this:

NDSolve[{eqns, IC, BC}, {fin, fit, mat, enz, oxi, glu}, {x, 0, 1}, {y,
   0, 1}, {t, 0, 80}
 , EvaluationMonitor :> (monitor = Row[{"t = ", CForm[t]}])
 ]

You can find more ideas here.

Perhaps integrating until a time where there is little progress and then looking at the result obtained that far will give you a clue on what is going on.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.