Mirroring graphics

Question

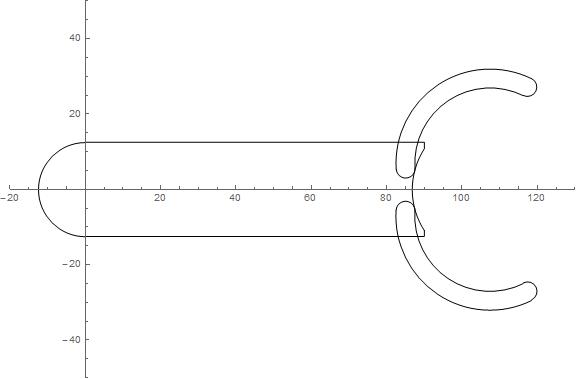
I am trying to tranform an object claw1 to create it mirror image claw2.

I tried using GeometricTransformation, but I did not get works. My code follows. How should I change it?

arm = {Circle[armCenter = {0, 0}, armR = 12.5, {Pi/2, 3*(Pi/2)}], 
   Circle[{106.79, 0}, 20, {2.57, 3.72}], 
       Line[{{0, armR}, {90, armR}, {90, armR}, {90, 10.87}}], 
   Line[{{0, -armR}, {90, -armR}, {90, -armR}, {90, -10.87}}]}; 
claw1 = {Circle[{106.79, 0}, 20, claw1a = {0.8, 2.89}], 
   Circle[{106.79, 0}, 25, claw1a], 
   Circle[{85, 5.6}, 2.5, claw1b = {2.89, 6.03}], 
       Circle[{122.54, 16.07}, 2.5, claw1c = {0.8, -2.35}]}; 
claw2 = {Circle[{106.79, 0}, 20, claw1a*-1], 
   Circle[{106.79, 0}, 25, claw1a*-1], 
   Circle[{85, -5.6}, 2.5, claw1b*-1], 
       Circle[{122.54, -16.07}, 2.5, claw1c*-1]}; 

angClaw1 = 
  GeometricTransformation[
   claw1, {RotationTransform[Pi/10, {85, 5.6}]}]; 
angClaw2 = 
  GeometricTransformation[
   claw2, {RotationTransform[-Pi/10, {85, -5.6}]}]; 

Graphics[{arm, angClaw1, angClaw2}, 
 PlotRange -> {{-20, 130}, {-50, 50}}, Axes -> True, 
 ImageSize -> Large]

Answer 1

You can do it with ReflectionTransform.

claw = 
  {Circle[{106.79, 0}, 20, claw1a = {0.8, 2.89}], 
   Circle[{106.79, 0}, 25, claw1a], 
   Circle[{85, 5.6}, 2.5, claw1b = {2.89, 6.03}], 
   Circle[{122.54, 16.07}, 2.5, claw1c = {0.8, -2.35}]};

The following code demonstrates the two claws rotating.

Manipulate[
  Graphics[
    {u = GeometricTransformation[claw, {RotationTransform[θ Degree, {85, 5.6}]}],
     GeometricTransformation[u, ReflectionTransform[{0, 1}, {85, 0}]],
     Dashed, InfiniteLine[{{0, 0}, {1, 0}}]},
     PlotRange -> {{75, 130}, {-45, 45}}, Frame -> True],
  {u, None},
  {θ, -15, 45, 1, Appearance -> "Labeled"}]