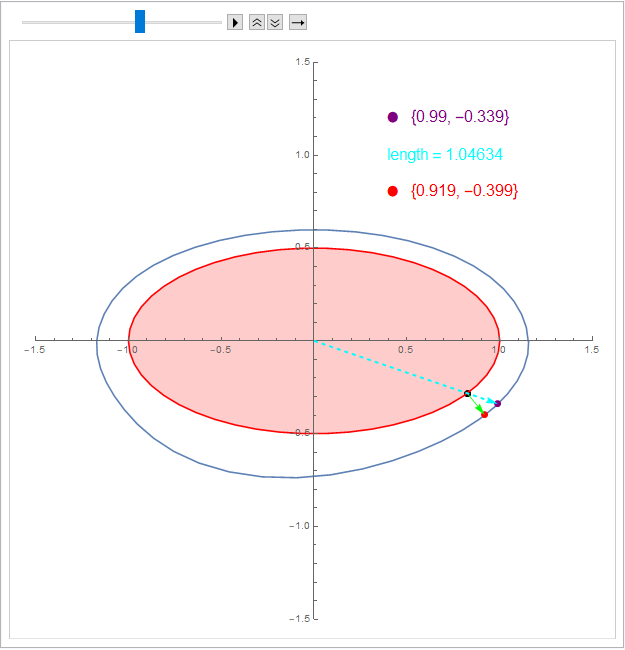
I have this code which animates a layer of fluid moving on the outside of an ellipse with a moving black point on the cylinder surface itself.
Is there a way to find the value of the blue outer line that is perpendicular to the ellipse surface at the point marked by the black dot as each stage of the animation?
The values for the code are:
cVals = {1., 0.992115, 0.968583, 0.929776, 0.876307, 0.809017, 0.728969, \
0.637424, 0.535827, 0.425779, 0.309017, 0.187381, 0.0627905, \
-0.0627905, -0.187381, -0.309017, -0.425779, -0.535827, -0.637424, \
-0.728969, -0.809017, -0.876307, -0.929776, -0.968583, -0.992115, \
-1., -0.992115, -0.968583, -0.929776, -0.876307, -0.809017, \
-0.728969, -0.637424, -0.535827, -0.425779, -0.309017, -0.187381, \
-0.0627905, 0.0627905, 0.187381, 0.309017, 0.425779, 0.535827, \
0.637424, 0.728969, 0.809017, 0.876307, 0.929776, 0.968583, 0.992115, \
1.}
sVals = {0., 0.125333, 0.24869, 0.368125, 0.481754, 0.587785, 0.684547, \
0.770513, 0.844328, 0.904827, 0.951057, 0.982287, 0.998027, 0.998027, \
0.982287, 0.951057, 0.904827, 0.844328, 0.770513, 0.684547, 0.587785, \
0.481754, 0.368125, 0.24869, 0.125333, 0., -0.125333, -0.24869, \
-0.368125, -0.481754, -0.587785, -0.684547, -0.770513, -0.844328, \
-0.904827, -0.951057, -0.982287, -0.998027, -0.998027, -0.982287, \
-0.951057, -0.904827, -0.844328, -0.770513, -0.684547, -0.587785, \
-0.481754, -0.368125, -0.24869, -0.125333, 0.}
solVals ={{0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25,
0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25,
0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25,
0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25,
0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25}, {0.231773, 0.223102,
0.21736, 0.215868, 0.218506, 0.223485, 0.228702, 0.232694, 0.235165,
0.236492, 0.237221, 0.237652, 0.237877, 0.237893, 0.237705,
0.237319, 0.236647, 0.235359, 0.232887, 0.22882, 0.223588, 0.218861,
0.21709, 0.220147, 0.227946, 0.23848, 0.249499, 0.259875, 0.269195,
0.276732, 0.281316, 0.282071, 0.27928, 0.27457, 0.269977, 0.266706,
0.264871, 0.264107, 0.264124, 0.264923, 0.266754, 0.269912,
0.274217, 0.278579, 0.28118, 0.280602, 0.276577, 0.269902, 0.261582,
0.252184, 0.241939}, {0.204681, 0.197575, 0.194278, 0.195382,
0.200351, 0.207264, 0.214157, 0.21958, 0.22322, 0.225323, 0.226494,
0.22712, 0.227439, 0.227497, 0.22732, 0.226864, 0.225938, 0.224082,
0.220664, 0.215287, 0.208395, 0.201638, 0.197536, 0.198316,
0.204589, 0.215152, 0.228384, 0.24353, 0.260356, 0.278019, 0.294529,
0.307096, 0.313128, 0.311555, 0.303816, 0.293681, 0.285306,
0.281068, 0.281492, 0.286386, 0.294783, 0.303971, 0.309975,
0.309662, 0.302287, 0.289238, 0.273117, 0.256413, 0.24085, 0.226948,
0.214759}, {0.184551, 0.180166, 0.17929, 0.182183, 0.188291,
0.195837, 0.203173, 0.208999, 0.21309, 0.215591, 0.217078, 0.217883,
0.218312, 0.218426, 0.218291, 0.217843, 0.216893, 0.21499,
0.211522, 0.206047, 0.198855, 0.191341, 0.185797, 0.184447,
0.188255, 0.196649, 0.208661, 0.224108, 0.243331, 0.266093,
0.290793, 0.314318, 0.332624, 0.342013, 0.34091, 0.331365, 0.319242,
0.31188, 0.313593, 0.322555, 0.332361, 0.33635, 0.331112, 0.316815,
0.296317, 0.273184, 0.250723, 0.230929, 0.214746, 0.201881,
0.191889}, {0.170798, 0.168322, 0.168874, 0.172614, 0.179096,
0.186693, 0.194032, 0.199914, 0.204201, 0.206938, 0.208671,
0.209649, 0.210204, 0.210387, 0.210306, 0.209903, 0.209037,
0.207303, 0.204131, 0.19904, 0.192168, 0.184658, 0.17854, 0.175899,
0.177817, 0.184073, 0.194152, 0.20832, 0.227447, 0.251961, 0.280949,
0.311696, 0.340004, 0.361346, 0.372725, 0.374318, 0.370007,
0.36535, 0.363685, 0.363153, 0.358975, 0.347301, 0.327639, 0.302198,
0.274711, 0.248424, 0.225697, 0.207332, 0.193424, 0.183113,
0.17575}, {0.160902, 0.159482, 0.160747, 0.16481, 0.171336,
0.178789, 0.186007, 0.191845, 0.19624, 0.199144, 0.201088, 0.202229,
0.202918, 0.203181, 0.203164, 0.202822, 0.202077, 0.200575,
0.197803, 0.193252, 0.18695, 0.179833, 0.173715, 0.170516, 0.171303,
0.175989, 0.184288, 0.196726, 0.214589, 0.239003, 0.270116,
0.306391, 0.344562, 0.380065, 0.408345, 0.425993, 0.431826,
0.426664, 0.412733, 0.392199, 0.366874, 0.338066, 0.307513,
0.277087, 0.248959, 0.224498, 0.204626, 0.189183, 0.177908,
0.169814, 0.164355}, {0.153071, 0.152184, 0.153786, 0.15795,
0.164411, 0.171684, 0.178768, 0.184543, 0.189019, 0.192057,
0.194191, 0.195485, 0.196314, 0.196663, 0.196721, 0.196446,
0.195826, 0.194555, 0.19218, 0.188181, 0.182521, 0.175988, 0.170226,
0.166971, 0.167173, 0.170712, 0.177389, 0.187948, 0.204232,
0.228522, 0.262741, 0.306994, 0.358345, 0.41036, 0.454678, 0.483194,
0.490829, 0.476829, 0.445276, 0.403247, 0.3586, 0.316921, 0.280894,
0.250584, 0.225525, 0.204925, 0.188572, 0.175861, 0.16662,
0.160002, 0.155699}, {0.146336, 0.145722, 0.147504, 0.151691,
0.158072, 0.165177, 0.172145, 0.177862, 0.182408, 0.185563,
0.187871, 0.189313, 0.190282, 0.190726, 0.190866, 0.190661,
0.190157, 0.189089, 0.187068, 0.183583, 0.178576, 0.172725,
0.167505, 0.164413, 0.164202, 0.16668, 0.171798, 0.180763, 0.1965,
0.223163, 0.2647, 0.321529, 0.388324, 0.454553, 0.507844, 0.537373,
0.537355, 0.508407, 0.457905, 0.39735, 0.338926, 0.290564, 0.254263,
0.227337, 0.206708, 0.190018, 0.176595, 0.165844, 0.157912,
0.152158, 0.148519}, {0.140236, 0.139792, 0.141697, 0.145893,
0.152202, 0.159163, 0.166033, 0.1717, 0.176311, 0.179571, 0.182043,
0.183626, 0.184738, 0.185283, 0.185513, 0.18538, 0.184979, 0.18408,
0.182366, 0.179362, 0.175013, 0.169879, 0.165205, 0.162181,
0.161369, 0.16266, 0.166476, 0.175034, 0.193067, 0.226941, 0.281132,
0.353204, 0.433414, 0.507461, 0.560878, 0.582599, 0.568315,
0.52147, 0.453123, 0.378515, 0.312536, 0.263423, 0.230779, 0.208764,
0.192438, 0.178814, 0.167391, 0.157832, 0.150658, 0.145386,
0.14215}, {0.134579, 0.134271, 0.136282, 0.140487, 0.146736,
0.153571, 0.160357, 0.165981, 0.170654, 0.174011, 0.176636,
0.178355, 0.179612, 0.180262, 0.18059, 0.180529, 0.180218, 0.17946,
0.178026, 0.1755, 0.17182, 0.167363, 0.163027, 0.159685, 0.157866,
0.157933, 0.161337, 0.172054, 0.197104, 0.243862, 0.313863,
0.399828, 0.488222, 0.562708, 0.608647, 0.616296, 0.584004,
0.518625, 0.43493, 0.351166, 0.283482, 0.238368, 0.211643, 0.194655,
0.181745, 0.170138, 0.159879, 0.150961, 0.144214, 0.139232,
0.136289}, {0.129289, 0.129107, 0.131217, 0.135432, 0.141628,
0.148348, 0.155059, 0.160644, 0.165376, 0.168822, 0.171593,
0.173445, 0.174845, 0.175607, 0.176037, 0.176048, 0.175815,
0.175184, 0.17403, 0.17201, 0.169, 0.165053, 0.160646, 0.156397,
0.153221, 0.152504, 0.157541, 0.174609, 0.212356, 0.275618,
0.360744, 0.456426, 0.546918, 0.615414, 0.64825, 0.637937, 0.586016,
0.50304, 0.407288, 0.31931, 0.254989, 0.217072, 0.196908, 0.184148,
0.173524, 0.163011, 0.153331, 0.144732, 0.13826, 0.133498,
0.130815}}
To build the plot I have used:
a = 1;
b = 0.5;
M = 0.1;
W = 1;
tbl = Map[Transpose[{a cVals + a cVals #, b sVals + b sVals #}] &,
solVals];
outerLayer =
Map[ListLinePlot[#, AspectRatio -> 1, ImageSize -> Large,
PlotRange -> {{-1.5, 1.5}, {-1.5, 1.5}}] &, tbl];
pointToTrack = 0;
movingpoint =
Table[ListPlot[
Transpose[{{a Cos[i M W - pointToTrack]}, {-b Sin[
i M W - pointToTrack]}}], AspectRatio -> 1,
PlotStyle -> Black, PlotMarkers -> {Automatic, Medium},
PlotRange -> {{-1.5, 1.5}, {-1.5, 1.5}}], {i, 0, 10}];
baseCylinder =
ListLinePlot[Transpose[{a cVals, b sVals}], PlotStyle -> Red,
Filling -> Axis, ImageSize -> Large, AspectRatio -> 1];
ListAnimate[
MapThread[Show[#1, #2, baseCylinder] &, {outerLayer, movingpoint}]]

I want to plot the values of the blue outer line that are perpendicular to the ellipse surface at the point marked by the moving black point on a separate graph later but I am unsure how to extract the values needed for this.
Any help/tips are greatly appreciated!
Edit:
Instead of finding the value above the moving point perpendicular to the surface, is there any way to find the length of a straight line from the center of the ellipse to the outer blue line passing through the moving point?