This is just a quick sketching out of an answer (rescales galore!)
textOnCurve[text_, f_, n_, p_: 0.01] :=
Text[Rotate[text, ArcTan @@ (f[Rescale[n + p, {0, 1}, {p, 1 - p}]] -
f[Rescale[n - p, {0, 1}, {p, 1 - p}]])], f[n]]
textCurve[string_, f_, stylef_: (# &), range_: {0, 1}] :=
With[{chars = Characters@string},
MapIndexed[textOnCurve[stylef@#1, f, Rescale[#2[[1]],{1, Length@chars}, range]] &, chars]]
Which can then be used like:
pts = {{0, 0}, {1, 1}, {2, -1}, {3, 0}};
LocatorPane[Dynamic[pts],
Dynamic@(
f = BezierFunction[pts];
Show[Graphics[{Point[pts], Line[pts],
textCurve["Some text here", f, Style[#, 20] &, {0.2, 0.6}]
}, Axes -> True]
, ParametricPlot[f[t], {t, 0, 1}]])
, LocatorAutoCreate -> True]
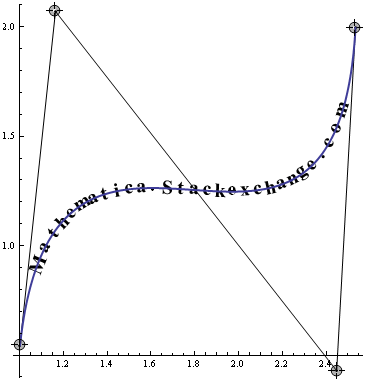
Update
This can be improved by adding proper positioning by fixing the lower midpoint in the rotation and position. Also using Szabolcs very nice equidistant spacings. However as I have stated in comments kerning is going to be trouble unless it's really taken seriusly into consideration.
textOnCurve[text_,f_,n_,p_: 0.01]:=
With[{angle=ArcTan@@Subtract@@(f/@Rescale[{n+p,n-p},{0,1},{p,1-p}])},
Rotate[Text[text,f[n],{0,-1}],angle,f[n]]
]
equidistantTextCurve[string_,f_,stylef_: (#&),range_: {0,1}]:=
Module[{chars,distance},
chars=Characters@string;
distance=functionEquidistant[f,Length@chars,range];
MapIndexed[textOnCurve[stylef@#1,f,distance[[#2[[1]]]]]&,chars]
]
LocatorPane[Dynamic[pts],
Dynamic@(f = BezierFunction[pts];
Show[Graphics[{Point[pts], Line[pts],
equidistantTextCurve["Mathematica.StackExchange.Com", f,
Style[#, 18] &, {0.15, 0.8}]
}, Frame -> True, PlotRange -> 2],
ParametricPlot[f[t], {t, 0, 1}]]), LocatorAutoCreate -> True]
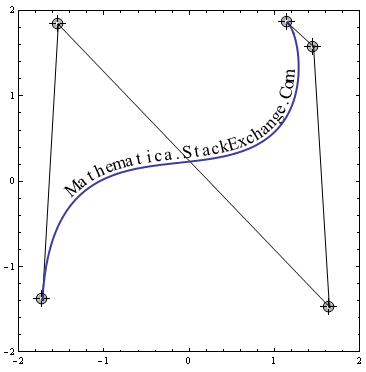
I'll leave it as an exercise to calculate proper kerning and getting an even better result.
