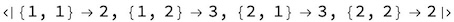Another way with ListCorrelate used with proper arguments, for example :
data = {1, 1, 1, 2, 1, 2, 2, 2, 1, 2, 1};
sub = {{1, 1}, {1, 2}, {2, 1}, {2, 2}};
Total@Boole@
ListCorrelate[#, data, {1, -1}, "paddingUselessHere", Equal, And] & /@ sub
{2, 3, 3, 2}
Another example
Let's say you're just looking for the longer pattern {1,2,1} :
Total@Boole@ListCorrelate[{1, 2, 1}, data, {1, -1}, "paddingUselessHere", Equal, And]
2
Explanation
It is easy to understand what ListCorrelate does with this example :
ListCorrelate[{p1, p2}, {a, b, c, d}, {1, -1}, "whatever", f, g]
{g[f[p1, a], f[p2, b]], g[f[p1, b], f[p2, c]], g[f[p1, c], f[p2, d]]}
(When the 4th argument of ListCorrelate is {1,-1} (default value), the 5th argument (padding) is useless, that's why i set it to "whatever".)