Optimization problems over the Integers

Question

Should be an easy question. How can I define a function which gets only integers in a form that I can use to find max and min for instance?

Example:

Find the Max of the expression (3 n + 4)/(2 n + 1) where n ∈ Integers

Answer 1

Diophantine problems are tough and there is no silver bullet. In your example this works:

i = IntegerPart; 
sol = NMaximize[{(3 i@ n + 4)/(2 i@n + 1), n > 1}, n]; 
i@n /. sol[[2]]

(* 1 *)

Answer 2

Bounding the range of n resolves the issue with Maximize

Maximize[{(3 n + 4)/(2 n + 1), Element[n, Integers], -100 <= n <= 100}, n]

{4, {n -> 0}}

Or,

Maximize[{(3 n + 4)/(2 n + 1), -100 <= n <= 100}, n, Integers]

{4, {n -> 0}}

Any large value for the range bound will work since

Limit[(3 n + 4)/(2 n + 1), n -> #] & /@ {Infinity, -Infinity}

{3/2, 3/2}

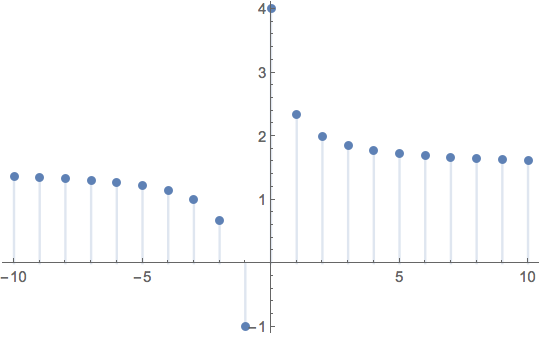
DiscretePlot[
 (3 n + 4)/(2 n + 1),
 {n, -10, 10},
 PlotRange -> All]