Region plot of transformed region giving odd result

Question

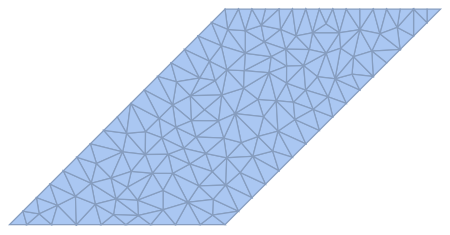
I tried applying a transformation to a rectangle:

RegionPlot[TransformedRegion[Rectangle[{0, 0}, {1, 1}], {#1^(1/3) + #2, 1 + #2} &]]

giving

In reality, it ought to be giving a parallelogram-shaped region, based on simply transforming a bunch of points:

Table[{#1^(1/3) + #2, 1 + #2} &[k, j], {k, 0, 1, 0.02}, {j, 0, 1, 
   0.02}] // ListPlot

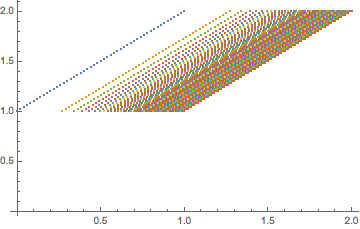
Any idea what's going wrong here? Am I using TransformedRegion wrong?

Answer 1

Looks to me to be a bug in RegionPlot. I say fhis beccause

DiscretizeRegion @ TransformedRegion[Rectangle[{0, 0}, {1, 1}], {#1^(1/3) + #2, 1 + #2} &]

gives

as expected.