I have a function that I'm trying to visualize and for some reason the plot only shows a small snippet of the graph of the function. The function, d, and relevant values are as follows:
G = 6.67*10^(-11)
c = 3*10^8
M = 10^(30)
J = 10^(20)
\[Gamma] = 0.018
rmin = M + Sqrt[M^2 - J^2/M^2]
d[r_, \[Theta]_] = 1 - (2 G M r)/(c^2 r^2 + J^2/M^2 Cos[\[Theta]]^2)
- (Sin[\[Theta]]^2 \[Gamma]^2)/c^2 (r^2 + J^2/(M^2 c^2) + ( 2 G J^2 r Sin[\[Theta]]^2)/(c^4 M (r^2 + J^2/(M^2 c^2) Cos[\[Theta]]^2)))
+ (8 \[Gamma] G r J Sin[\[Theta]]^2)/(c^4 (r^2 + J^2/(M^2 c^2) Cos[\[Theta]]^2))
The exact values of \[Gamma], M and J aren't particularly significant, although M and J are meant to be large. I'm trying to plot the graph of this function using Plot3D
Plot3D[
d[Sqrt[x^2 + y^2], ArcCos[x/Sqrt[x^2 + y^2]]],
{x, 0, 3 M},
{y,0, 6 M},
RegionFunction -> Function[{x, y, z}, rmin < Sqrt[x^2 + y^2] ]
]
I'm really new when it comes to working with mathematica, but I've seen online that sometimes varying PlotPoints and MaxRecursion can help to fix a plot. I tried setting PlotPoints -> {50, 100, 1000}, as well as MaxRecursion -> 10 (I don't if these values are high or low w.r.t Mathematica's default settings), changing these tended to make Mathematica really sluggish, moreover they didn't produce any more of the plot. I've also tried setting ClippingStyle -> None and PlotRange -> All and none of these had any effect.
I'm thinking that maybe It could be the large values that might be throwing Mathematica off, but I was under the impression that Mathematica could deal with 'astronomical numbers' (literally). Anyway if anybody has any insight into what's going wrong or just helpful advice it would be very much appreciated.
EDIT: In the comments it was mentioned that (0,0) is a potential problem, but I have an analogous function
M = 10
J = 1
\[Gamma] = 0.018
g[r_, \[Theta]_] = 1 - 2 M r/(r^2 + J^2/M^2 Cos[\[Theta]]^2)
- \[Gamma]^2 Sin[\[Theta]]^2 (r^2 + J^2/M^2 + 2 r J^2 Sin[\[Theta]]^2/(M (r^2 + J^2/M^2 Cos[\[Theta]]^2)))
+ \[Gamma] 8 r J Sin[\[Theta]]^2/(r^2 + J^2/M^2 Cos[\[Theta]]^2)
Which in principle is the same, and should encounter the same issue at (0,0), but Plot3D manages just fine.

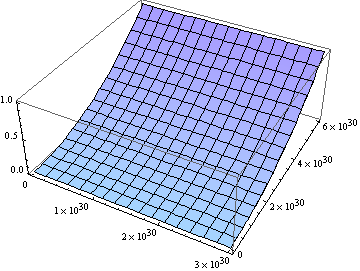
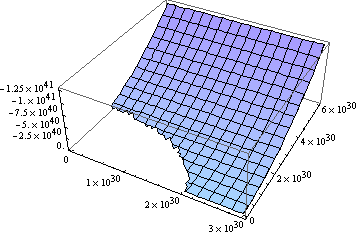
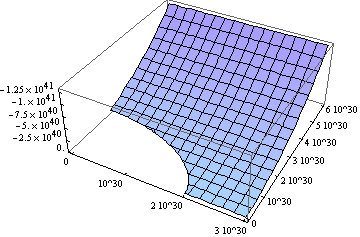
q... $\endgroup$qwas supposed to be ad, and it's true that at (0,0) there is a singularity, but doesn'tRegionFunctionexclude that point? $\endgroup$