How to interpolate an implicit function?

Question

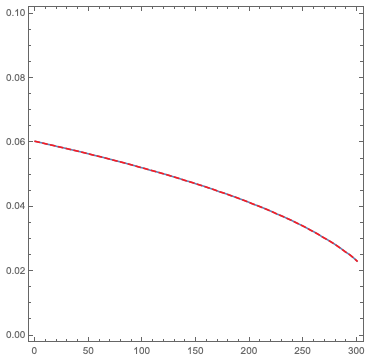
chi(t) is implicit function. The implicit function can be plotted by ContourPlot.

Is it possible to obtain chi(t) explicitly using function interpolation?
chi=Interpolation[...], t∈(0, 300)

ClearAll["Global`*"]

eq[chi_, t_] := 
  0.7*(t - 315)*(chi)^(1/1.6) + 
   0.008*(chi)^(1/1.6 + 1/0.4)*1000^(1/0.4) - 1;


ContourPlot[eq[chi, t] == 0, {t, 0, 300}, {chi, 0, 100*10^(-3)}]

Answer 1

Clear[chi, eq, t]

eq[chi_, t_] := 
  7/10*(t - 315)*(chi)^(10/16) + 8/1000*(chi)^(10/16 + 10/4)*1000^(10/4) - 1;

Solve for chi directly,

chi[t_] = Piecewise[List @@@ SolveValues[eq[c, t] == 0, c, Reals]];

Comparing the two plots

Show[
 ContourPlot[eq[chi, t] == 0, {t, 0, 300}, {chi, 0, 100*^-3}],
 Plot[chi[t], {t, 0, 300}, PlotStyle -> {{Red, Dashed}}]]

Answer 2

You can extract the points from the plot and interpolate them:

q[chi_, t_] = 0.7*(t - 315)*(chi)^(1/1.6) + 0.008*(chi)^(1/1.6 + 1/0.4)*1000^(1/0.4) - 1;
plt = ContourPlot[eq[chi, t] == 0, {t, 0, 300}, {chi, 0, 100*10^(-3)}];
pts = First@Cases[Normal@plt, Line[a_] :> a, Infinity];
f = Interpolation[pts, InterpolationOrder -> 1];
Show[plt, Plot[f[x], {x, 0, 300}, PlotStyle -> {{Red, Dashed}}]]