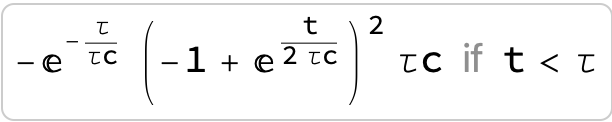Solving analytical integral

Question

I am trying to solve the following integral

\begin{equation} \left[\int_{0}^{t/2}d\tau-\int_{t/2}^{t}d\tau\right]\left[\int_{0}^{t/2}d\tau'e^{-\left|\tau-\tau'\right|/\tau_{c}}-\int_{t/2}^{t}d\tau'e^{-\left|\tau-\tau'\right|/\tau_{c}}\right] \end{equation}

using Mathematica, but I do not get any answer for it. Is there any trick to deal with this type of integrals?

Here is the implementation for the parentheses on the right

Integrate[Exp[-Abs[\[Tau]-\[Tau]p]/\[Tau]c], {\[Tau]p, 0, t/2}]-  Integrate[Exp[-Abs[\[Tau]-\[Tau]p]/\[Tau]c], {\[Tau]p, t/2, t}]

Thanks!

Answer 1

If you add assumptions that variables are positive, then

Assuming[{t > 0, τc > 0, τ > 0},
 Integrate[
    Exp[-Abs[τ - τp]/τc] // ComplexExpand, {τp, 0, t/2}] -
   Integrate[
    Exp[-Abs[τ - τp]/τc] // ComplexExpand, {τp, t/2, t}] //
   FullSimplify]