Why VertexCoordinate does not work here?

Question

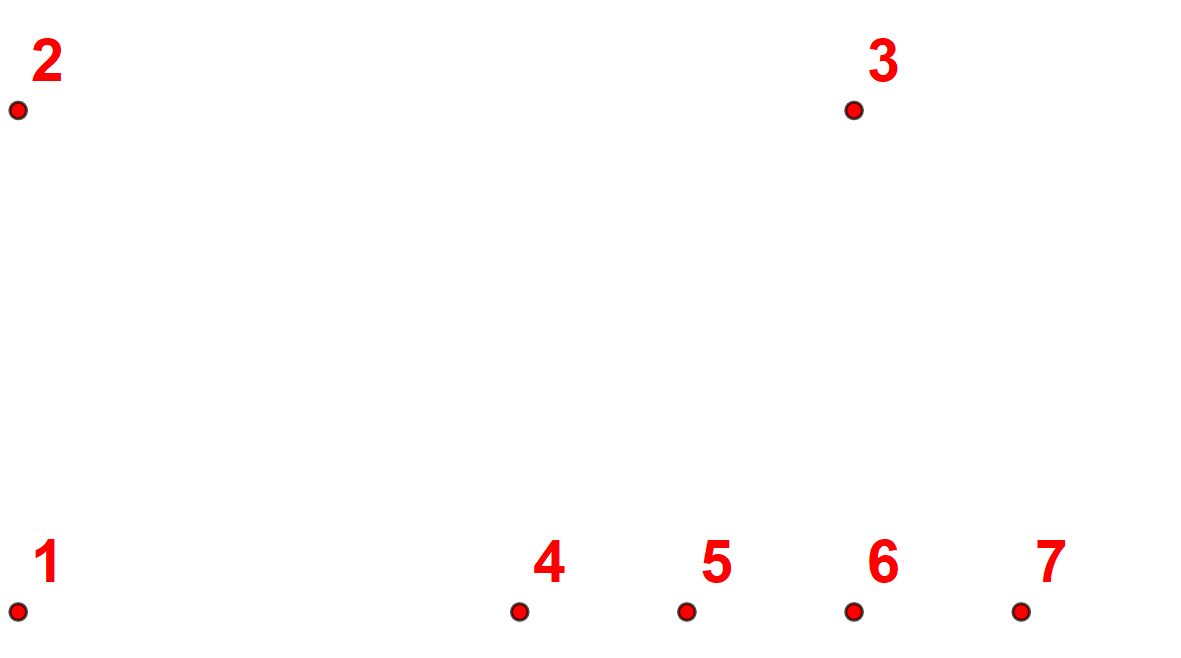
First I plotted this and got a nice graph.

Graph[Range[7], {},
VertexLabels ->
Thread[Range[7] -> (Style[#, Red, 20, Bold] & /@ Range[7])],
VertexSize -> Small, VertexStyle -> Red,
VertexCoordinates -> {1 -> {0, 0}, 2 -> {0, 3}, 3 -> {5, 3}},
EdgeStyle -> Directive[Green, Thickness[0.05]],
EdgeLabelStyle -> Directive[Blue, Bold, 50]]

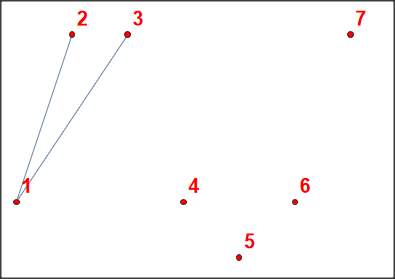
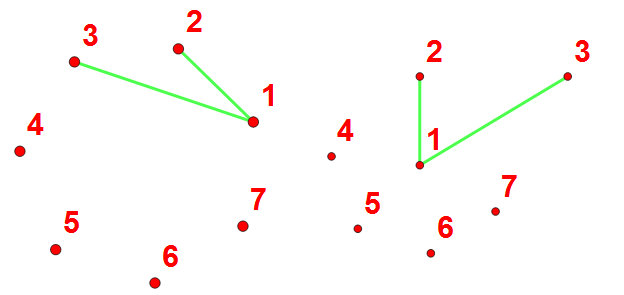
Next I added two edges and it ruined my graph. The vertexcoordinate doesn't seem to work anymore. Does anyone know why and how to fix this?

Graph[Range[7], {1 \[UndirectedEdge] 2, 1 \[UndirectedEdge] 3},
VertexLabels ->
Thread[Range[7] -> (Style[#, Red, 20, Bold] & /@ Range[7])],
VertexSize -> Small, VertexStyle -> Red,
VertexCoordinates -> {1 -> {0, 0}, 2 -> {0, 3}, 3 -> {5, 3}},
EdgeStyle -> Directive[Green, Thickness[0.05]],
EdgeLabelStyle -> Directive[Blue, Bold, 50]]

Answer 1

The problem is, that MMA chooses ridiculous large coordinates for vertices without explicitly given coordinates. Why, I do not know. If you specify all coordinates explicitely, you do not have a problem. E.g.

Graph[Range[7], {1 \[UndirectedEdge] 2, 1 \[UndirectedEdge] 3}, 
  VertexLabels -> 
   Thread[Range[7] -> (Style[#, Red, 20, Bold] & /@ Range[7])], 
  VertexSize -> Small, VertexStyle -> Red, 
  VertexCoordinates -> Table[i -> {i, Mod[i^2, 5]}, {i, 7}]]

Answer 2

Define (and visualize) points on a circle with radius 3 (arbitrarily chosen and starting at 20 degrees).

cpts = CirclePoints[{3, 20 Degree}, 7]
Graphics[{Red, AbsolutePointSize[5], Point@cpts}, ImageSize -> Small]

Now use these points as VertexCoordinates. In the first variation, use the cpts.

Graph[Range[7], {1 \[UndirectedEdge] 2, 1 \[UndirectedEdge] 3}
 , VertexLabels -> 
  Thread[Range[7] -> (Style[#, Red, 20, Bold] & /@ Range[7])]
 , VertexCoordinates -> cpts
 , VertexSize -> Small
 , VertexStyle -> Red
 , EdgeStyle -> Directive[Green, AbsoluteThickness[2]]
 , EdgeLabelStyle -> Directive[Blue, Bold, 50]
 (*,PlotRange\[Rule]{{-6,6},{-6,6}}*)
 , ImageSize -> Small
 ]

For the second variation utilize the three given points and join the rest from the cpts.

Graph[Range[7], {1 \[UndirectedEdge] 2, 1 \[UndirectedEdge] 3}
 , VertexLabels -> 
  Thread[Range[7] -> (Style[#, Red, 20, Bold] & /@ Range[7])]
 , VertexCoordinates -> {1 -> {0, 0}, 2 -> {0, 3}, 3 -> {5, 3}}~Join~
   MapThread[Rule, {{4, 5, 6, 7}, cpts[[-4 ;; -1]]}]
 , VertexSize -> Small
 , VertexStyle -> Red
 , EdgeStyle -> Directive[Green, AbsoluteThickness[2]]
 , EdgeLabelStyle -> Directive[Blue, Bold, 50]
 , ImageSize -> Small
 ]

---

Result: