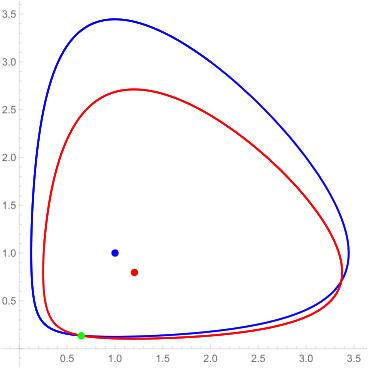
I have to solve the next problem: We know that a=b=c=d=1 and x0=2,y0=3,q=0.2,On the t=[0,20] interval there is no fishing but on the t=[20,40] interval there is.Show the adequate trajectory(the first one is blue,the second is red)the equilibrium points(blue and red),and the point coresponding to the transition(green).
And after solving this we should get the next graph:
Here is my take on it,but for some reason the red trajectory is off and I don't know how to find the green point.
a = b = c = d = 1;
x0 = 2;
y0 = 3;
q = 0.2;
egyensulypont1 =
Graphics[{Blue, Thick, PointSize[0.02], Point[{c/d, a/b}]}];
egyensulypont2 =
Graphics[{Red, Thick, PointSize[0.02],
Point[{(c + q)/d, (a - q)/b}]}];
Soln = NDSolve[{x'[t] == a*x[t] - b*x[t]*y[t],
y'[t] == -c*y[t] + d*x[t]*y[t], x[0] == x0, y[0] == y0}, {x,
y}, {t, 0, 20}]
Solh = NDSolve[{x'[t] == (a - q)*x[t] - b*x[t]*y[t],
y'[t] == -(c + q)*y[t] + d*x[t]*y[t], x[0] == x0, y[0] == y0}, {x,
y}, {t, 20, 40}]
Show[ParametricPlot[{x[t], y[t]} /. Soln, {t, 0, 20},
PlotStyle -> Blue],
ParametricPlot[{x[t], y[t]} /. Solh, {t, 20, 40},
PlotStyle -> Red], egyensulypont1, egyensulypont2]

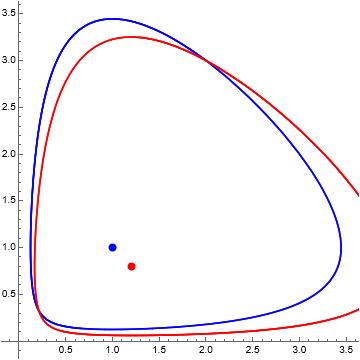
Solnas the initial condition ofSolh, instead of using the same initial condition of{x0, y0}. That is also the green point. $\endgroup$