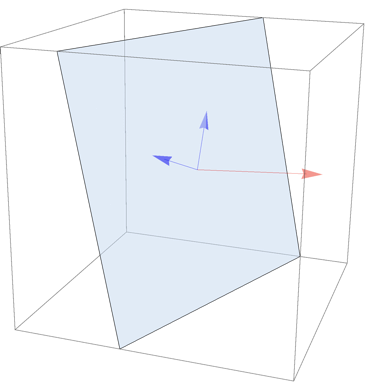
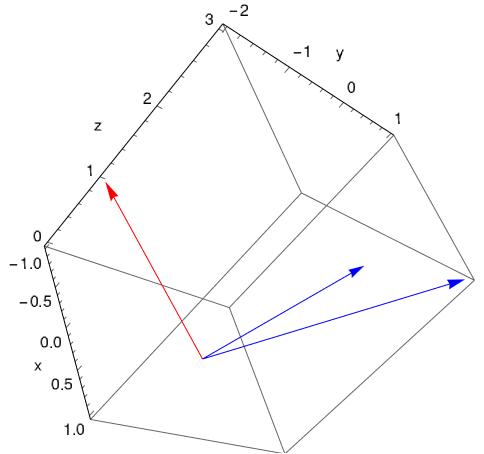
I'm trying to plot a normal vector to a plane. I know I'm doing something I should know better, but can't seem to find. Vectors {0, 1, 2}, {1, 1, 3} obviously determine a plane. Their Cross Product is {1, 2, -1}, which is normal. Unfortunately this code (all from the origin):
Graphics3D[{{Blue, Arrow[{{0, 0, 0}, {0, 1, 2}}]}, {Red, Arrow[{{0, 0, 0}, {-1, -2, 1}}]},
{Blue, Arrow[{{0, 0, 0}, {1, 1, 3}}]}}]
Yields,
Which doesn't look quite right. Just don't trust pictures? Any thoughts appreciated. Trying a different way I found some Mathematica code from a multivariable course for normals to a plane... and I got the same thing...


BoxRatios. $\endgroup$