0
$\begingroup$

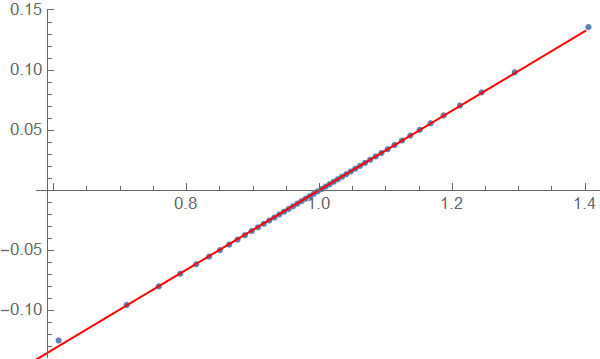
I have these point

p = {1.4048301787418027` + 0.13589831076513692` I, 1.2937864859657142` + 0.09826442126033227` I, 1.2439169436341522` + 0.08152085090585755` I, 1.2112251127756706` + 0.07057066521001881` I, 1.1867797359572914` + 0.06239213624408477` I, 1.1671819237029237` + 0.05584007186977612` I, 1.1507678762649645` + 0.050355104251339586` I, 1.1365972662436867` + 0.04562153217223474` I, 1.124085953294084` + 0.041443428931470704` I, 1.1128457075115394` + 0.037690672840169796` I, 1.1026049923874517` + 0.034272306091684974` I, 1.0931660902037226` + 0.031122129476503592` I, 1.0843802257181003` + 0.028190350543236032` I, 1.076132312976041` + 0.025438465710119672` I, 1.0683311706306955` + 0.02283597890972666` I, 1.060903003028386` + 0.020358216403672635` I, 1.053786913489549` + 0.017984823404026413` I, 1.0469317264511402` + 0.015698699656082248` I, 1.0402936772643052` + 0.013485225924340693` I, 1.0338346910665444` + 0.011331687936209292` I, 1.027521069271944` + 0.009226836941693186` I, 1.021322461994918` + 0.007160546106256114` I, 1.0152110424227831` + 0.0051235346034142525` I, 1.0091608233679767` + 0.003107139398530981` I, 1.0031470719276647` + 0.0011031199836812119` I, 0.9971457882879402` - 0.0008965152800982101` I, 0.9911332209734355` - 0.0028996704469104913` I, 0.9850853942726508` - 0.004914318965930066` I, 0.9789776246938359` - 0.006948664028432134` I, 0.9727840022953297` - 0.009011310260944524` I, 0.9664768094358419` - 0.011111455803159562` I, 0.9600258433964858` - 0.013259115782829277` I, 0.9533975994565845` - 0.015465391402985825` I, 0.9465542556662075` - 0.017742803834833825` I, 0.9394523769004093` - 0.02010571977953537` I, 0.9320412189796319` - 0.022570907472936778` I, 0.9242604553685143` - 0.025158280726805794` I, 0.9160370544286163` - 0.027891919050845516` I, 0.9072808772098193` - 0.030801502627903685` I, 0.8978782922954255` - 0.03392438838789599` I, 0.8876826109570178` - 0.037308710478383814` I, 0.8764992119353089` - 0.041018184124120435` I, 0.864061351522674` - 0.045139880920104784` I, 0.8499886226970275` - 0.04979749980583017` I, 0.8337105619927074` - 0.05517557183546196` I, 0.814313066911355` - 0.06156755652317412` I, 0.7901896657264539` - 0.0694831242611486` I, 0.7580949362489926` - 0.07993065003390927` I, 0.7096897586662804` - 0.09538398209765551` I, 0.6071597345880032` - 0.1250060626682752` I}

Now I did

sol = FindFit[p, a x + b, {a, b}, x];
eqnR[x_] := a x + b /. sol;
eqnR[x]

Which gives me the output

(1.24601 + 0.0818429 I) - (0.00962482 + 0.00319108 I) x

I want to plot the list plot by doing

ListPlot[Transpose[{Re[p],Im[p]}]]

and also plot the straight line from the find-fit given by eqnR[x] on the same plot.

But I am not sure how to plot a straight line with complex parameters. Any suggestions?

This is the list plot. What I would like to have is a plot of straight-line fitting all these points. enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

You can try

     re=Re[eqnR[x]];
     im=Im[eqnR[x]];
     ParametricPlot[{re,im},{x,-100,100]
Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Note: ParametricPlot[ReIm[eqnR[x]],{x,-100,100] gives the same behavior! $\endgroup$
    – thorimur
    Commented Mar 27, 2021 at 2:02
3
$\begingroup$

We use ReIm@p to get the real pairs then use FindFit.

sol1 = FindFit[ReIm@p, a x + b, {a, b}, x];
eqnR1[x_] := a x + b /. sol1;
eqnR1[x]
Show[ListPlot[ReIm@p, Joined -> False], 
 Plot[eqnR1[x], {x, 0, 1.4}, PlotStyle -> Red]]

enter image description here

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ eqnR is complex-valued, though, so I'm not sure this works... $\endgroup$
    – thorimur
    Commented Mar 27, 2021 at 2:04
  • $\begingroup$ @thorimur Here we doesn't use complex fit. $\endgroup$
    – cvgmt
    Commented Mar 27, 2021 at 2:14
  • $\begingroup$ ah, I see, so it's a different eqnR. Makes sense. $\endgroup$
    – thorimur
    Commented Mar 27, 2021 at 2:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.