How can I use NDSolve when running out of RAM?
The code I am implementing:
sol = NDSolve[ pdes, {A, B, C},
{x, -L, L}, {t, Tf, Tf} ]
in order to obtain the end solution of NDSolve to be exported to the next run (due to limited RAM).
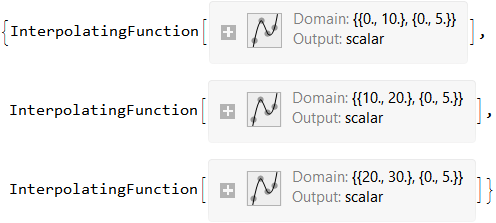
In previous attempts the solution of NDSolve was exported as an interpolating function, such as:
Export["sol_Tf.wdx",sol]
sol_Tf.wdx is the export file of the NDSolve run.
Also see my previous question.
I then imported it as:
Import["sol_Tf.wdx"]
and used the boundary conditions:
A[x, Ti] == A[x, Tf]
B[x, Ti] == B[x, Tf]
C[x, Ti] == C[x, Tf]
But the results were different from running it all in one go. What went wrong here? Is there a better way of doing this?
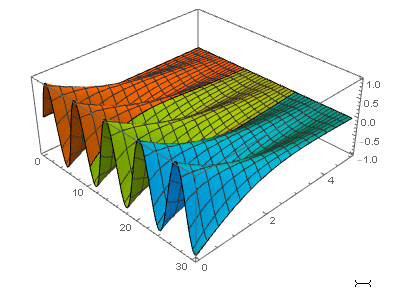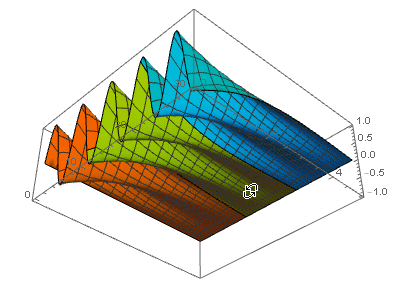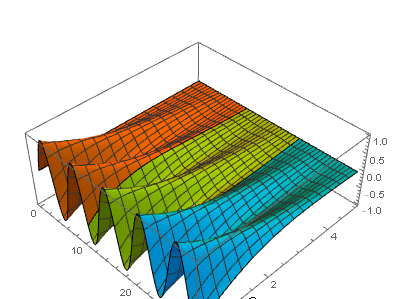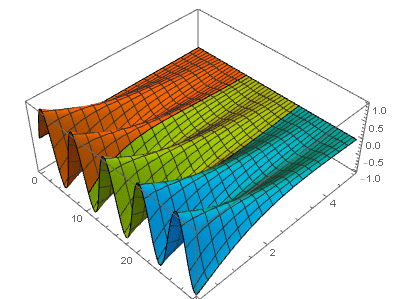
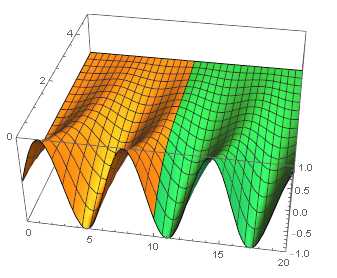
For example, in the heat equation:
sol = NDSolve[{D[u[t, x], t] == D[u[t, x], x, x], u[0, x] == 0,
u[t, 0] == Sin[t], u[t, 5] == 0}, u, {t, 0, 10}, {x, 0, 5}]
I would want to continue it with the end results of the previous run, as the new boundary conditions of the next run; RAM free, and by running it again from t = 10 to t = 20.