I was working on a project about optimal strategies for HIV treatment, models used from [Butler, Kirschner, and Lenhart] 1997. This model explains the spread of HIV viruses in the human body, where there is one control function u(t).
My work is following pontryagin maximum principle. But i have a problem solving the differential equation system, where there are 6 differential equations with 6 initial conditions. Here i use software Mathematica 11 when I execute the code there is no error that appears and he just returning DSolve code that i write.
ClearAll["Global`*"];
dT = s/(1 + V[t]) - m1 T[t] + r T[t] (1 - (T[t] + Ti[t])/Tm) -
u[t] k V[t] T[t];
dTi = u[t] k V[t] T[t] - m2 Ti[t];
dV = M m2 Ti[t] - m3 V[t];
H = A T[t] - (1 - u[t])^2 + l1[t] dT + l2[t] dTi + l3[t] dV ;
u[t] = 1/2 (2 - k l1[t] T[t] V[t] + k l2[t] T[t] V[t])
eq1 = D[l1[t], t] == D[H, l1[t]];
eq2 = D[l2[t], t] == D[H, l2[t]];
eq3 = D[l3[t], t] == D[H, l3[t]];
eq4 = D[T[t], t] == -D[H, T[t]];
eq5 = D[Ti[t], t] == -D[H, Ti[t]];
eq6 = D[V[t], t] == -D[H, V[t]];
s = 10; m1 = 0.02; m2 = 0.5; m3 = 4.4; r = 0.03; Tm = 1500; k = \
0.000024; M = 300; A = 1;
DSolve[{eq1, eq2, eq3, eq4, eq5, eq6, T[0] == 800, Ti[0] == 0.04,
V[0] == 1.5, l1[20] == 0, l2[20] == 0, l3[20] == 0}, {l1[t], l2[t],
l3[t], T[t], Ti[t], V[t]}, t]
I cannot guarantee that the analytical solution exists, but is there something wrong with code I wrote? Or is there an alternative solution to complete the differential equation system?
I hope you are pleased to check the file that I attached. Control-Optimal.nb
Many thanks




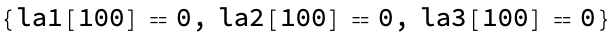
NDSolve. I noticed that the equations forT'[t],Ti'[t]andV'[t]in your code do not match the system you want to solve. In any case, you might want to include all your code in the question in case people do not want to download a notebook. $\endgroup$