This sort of post hoc reasoning behind a decision made by a group of developers is a little dangerous. I think a better phrasing of the question might be, What are the advantages of NDSolveValue over NDSolve, given that NDSolveValue returns only one of the solutions computed by NDSolve? Encouraged by @Szabolcs and by the upvotes on my oversimplified comment, here goes....
I think the primary use of NDSolveValue (singular, not plural NDSolveValues) is to return a single function ifn (or function expression ifn[x]) that can be evaluated on values of the independent variable(s). @Carl Woll has already discussed some aspects of the convenience of this, but I would add that this use probably occurs many more times in practice than all other uses put together, because so many useful ODEs are of the form $y^{(n)} = F\big(x,y,y',\dots,y^{(n-1)}\big)$, usually just $y'=F(x,y)$ or $y''=F(x,y,y')$. NDSolve will return a single solution to a valid IVP for such an ODE, and NDSolveValue therefore will also return the only solution.
My comment suggested that Values@NDSolve[..] would be equivalent to a "plural" form of NDSolveValue, but that's not exactly right. NDSolveValue is much more general in what it can return, except that its return value will be based on the first solution returned by NDSolve, other solutions being ignored. AFAICT, the following two lines are equivalent:
NDSolveValue[<IVP>, expr, <INTERVAL>]
expr /. First@NDSolve[<IVP>, <VARIABLES>, <INTERVAL>]
It seems the expression expr can be anything. If expr = 2, then 2 is returned; if expr = Plot[Sin[t], {t, 0, 10}], then the plot is returned, warnings aside. As far as Values@NDSolve[..] goes, the equivalence limited:
vnds = Values@ NDSolve[{x'[t]^2 == x[t]^2, x[0] == 1}, {x}, {t, 0, 1}];
ndsv = NDSolveValue[{x'[t]^2 == x[t]^2, x[0] == 1}, {x}, {t, 0, 1}];
First[vnds] === ndsv
(* True *)
vnds = Values@ NDSolve[{x'[t]^2 == x[t]^2, x[0] == 1}, x, {t, 0, 1}];
ndsv = NDSolveValue[{x'[t]^2 == x[t]^2, x[0] == 1}, x, {t, 0, 1}];
First[vnds] === ndsv
First@First[vnds] === ndsv
(*
False
True
*)
They are equivalent if expr = {x} -- that is, if NDSolveValue returns the value of the list {x}, but not if NDSolveValue returns just the function x.
Instead of focusing on the issue of the lost solutions, one should rather think of the advantages of having some syntactic sugar equivalent to expr /. First@NDSolve[..]. A couple favorites:
ListLinePlot@NDSolveValue[ivp, x, {t,..}] (* equiv. to Plot[x[t], {t,..}] *)
NSolveValue[{y'[x] == f[x], y[a] == 0}, (* equiv. to NIntegrate[f[x], {x, a, b}] *)
y[b], {x, a, b}]
Those are aside from the highly useful InterpolatingFunction returned by NDSolveValue[ivp, x, {t, 0, 1}]. I think almost everyone who uses ODEs a lot hates always having to remember to type First in x[t] /. First[sol].
OTOH, if you are analyzing a solution, I find sol = NDSolve[ivp, x,..] convenient for plugging into the ODE, invariants and so forth.
Workarounds:
(1)
If you want an NDSolveValues[] or NDSolveValueList[], try this:
ClearAll[NDSolveValueList];
NDSolveValueList[sys_, expr_, indeps : {_, _, _} .., rest___] :=
Module[{sol, deps},
deps = Internal`ProcessEquations`FindDependentVariables[
Flatten@{sys}, {indeps}[[All, 1]]];
sol = NDSolve[sys, deps, indeps, rest];
(expr /. sol) /; FreeQ[sol, NDSolve]
];
I don't know much about Internal`ProcessEquations`FindDependentVariables, so it might not work in all cases. Alternatively, for non-PDE systems, one might use the following, which uses documented functions:
ClearAll[NDSolveValueList];
NDSolveValueList[sys_, expr_, {t_, a_, b_}, opts___?OptionQ] :=
Module[{state, states},
states = NDSolve`ProcessEquations[sys, expr, {t, a, b}, opts];
Table[
state = states[[i]];
NDSolve`Iterate[state, {a, b}];
First@Values@NDSolve`ProcessSolutions@state,
{i, Length@states}] /; FreeQ[states, $Failed]
];
If there's a way to get the time-integration interval {a, b} from state, it does not seem to be documented. If someone finds or knows it, then this could probably be extended to PDEs with ease. The data is inside state.
(2)
If you want simply to get a different solution from NDSolveValue, there are two approaches. Both determine the solution by the highest derivative value. One uses the method "EquationSimplification" -> "Residual", and the other differentiates the ODE so that the derivative value becomes an initial condition.
NDSolveValue[
{x'[t]^2 == x[t]^2,
{x[0], x'[0]} == Values@
Solve[{x'[t]^2 == x[t]^2, x[0] == 1} /. t -> 0, {x'[0], x[0]}][[2]]},
x, {t, 0, 1},
Method -> {Automatic, "EquationSimplification" -> "Residual"}]
NDSolveValue[
{D[x'[t]^2 == x[t]^2, t],
{x[0], x'[0]} == Values@
Solve[{x'[t]^2 == x[t]^2, x[0] == 1} /. t -> 0, {x'[0], x[0]}][[2]]},
x, {t, 0, 1}]

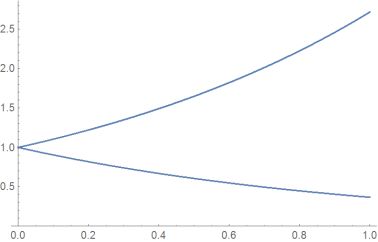
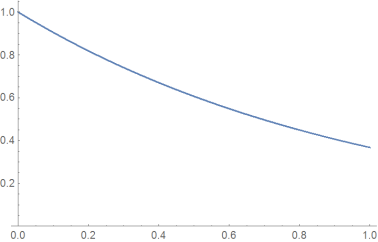

NDSolveValuespits outndsvbwarning, and I think the warning message is quite clear. And as mentioned by J.A in the answer below, this has been mentioned in the possible issues ofNDSolveValue, at least from v11.2. $\endgroup$Values@NSolve[..]....It's not clear to me what's hard to understand about the convenience of having a call that returns a functionfthat you can apply directly to inputf[0.2]and so forth. You can't do that if a list of functions is returned, but have to useThrough[f[0.2]]or some other workaround. $\endgroup$listx=Values@NSolve[..]exactly returns what I would expect fromNDSolveValues: A list of pure functions!!! The single solutions in listx can be addressed through listx[index][t] $\endgroup$