How to plot Bernouli Differential equation?

Question

I have 2 questions.

Bernoulli differential equation: $ y'(x)-y(x)=\dfrac{\mathrm e^x}{y(x)} $

When solving this step by step following this Clay Roose book [page 81, Example 3.13(M)], I get one solution $ \sqrt{\mathrm e^x \left(c_1 \mathrm e^x-2\right)} $.

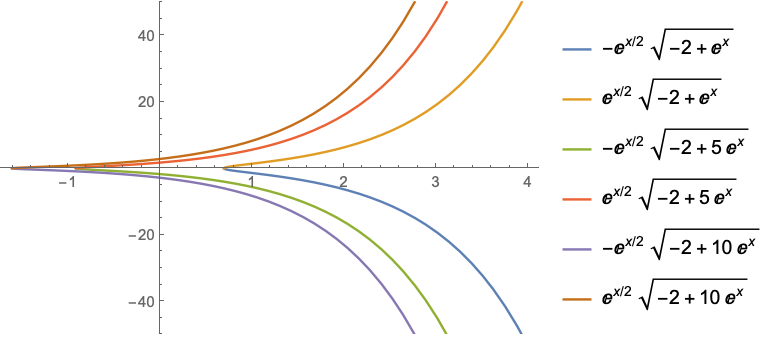
When i solve this with DSolve, I get $ \left\{\left\{y(x)\to -\mathrm e^{x/2} \sqrt{c_1 \mathrm e^x-2}\right\}, \left\{y(x)\to \mathrm e^{x/2} \sqrt{c_1 \mathrm e^x-2}\right\}\right\} $.

Questions are:

1. Why is there difference and where is mistake in solving step by step?

2. I know how to plot if I am going step by step, but how to plot solve which I get with DSolve because there are 2x branches of solve?

Answer 1

eqn = y'[x] - y[x] == E^x/y[x];

sol = DSolve[eqn, y, x]

(* {{y -> Function[{x}, -E^(x/2) Sqrt[-2 + E^x C[1]]]}, {y -> 
   Function[{x}, E^(x/2) Sqrt[-2 + E^x C[1]]]}} *)

Both solutions satisfy the equation

eqn /. sol // Simplify

(* {True, True} *)

Plot[Evaluate[
  Table[Tooltip[y[x] /. sol /. C[1] -> c], {c, {1, 5, 10}}]], {x, -2, 4},
 PlotRange -> {-50, 50}, PlotLegends -> "Expressions"]