How to remove the 2 same plot from arrays of plot

Question

ClearAll["Global`*"];
Clear[b]
L = 4;
z1 = L/3;
Y = 2*10^11;
Iyy = 8.333*10^-6;
A = 0.1^2;
kbt = (Y*Iyy)/L^3;
kbr = (Y*Iyy)/L;
\[Rho] = 7850;
mb = 7850*A*L;
w1 = A1*Sin[b*x] + B1*Cos[b*x] + C1*Sinh[b*x] + E1*Cosh[b*x];
w2 = A2*Sin[b*(x - z1)] + B2*Cos[b*(x - z1)] + C2*Sinh[b*(x - z1)] + 
   E2*Cosh[b*(x - z1)];
w = Piecewise[{{w1, x <= z1}, {w2, x > z1}}];
(*SS BC*)
bcd0 = w1 /. {x -> 0};
bcm0 = (D[w1, {x, 2}]) /. {x -> 0};
bcdl = w2 /. {x -> L};
bcml = D[w2, {x, 2}] /. {x -> L};
(*Compatability condition for translation spring*)
ccd1 = (w1 /. {x -> z1}) - (w2 /. {x -> z1});
ccs1 = ((D[w1, {x}]) /. {x -> z1}) - ((D[w2, {x}]) /. {x -> z1});
ccm1 = ((D[w1, {x, 2}]) /. {x -> z1}) - ((D[w2, {x, 2}]) /. {x -> z1});
ccsh1 = ((D[w1, {x, 3}]) /. {x -> z1}) - ((D[w2, {x, 3}]) /. {x -> 
       z1}) + KT*(w1 /. {x -> z1});
(*Compatability condition for rotational spring*)
ccd2 = (w1 /. {x -> z1}) - (w2 /. {x -> z1});
ccs2 = ((D[w1, {x}]) /. {x -> z1}) - ((D[w2, {x}]) /. {x -> z1});
ccm2 = ((D[w1, {x, 2}]) /. {x -> z1}) - ((D[w2, {x, 2}]) /. {x -> 
       z1}) + KR*((D[w1, {x, 1}]) /. {x -> z1});
ccsh2 = ((D[w1, {x, 3}]) /. {x -> z1}) - ((D[w2, {x, 3}]) /. {x -> 
       z1});

(*Forming matrix for translational springs *)

RT = Normal@
   CoefficientArrays[{bcd0, bcm0, bcdl, bcml, ccd1, ccs1, ccm1, 
      ccsh1}, {A1, B1, C1, E1, A2, B2, C2, E2}][[2]];
R1 = MatrixForm[RT];
MatrixRank[RT];
P1 = FullSimplify[Det[RT]];
f[k1t_, beta_] := 
 Module[{m}, KT = k1t; r = beta; s1 = P1; 
  s2 = NSolve[s1 == 0 && 0 < b < 30]; s3 = b /. s2; s4 = Flatten[s3]; 
  s5 = s4[[r]]; {uu, ww, vv} = 
   SingularValueDecomposition[RT /. b -> s5];
  NN = Last[Transpose[vv]]; A1 = NN[ [1]]; B1 = NN[ [2]]; 
  C1 = NN[ [3]]; E1 = NN[ [4]]; A2 = NN[ [5]]; B2 = NN[ [6]]; 
  C2 = NN[ [7]]; E2 = NN[ [8]]; m = w /. b -> s5; Return[m]]

(*Forming matrix for rotational springs *)

Clear[b, A1, B1, C1, E1, A2, B2, C2, E2]
RR = Normal@
   CoefficientArrays[{bcd0, bcm0, bcdl, bcml, ccd2, ccs2, ccm2, 
      ccsh2}, {A1, B1, C1, E1, A2, B2, C2, E2}][[2]];
R2 = MatrixForm[RR];
MatrixRank[RR];
P2 = FullSimplify[Det[RR]];
g[k1r_, beta_] := 
 Module[{m}, KR = k1r; r = beta; s1 = P2; 
  s2 = NSolve[s1 == 0 && 0 < b < 30]; s3 = b /. s2; s4 = Flatten[s3]; 
  s5 = s4[[r]]; {uu, ww, vv} = 
   SingularValueDecomposition[RR /. b -> s5];
  NN = Last[Transpose[vv]]; A1 = NN[ [1]]; B1 = NN[ [2]]; 
  C1 = NN[ [3]]; E1 = NN[ [4]]; A2 = NN[ [5]]; B2 = NN[ [6]]; 
  C2 = NN[ [7]]; E2 = NN[ [8]]; m = w /. b -> s5; Return[m]]
n1 = 1;
n2 = 5;
soft = Table[f[0, i], {i, n1, n2}];
hardL = Table[f[1*^12, i], {i, n1, n2}];
hardR = Table[g[1*^12, i], {i, n1, n2}];
dim = Length[soft]*2 + Length[hardR];
modes = Flatten[{soft, hardL, hardR}];
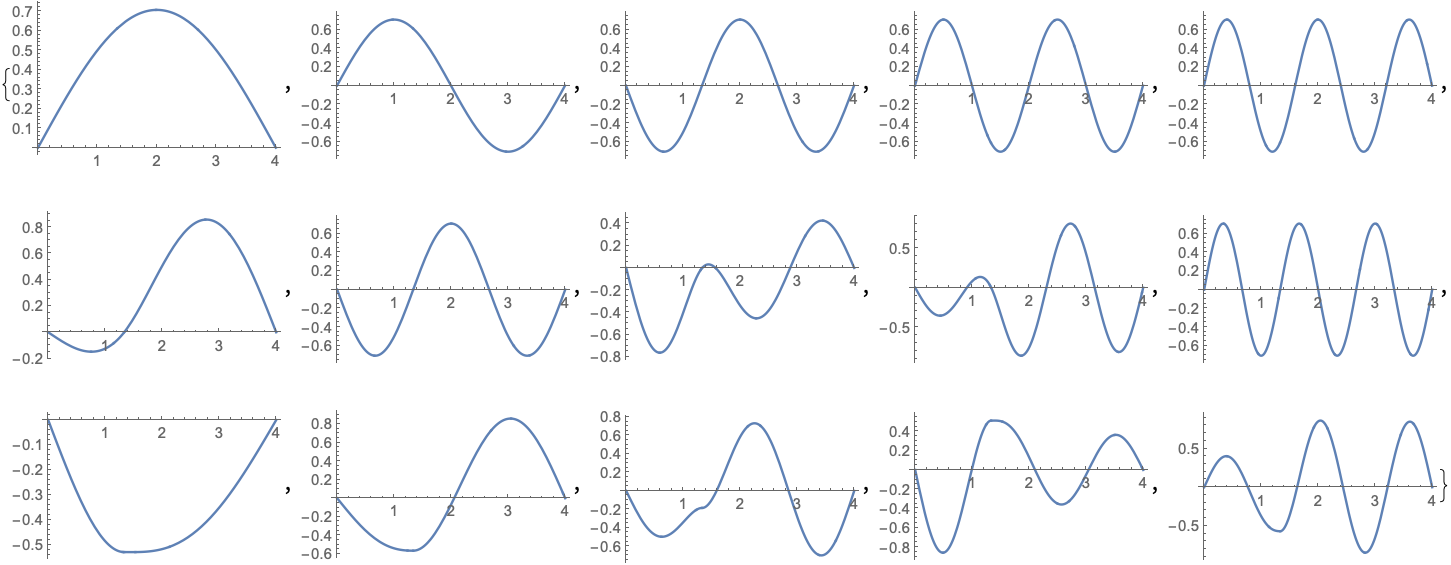
Table[Plot[modes[[i]], {x, 0, L}], {i, 1, Length[modes]}]

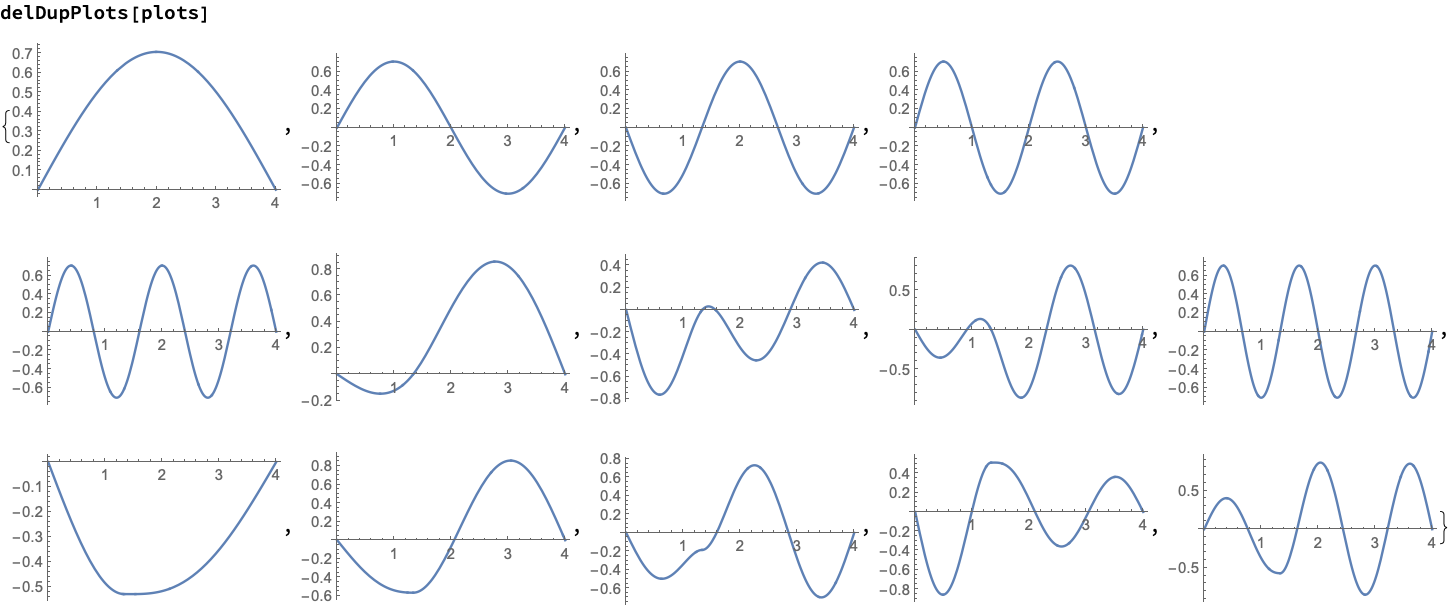
I have a list of plots in which some plots are same. I just wanted to create the list which contains unique plots. How to do this. I don't want to do it manually. because the size of the list is going to increase. Is there any build in function in Mathematica to handle this situation. I don't know how to carry out this task, there is no Logic in my mind right now to code and remove this from the list.

Answer 1

This is fairly slow.

Using your code

plots = Plot[#, {x, 0, L}] & /@ modes

plots[[3]] and plots[[7]] are visually the same

delDupPlots[plots_, threshold : _Integer?Positive : 100] := 
 Fold[Drop[#1, {#2}] &, plots, 
  Select[Table[{i, j, 
        Total@Flatten[
          ImageData@Binarize@ImageDifference[plots[[i]], plots[[j]]]]}, {i, 1,
         Length[plots] - 1}, {j, i + 1, Length[plots]}] // 
      Flatten[#, 1] &, #[[3]] < threshold &][[All, 2]] // ReverseSort]

delDupPlots eliminates plots[[7]]

delDupPlots[plots]