How to retrieve updated point coordinates that have been scaled or translated?

Question

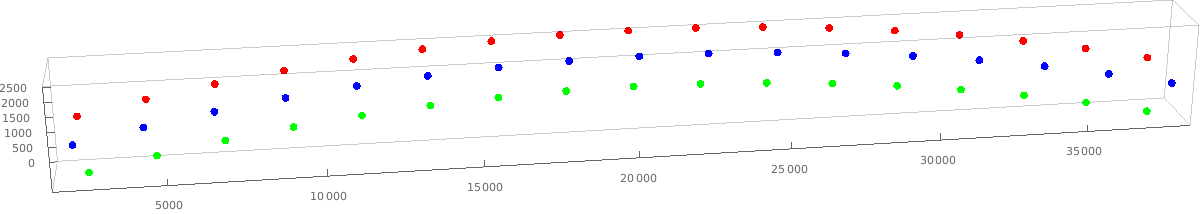
I'm sort of building off my previous question but the conditions of the points I'm trying to recover are different and therefore that solution is no longer applicable. Given said data, I'm trying to reconfigure the points that have been scaled and translated below so that I can do further geometric manipulation on them (such as to Transpose the 3 lists of points so as to end up with triplets that could be made into Polygon). However, I can't seem to directly interact with the updated point coordinates, it always reverts back to the original points derived by the arc.

 arc3D[{a_, b_, m_}, n_: 60, prim_: Line] := 
  Module[{\[Alpha], lab, axis, aarc, tm, alpha},
    lab = m + Norm[a - m]*Normalize[b - m];
    axis = (a - m)\[Cross](b - m);
    aarc = (VectorAngle[a - m, b - m]);
    tm = RotationMatrix[alpha, axis];
    prim@Table[m + tm.(a - m), {alpha, 0, aarc, aarc/n}]
    ]

coord={{0,0,0},{40000,0,0},{40000/2,0,40000*2}};
data=arc3D[coord,18,Line];

g1  = Graphics3D[
{Green,AbsolutePointSize[8],
 Scale[#,scaleScript[1200],coord[[3]]] &/@ Point /@ Rest@Drop[data[[1]],-1]}
];
g2 = Graphics3D[
{Red,AbsolutePointSize[8],
 Translate[#,{0,1200,0}]&/@ Point/@ Rest@Drop[data[[1]],-1]}
];
g3 = Graphics3D[
{Blue,AbsolutePointSize[8],
 Translate[#,{0,-1200,0}]&/@ Point/@ Rest@Drop[data[[1]],-1]}
];
Show[g1,g2,g3]

Answer 1

It turns out that this question is easier than the linked question as Normal works here to transform the coordinates but it doesn't in the linked case.

scaleScript[1200] = .9;
pts = N@Cases[#, Point[x_] :> x, Infinity] & /@ First[Normal@Show[g1, g2, g3]];
Show[g1, g2, g3, Graphics3D[{Opacity[.5], Polygon@Transpose[pts]}]]

Note: Documentation (Scale >> Properties and Relations and Translate >> Properties and Relations and GeometricTransformation >> Properties and Relations ) says:

• When possible, Normal will transform the coordinates explicitly.

but it is not at all clear what "When possible" means.