Mathematica works on the general case, not the specific, when it comes to
simplifications. Let try to find what is going on. Mathematica says that
\begin{align*}
I & =\int_{0}^{1}\sin\left( k\pi x\right) \sin\left( 2n\pi x\right) dx\\
& =\frac{1}{2\pi}\left( \frac{\sin\left( \left( k-2n\right) \pi\right)
}{k-2n}-\frac{\sin\left( \left( k+2n\right) \pi\right) }{k+2n}\right)
\end{align*}
Now, when you said that $k,n$ are integers, then $k-2n$ is also an integer, as
well as $k+2n$. Therefore the above becomes zero. Which agrees with what
Mathematica gives. Mathematica will not consider the special case here of what
happenes if $k=2n$, since this is a special case of $k$.
Now lets look at what happens when you give specific case when $k=2n$. Then the result above becomes
\begin{align*}
I & =\frac{1}{2\pi}\left( \frac{\sin\left( \left( 2n-2n\right)
\pi\right) }{2n-2n}-\frac{\sin\left( \left( 2n+2n\right) \pi\right)
}{2n+2n}\right) \\
& =\frac{1}{2\pi}\left( \frac{\sin\left( m\pi\right) }{m}-\frac{\sin\left(
4n\pi\right) }{4n}\right)
\end{align*}
Where $m=2n-2n$. (did not want to put zero, since need to take limit). Then
the above becomes
$$
I=\frac{1}{2\pi}\frac{\sin\left( m\pi\right) }{m}-\frac{1}{2\pi}\frac
{\sin\left( 4n\pi\right) }{4n}
$$
Since $n$ is integer, then the second term above is zero. (notice also, here there is special case, what if $n=0$? Then you'll get 1/2 also for the second term and the whole thing becomes zero, like case 1, But since $n=0$ is special case, it is not considered). Now the above becomes
$$
I=\frac{1}{2\pi}\frac{\sin\left( m\pi\right) }{m}
$$
But $\lim_{m\rightarrow0}\frac{\sin m\pi}{m}=\pi$, hence the above becomes
\begin{align*}
I & =\frac{1}{2\pi}\pi\\
& =\frac{1}{2}
\end{align*}
Which is what Mathematica gives.
ClearAll[n,k,x]
Assuming[ Element[k,Integers]&&Element[n,Integers],
Simplify[Integrate[Sin[k Pi x]*Sin[2*n Pi x],{x,0,1}]]]
(*0*)
Assuming[ Element[n, Integers] && k == 2 n,
Simplify[Integrate[Sin[k Pi x]*Sin[2*n Pi x], {x, 0, 1}]]]
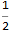

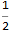
(2 n Cos[2 n \[Pi]] Sin[k \[Pi]] - k Cos[k \[Pi]] Sin[2 n \[Pi]])/( k^2 \[Pi] - 4 n^2 \[Pi]), which is either zero orIndeterminatefor integer variables. After repairing the=that should be==in Code 2, I get1/8 (4 - Sin[4 n \[Pi]]/(n \[Pi])),which is1/2for integern. $\endgroup$int1, and forLimit[int1, k -> 2 n, Assumptions -> k \[Element] Integers && n \[Element] Integers]the desired result1/2. $\endgroup$ClearAll[n, k, x]; Assuming[Element[k, Integers] && Element[n, Integers], Integrate[Sin[k Pi x]*Sin[2*n Pi x], {x, 0, 1}]]I get0as result. Maybe in Version 8.0 Assuming worked different than Assumptions. It seems in higher versions they operate the same way. $\endgroup$