Slowly Varing Color in the Listplot (by iteration, time or next data point)

Question

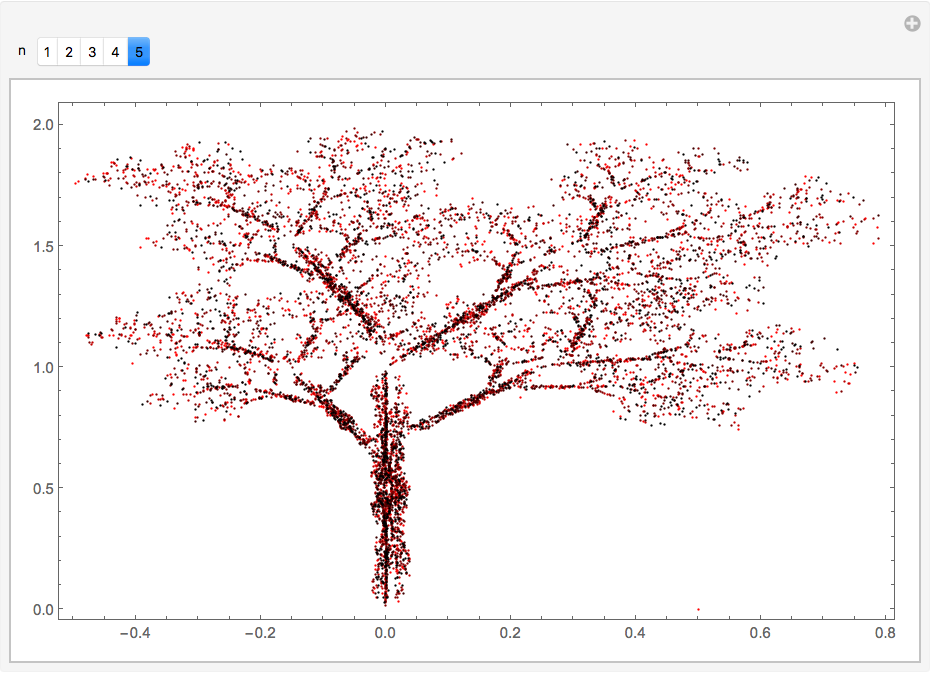
I am having little trouble with coloring the list plot. I want to vary the color slowly.

For example, if I have data

{0,1,2,3,5,7,8,9,7,5,2,1,3,5}

I want it to be

{Red -> slowly change ->Black}

to see how it change by the iteration. Which means that 0 will be red, 5 will be black, and in between number 1 2 3////5 2 1 3 will slowly change from darker red to light black.

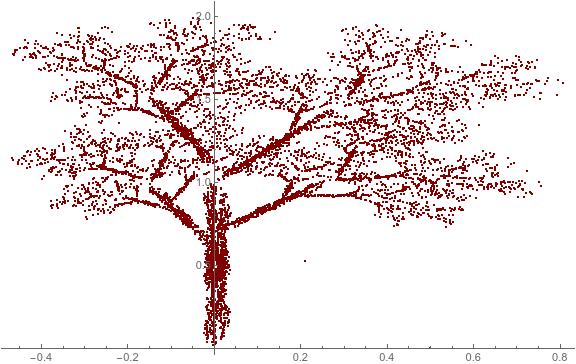
I have data using simple fractal pattern. I am just curious to see how the each iteration in fractal pattern moves. Maybe it might not show anything.

f[pt_] := Module[{r},
  r = RandomReal[{0, 1}];
  Which[0 <= r < 0.1, {0.05*pt[[1]], 0.5 pt[[2]]},
   0.1 <= r < 0.2, {0.05*pt[[1]], -0.5 pt[[2]] + 1},
   0.2 <= r < 0.4, {0.46*pt[[1]] - 0.15 pt[[2]], 
    0.39 pt[[1]] + 0.38 pt[[2]] + 0.6},
   0.4 <= r < 0.6, {0.47*pt[[1]] - 0.15 pt[[2]], 
    0.17 pt[[1]] + 0.42 pt[[2]] + 1.1},
   0.6 <= r < 
    0.8, {0.43*pt[[1]] + 0.28 pt[[2]], -0.25 pt[[1]] + 0.45 pt[[2]] + 
     1},
   0.8 <= r < 
    1, {0.42*pt[[1]] + 0.26 pt[[2]], -0.35 pt[[1]] + 0.31 pt[[2]] + 
     0.7}
   ]
  ]
ListPlot[NestList[f, {0.5, 0}, 10000], ImageSize -> Large, 
 PlotRange -> All, PlotStyle -> Hue[1, 1, 0.5]]

This will generate

I am trying to use ColorFunction, but I could not figure it out. Thank you for helping me.

Answer 1

f[pt_] := Module[{r}, r = RandomReal[{0, 1}];
  Which[0 <= r < 0.1, {0.05*pt[[1]], 0.5 pt[[2]]}, 
   0.1 <= r < 0.2, {0.05*pt[[1]], -0.5 pt[[2]] + 1}, 
   0.2 <= r < 0.4, {0.46*pt[[1]] - 0.15 pt[[2]], 
    0.39 pt[[1]] + 0.38 pt[[2]] + 0.6}, 
   0.4 <= r < 0.6, {0.47*pt[[1]] - 0.15 pt[[2]], 
    0.17 pt[[1]] + 0.42 pt[[2]] + 1.1}, 
   0.6 <= r < 
    0.8, {0.43*pt[[1]] + 0.28 pt[[2]], -0.25 pt[[1]] + 0.45 pt[[2]] + 1}, 
   0.8 <= r < 
    1, {0.42*pt[[1]] + 0.26 pt[[2]], -0.35 pt[[1]] + 0.31 pt[[2]] + 0.7}]]

SeedRandom[0];

Break the data into 5 subsets of 2000 data points.

data = NestList[f, {0.5, 0}, 9999] // Partition[#, 2000] &;

Manipulate[
 ListPlot[data[[1 ;; n]],
  ImageSize -> Large,
  PlotStyle -> (Blend[{Red, Black}, (# - 1)/4] & /@ Range[5]),
  Frame -> True, Axes -> False,
  PlotRange -> All],
 {{n, 5}, Range[5]}]