I have a list of data, and I interpolate it to a function. Then I need to do an integration with the interpolating function. But I found that the speed is unacceptably slow.
My data is here (a little long, but don't go away :) ):
data = {-0.00799443, -0.00581522, -0.00557107, -0.00543862, -0.00528042, \
-0.00508618, -0.00486091, -0.00461279, -0.00435009, -0.00408028, \
-0.0038098, -0.00354416, -0.00328805, -0.00304535, -0.00281906, \
-0.0026108, -0.00242045, -0.00224588, -0.00208332, -0.00192845, \
-0.00177801, -0.00163133, -0.00149092, -0.00136145, -0.0012474, \
-0.00115024, -0.00106716, -0.000992246, -0.000919878, -0.000848073, \
-0.000779184, -0.000717175, -0.000663667, -0.000616407, -0.00057173, \
-0.000528424, -0.000488614, -0.000454547, -0.000425288, -0.000397686, \
-0.000370268, -0.00034446, -0.000321488, -0.00030009, -0.000278782, \
-0.000258483, -0.000240725, -0.000224931, -0.000209972, -0.000196452, \
-0.000184918, -0.000174195, -0.000163592, -0.000153752, -0.000144418, \
-0.000134884, -0.000125771, -0.000117444, -0.000109436, -0.000102175, \
-0.0000959463, -0.0000902133, -0.000085125, -0.0000806452, \
-0.0000762082, -0.0000719591, -0.0000677566, -0.000063368, \
-0.0000591507, -0.0000549953, -0.0000510613, -0.0000475951, \
-0.0000444333, -0.0000417897, -0.0000394736, -0.0000373836, \
-0.0000354749, -0.0000334705, -0.0000314543, -0.0000292503, \
-0.0000269879, -0.0000247026, -0.0000224853, -0.0000204942, \
-0.0000187118, -0.0000172668, -0.0000160166, -0.0000149913, \
-0.0000139883, -0.0000129844, -0.000011827, -0.0000105289, \
-9.06132*10^-6, -7.50783*10^-6, -5.94092*10^-6, -4.46213*10^-6, \
-3.15097*10^-6, -2.0399*10^-6, -1.13236*10^-6, -3.57489*10^-7,
3.57489*10^-7, 1.13236*10^-6, 2.0399*10^-6, 3.15097*10^-6,
4.46213*10^-6, 5.94092*10^-6, 7.50783*10^-6,
9.06132*10^-6, 0.0000105289, 0.000011827, 0.0000129844, \
0.0000139883, 0.0000149913, 0.0000160166, 0.0000172668, 0.0000187118, \
0.0000204942, 0.0000224853, 0.0000247026, 0.0000269879, 0.0000292503, \
0.0000314543, 0.0000334705, 0.0000354749, 0.0000373836, 0.0000394736, \
0.0000417897, 0.0000444333, 0.0000475951, 0.0000510613, 0.0000549953, \
0.0000591507, 0.000063368, 0.0000677566, 0.0000719591, 0.0000762082, \
0.0000806452, 0.000085125, 0.0000902133, 0.0000959463, 0.000102175, \
0.000109436, 0.000117444, 0.000125771, 0.000134884, 0.000144418, \
0.000153752, 0.000163592, 0.000174195, 0.000184918, 0.000196452, \
0.000209972, 0.000224931, 0.000240725, 0.000258483, 0.000278782, \
0.00030009, 0.000321488, 0.00034446, 0.000370268, 0.000397686, \
0.000425288, 0.000454547, 0.000488614, 0.000528424, 0.00057173, \
0.000616407, 0.000663667, 0.000717175, 0.000779184, 0.000848073, \
0.000919878, 0.000992246, 0.00106716, 0.00115024, 0.0012474, \
0.00136145, 0.00149092, 0.00163133, 0.00177801, 0.00192845, \
0.00208332, 0.00224588, 0.00242045, 0.0026108, 0.00281906, \
0.00304535, 0.00328805, 0.00354416, 0.0038098, 0.00408028, \
0.00435009, 0.00461279, 0.00486091, 0.00508618, 0.00528042, \
0.00543862, 0.00557107, 0.00581522, 0.00799443}
Interpolate it:
f = Interpolation[data];
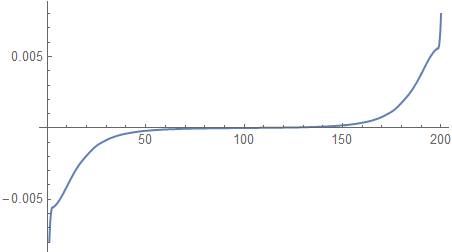
Plot[f[x], {x, 1, 200}, PlotRange -> All]
It looks like:
Now I define a function:
Clear[b];
b[x_, y_] := NIntegrate[
Cross[{0, f[s], 0}, {x - s, 0, y}][[{1, 3}]]/((x - s)^2 + y^2),
{s, 1, 200}
];
The integration is so slow!!
b[1., 2.] // AbsoluteTiming
(*{1.17772, {-0.00827965, -0.0104805}}*)
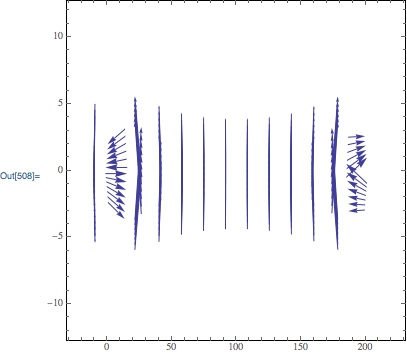
What I want to do is a vector plot:
VectorPlot[b[x, y], {x, -10, 210}, {y, -3, 3}]
But with this kind of slow integration, this is painful. Are there better ways to speed up the integration?