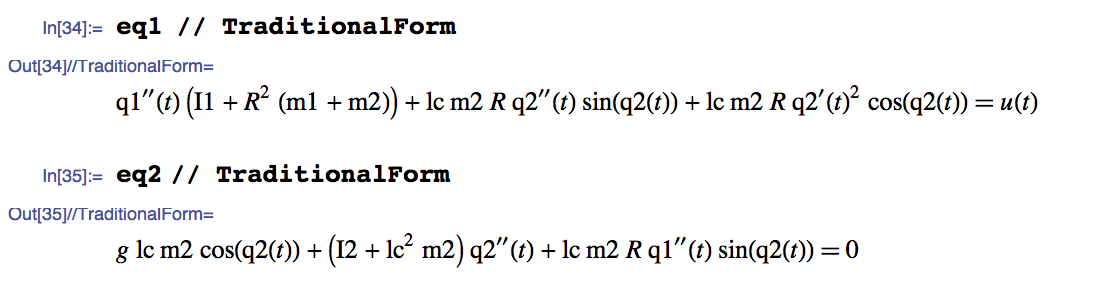Rearranging dynamic equations

Question

I'm deriving dynamic equations for a simple system, this is what I get:

How can I rearrange these 2 equations into the form like this:

My code is:

Subscript[x, 1] = -Subscript[q, 1][t] R; 
Subscript[y, 1] = 0; 
Subscript[x, 2] = 
  Subscript[x, 1] + Subscript[l, c] Cos[Subscript[q, 2][t]]; 
Subscript[y, 2] = Subscript[l, c] Sin[Subscript[q, 2][t]];
Subscript[v, 1] = Sqrt[
  D[Subscript[x, 1], t]^2 + D[Subscript[y, 1], t]^2]; 
Subscript[v, 2] = Sqrt[
  D[Subscript[x, 2], t]^2 + D[Subscript[y, 2], t]^2];
Subscript[w, 1] = D[Subscript[q, 1][t], t];
Subscript[w, 2] = D[Subscript[q, 2][t], t];
K = 1/2 (Subscript[II, 1] Subscript[w, 1]^2) + 
   1/2 (Subscript[II, 2] Subscript[w, 2]^2) + 
   1/2 (Subscript[m, 1] Subscript[v, 1]^2) + 
   1/2 (Subscript[m, 2] Subscript[v, 2]^2); 
P = g*Subscript[l, c]*Subscript[m, 2]*Sin[Subscript[q, 2][t]]; 
L = K - P; 
eq1 = Simplify[
   D[D[L, Subscript[q, 1]'[t]], t] - D[L, Subscript[q, 1][t]] ==  
    u[t]];
eq2 = Simplify[
   D[D[L, Subscript[q, 2]'[t]], t] - D[L, Subscript[q, 2][t]] == 0];

Thank you!

Answer 1

this should get you started:

{{d1, m12}, {m11, zero}} =  CoefficientList[ eq1[[2]], {q1''[t], q2''[t]}]

note Simplify decided to put u[t] on the left and the eq1[[2]] is picking the right hand side of the simplified equation. You'd probably be better off to work directly with your left side expression before building it into an equation.