Finding the period of an array of integers

Question

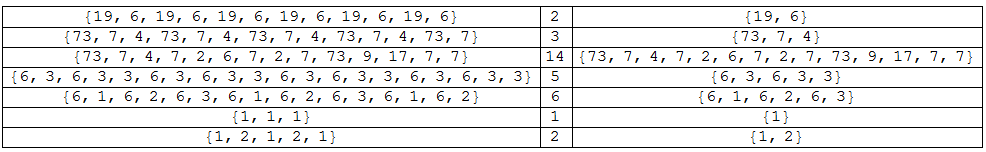
A simple array of integers is given. The problem is to detect if a pattern is repeatedly occurring in the array, and find the length of that pattern.

For example, for

{19, 6, 19, 6, 19, 6, 19, 6, 19, 6, 19, 6}

pattern {19, 6} should be detected and its length is 2.

For

{73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7}

pattern {73, 7, 4} should be detected and its length is 3. (at the end of the array there need not be the complete pattern, but the pattern should start at the beginning of the array)

For

{73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17, 7, 7}

the whole array is the pattern and its length is 14.

Related links

MATLAB function seqperiod()

SO question on cycle detection

Related question on this site

Wikipedia article on cycle detection

Answer 1

This uses partitioning, with padding if required, to make sublists.

f = Module[{b, c = 1},
    While[Length[b = Union@Partition[#, c, c, {1, 1}, Take[#, c]]] > 1, c++];
    {Length@First@b, First@b}] &;

Example

f@{73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7}

{3, {73, 7, 4}}

Answer 2

ClearAll[len]

len[{p__, p__ .., e___}] /; MatchQ[{p}, {e, __}] := Length[{p}]
len[p_] := Length[p]

len /@ lists
(* {2, 3, 14} *)

Answer 3

I won't bet my hand for this but seems to be ok:

ClearAll[return];
return[x : {0 ..., 1}, list_] := {#, list[[;; #]]} &[Length@x];
return[x_, y_] := {Length@y, y};

sqPeriod[list_] := return[FindLinearRecurrence[list], list]



sqPeriod /@ {
   {19, 6, 19, 6, 19, 6, 19, 6, 19, 6, 19, 6},
   {73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7},
   {73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17, 7, 7}
   } // Column

Answer 4

This answer only returns the period. If you want to extract the repeating substring, just use Take[list, period].

sequencePeriod = Compile[{{l, _Integer, 1}},
  With[{n = Length[l]},
   Catch[
    Do[
     If[
       Catch[
        Do[
         Do[
           If[l[[j]] != l[[k]], Throw[False]];,
           {k, i + j, n, i}
           ];,
         {j, i}
         ];
        Throw[True];
        ],
       Throw[i];
       ];,
     {i, Quotient[n, 2]}
     ];
    Throw[n];
    ]
   ]
  ]

For each trial period i, I go through the elements j of the trial list (1 through i) and make sure that each one is repeated in each subsequent copy of the list (k, offset from j by multiples of the period i).

This is the fastest solution so far:

I generated the lists for these tests with

Join @@ ConstantArray[RandomInteger[n, n], {n}]

• Pickett's solution is the most elegant, but seems to have exponential complexity.
• Kuba's has around quartic complexity due to the generality of FindLinearRecurrence.
• Chris has cubic performance, since he splits and compares the entire list at each trial period.
• My solution has quadratic performance, since we stop comparison as soon as we encounter a nonmatching element. It also has low memory usage, since I don't manipulate the array at all.

Answer 5

In versions 10+, there is FindTransientRepeat:

• FindTransientRepeat[list, n] returns a pair of lists {transient,repeat} where the elements of repeat occur successively at least n times at the end of list.
• FindTransientRepeat accepts an incomplete copy of the repeated sublist in the last position
• The minimum number of repetitions refers to complete repetitions

For the examples in the posted question

lists = {{19, 6, 19, 6, 19, 6, 19, 6, 19, 6, 19, 6}, 
         {73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7},
         {73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17, 7, 7}};

we get

FindTransientRepeat[#, 2]& /@ lists

{{{}, {19, 6}},
{{}, {73, 7, 4}},
{{73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17}, {7}}}

We can define a function that processes the output of FindTransientRepeat to get the results in desired form:

Clearall[repeatsF]
repeatsF = Module[{ftr = FindTransientRepeat[#, 2], lst = #},
 If[First @ ftr === {}, {Length @ Last @ ftr, Last @ ftr}, {Length @ lst, lst}]]&;

 repeatsF /@ lists

{{2, {19, 6}},
{3, {73, 7, 4}},
{14, {73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17, 7, 7}}}

Answer 6

Using the undocumented "Periodic" padding as the third argument of PadRight:

ClearAll[fpF, fpF2]
fpF = Block[{i = 1}, While[i < Length@# && 
      PadRight[#[[;; i]], Length@#, "Periodic"] != #, i++]; i] &;
fpF2 = Block[{i = 1}, While[i < Length@# && 
      PadRight[#[[;; i]], Length@#, "Periodic"] != #, i++]; {i, #[[;; i]]}] &;

Examples: Using tc from @ubpdqn's answer:

tc = {{19, 6, 19, 6, 19, 6, 19, 6, 19, 6, 19, 6}, 
      {73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7},
      {73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17, 7, 7}, 
      {6, 3, 6, 3, 3, 6, 3, 6, 3, 3, 6, 3, 6, 3, 3, 6, 3, 6, 3, 3},
      {6, 1, 6, 2, 6, 3, 6, 1, 6, 2, 6, 3, 6, 1, 6, 2},
      {1, 1, 1}, 
      {1, 2, 1, 2, 1}};

fpF /@ tc
(* {2, 3, 14, 5, 6, 1, 2} *)

{#, ## & @@ fpF2@#} & /@ tc // Grid[#, Dividers -> All] & 
(* or {#,fpF @ #, #[[;;fpF @ #]]}&/@tc //Grid *)

If a complete periodic pattern were sought, we could search for periods less than or equal to half the Length of the input list:

ClearAll[fpFa, fpFb]
fpFa = Block[{i = 1, n = Length@#},  While[i < 1 + n/2 && 
        PadRight[#[[;; i]], n, "Periodic"] != #, i++]; i = If[i < 1 + n/2, i, n]] &;
fpFb = Block[{i = 1, n = Length@#}, While[i < 1 + n/2 && 
        PadRight[#[[;; i]], n, "Periodic"] != #, i++]; i = If[i < 1 + n/2, i, n]; 
        {i, #[[;; i]]}] &;

Answer 7

FindRepeat meets your need perfectly.

FindRepeat[{19, 6, 19, 6, 19, 6, 19, 6, 19, 6, 19, 6}]

{19, 6}

FindRepeat[{73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7, 4, 73, 7}]

{73, 7, 4}

FindRepeat[{73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17, 7, 7}]

{73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17, 7, 7}

Answer 8

Late to party, and liking all answers but esp Chris Degnen:

per[u_] := Module[{j = 1, lg = Length@u},
  While[Total[
     Abs[Take[Join @@ ConstantArray[u[[;; j]], Ceiling[lg/j]], lg] - 
       u]] != 0, j++]; {j, u[[;; j]]}]

Some test cases:

tc={{19, 6, 19, 6, 19, 6, 19, 6, 19, 6, 19, 6}, {73, 7, 4, 73, 7, 4, 73, 
  7, 4, 73, 7, 4, 73, 7}, {73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 9, 17, 7, 
  7}, {6, 3, 6, 3, 3, 6, 3, 6, 3, 3, 6, 3, 6, 3, 3, 6, 3, 6, 3, 
  3}, {6, 1, 6, 2, 6, 3, 6, 1, 6, 2, 6, 3, 6, 1, 6, 2}, {1, 1, 1}, {1,
   2, 1, 2, 1}}

Testing: per/@tc

yields:

{{2, {19, 6}}, {3, {73, 7, 4}}, {14, {73, 7, 4, 7, 2, 6, 7, 2, 7, 73, 
   9, 17, 7, 7}}, {5, {6, 3, 6, 3, 3}}, {6, {6, 1, 6, 2, 6, 
   3}}, {1, {1}}, {2, {1, 2}}}