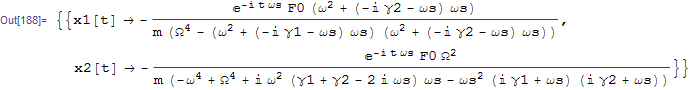Symbolically solve a system of coupled second order differential equations

Question

I have posted a similar question in another forum where the general consensus seems to suggest that it is not possible to symbolic solve a system of coupled second order differential equations with damping (dissipation) and driving forces.

However, I have found in many papers and books writing out analytical formula of the solutions to such coupled equations. So, it must be possible. I just do not know how.

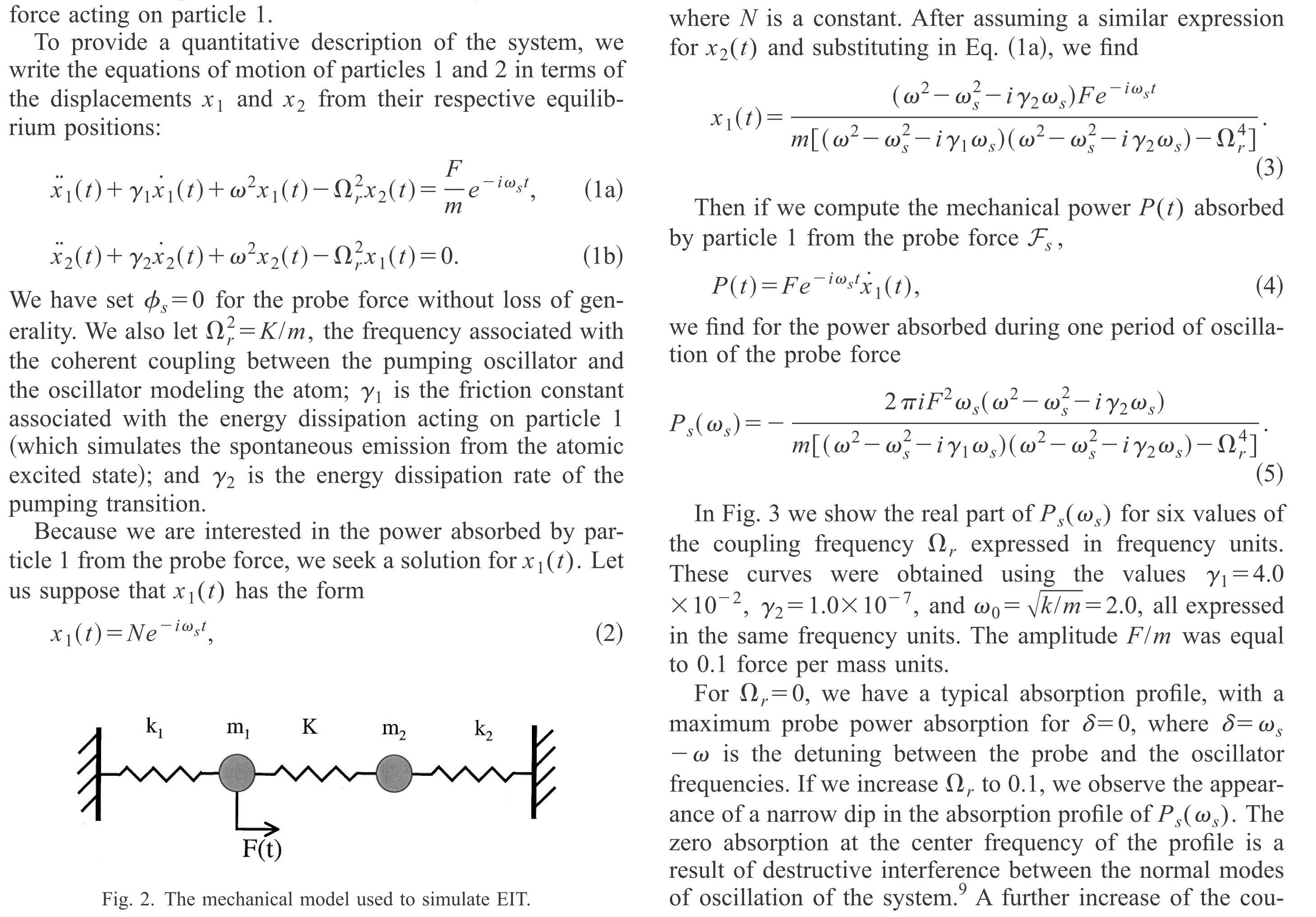
Attached is the system I am trying to solve. Although solving this sort of equation with two masses, no damping (dissipation) and with only one driving force is simple enough, even by hand, it is impossible to do the same for a system with two different damping constants and two driving forces. I have been trying for two weeks now, but could not figure out the solution.

Of course, I could get the numeric solution, but I could not get the same result obtained from Eq. 5. So, I was wondering if someone could help me how to solve this analytically. Any solution to this problem would be of interest.

I am completely new to Mathematica, so any example worksheets on this sort of equation would be gratefully appreciated!

Answer 1

How about

eqns = {x1''[t] +  γ1 x1'[t] + ω^2 x1[t] - Ω^2 x2[t] == F/m Exp[-I ωs t],
  x2''[t] +  γ2 x2'[t] + ω^2 x2[t] - Ω^2 x1[t] == 0};

Substituting a forced solution

eqns2 = eqns /.(rule= {
   x1 ->  Function[t, A1 Exp[-I ωs t]],
   x2 ->  Function[t, A2 Exp[-I ωs t]]})

and solving for the amplitude

 sol= Solve[eqns2, {A1, A2}] // Flatten // FullSimplify

(*
   {A1 -> (F (ω^2 + ωs (-ωs - I γ2)))/( m (-Ω^4 + (ω^2 + ωs(-ωs - I γ1))(ω^2 + ωs (-ωs-I γ2)))), 
    A2 -> (F Ω^2)/( m (-Ω^4 + (ω^2 + ωs (-ωs -  I γ1)) (ω^2 + ωs (-ωs - I γ2))))}
*)

If you want to get the power,

F Exp[I ωs t] x1'[t] /. rule /. sol // FullSimplify

(*
==> -(( I F^2 ωs  (-ω^2 + ωs (ωs + I γ2)))/( m (Ω^4 - (ω^2 + ωs (-ωs - 
          I γ1)) (ω^2 + ωs (-ωs - I γ2)))))
*)

Answer 2

Another way:

ClearAll[x1, x2, t, γ1, γ2, Ω, ω, ωs, x10, x20]
eq1 = Hold[D[x1[t], {t, 2}]] + γ1 Hold[D[x1[t], t]] + ω^2 x1[t] - Ω^2 x2[t] == 
      F0/m Exp[-I ωs t];
eq2 = Hold[D[x2[t], {t, 2}]] + γ2 Hold[D[x2[t], t]] + ω^2 x2[t] - Ω^2 x1[t] == 0;
eq11 = Release[eq1 /. {x1[t] ->  N1 Exp[-I ωs t], x2[t] ->  N2 Exp[-I ωs t]}];
eq22 = Release[eq2 /. {x1[t] ->  N1 Exp[-I ωs t], x2[t] -> N2 Exp[-I ωs t]}];
sub = {N1 Exp[-I ωs t] -> x1[t], N2 Exp[-I ωs t] -> x2[t]};
Simplify@Solve[{eq11 /. sub, eq22 /. sub}, {x1[t], x2[t]}]

Made it all Greek letters. Thanks to Halirutan's program and Simon letting me know about it.