If the equation of ellipse is:
$$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1,$$
then how can the major and minor axes values in the denominators be extracted from eq?
eq = x^2/4 + y^2/3 == 1;
a^2=4; a=2; b^2=3; b=sqrt(3)
If the equation of ellipse is:
$$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1,$$
then how can the major and minor axes values in the denominators be extracted from eq?
eq = x^2/4 + y^2/3 == 1;
a^2=4; a=2; b^2=3; b=sqrt(3)
Expressing the ellipse as eq == 0:
eq = x^2/4 + y^2/3 - 1;
The semi-major axes are calculated from the eigenvalues of the second derivatives of eq:
1/Sqrt[Eigenvalues[D[eq, {{x, y}, 2}]/2]] // NumericalSort
(* {Sqrt[3], 2} *)
This is more general than pattern-matching approaches and also works on rotated ellipses:
eq = x^2/4 + x y/7 + y^2/3 - 1;
1/Sqrt[Eigenvalues[D[eq, {{x, y}, 2}]/2]] // NumericalSort
(* {2 Sqrt[42/(49 + Sqrt[193])], 2 Sqrt[42/(49 - Sqrt[193])]} *)
eq1 = x^2/4 + y^2/3 == 1;
eq2 = x^2/a^2 + y^2/b^2 == 1;
Solve[Denominator[Flatten@(List @@@ List @@ eq1)] ==
Denominator[Flatten@(List @@@ List @@ eq2)],
Assumptions -> {a > 0 && b > 0}]
{{a -> 2, b -> Sqrt[3]}}
Another possibility is (*8 more left to do *)
ClearAll["Global`*"]
eq = x^2/4 + y^2/3 == 1;
normalized = First[eq] - Last[eq]
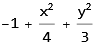
Coefficient[normalized, x^Exponent[normalized, x]];
Sqrt[Denominator[%]] (*a*)
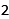
Coefficient[normalized, y^Exponent[normalized, y]];
Sqrt[Denominator[%]] (*b*)
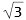
If the exponent is not meant to be the max but only 2 then do
ClearAll["Global`*"]
eq = x^2/4 + y^2/3 == 1;
normalized = First[eq] - Last[eq]
Sqrt[Denominator[Coefficient[normalized, x^2]]] (*a*)
Sqrt[Denominator[Coefficient[normalized, y^2]]] (*b*)

eq = x^2/4 + y^2/3 == 1;
TreeForm[eq]
To extract the coefficients:
(1/Sqrt[#]) &@(First /@ Level[eq, {2}])
{2, Sqrt[3]}
try https://resources.wolframcloud.com/FunctionRepository/resources/EllipseProperties
ass = ResourceFunction["EllipseProperties"][x^2/4 + y^2/3 == 1, {x, y}];
ass["SemimajorAxisLength"]
ass["SemiminorAxisLength"]
2
Sqrt[3]