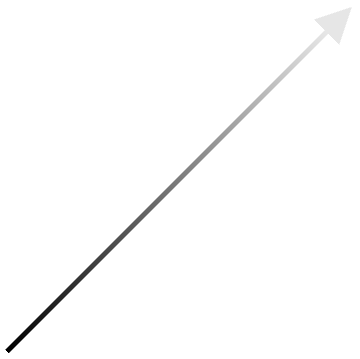
Graphics @
{Arrowheads[{{.1, 1, {Graphics[{Opacity[.1, Black],
Polygon[{{-1, 1/2}, {0, 0}, {-1, -1/2}}]}], 1}}}],
AbsoluteThickness[5],
Arrow[Line[{{2.5, 2.5}, {5, 5}},
VertexColors -> {Opacity[1, Black], Opacity[.1, Black]}]]}
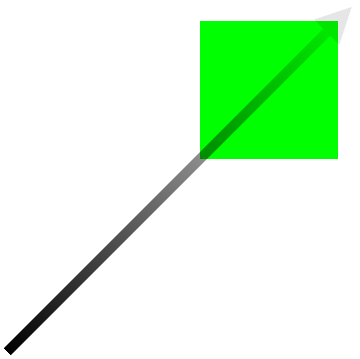
Graphics @
{Green, Rectangle[{3.9, 3.9}],
Arrowheads[{{.1, 1, {Graphics[{Opacity[.1, Black],
Polygon[{{-1, 1/2}, {0, 0}, {-1, -1/2}}]}], 1}}}],
AbsoluteThickness[10],
Arrow[Line[{{2.5, 2.5}, {5, 5}},
VertexColors -> {Opacity[1, Black], Opacity[.1, Black]}]]}
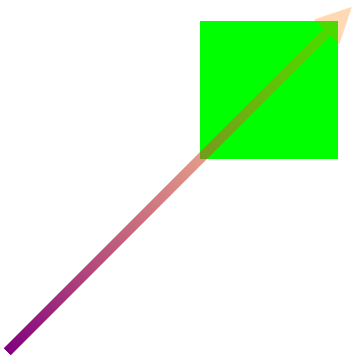
Replace Opacity[1, Black] with Opacity[1, Purple] and Opacity[.1, Black] with Opacity[.3, Orange] to get
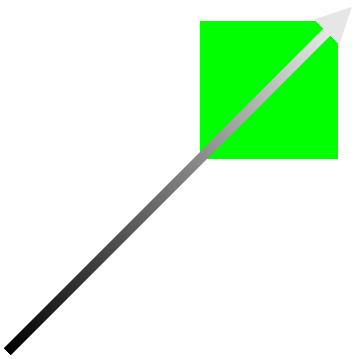
Alternatively, without Opacity:
Graphics @
{Green, Rectangle[{3.9, 3.9}],
Arrowheads[{{.1, 1, {Graphics[{GrayLevel[.9],
Polygon[{{-1, 1/2}, {0, 0}, {-1, -1/2}}]}], 1}}}],
AbsoluteThickness[10],
Arrow[Line[{{2.5, 2.5}, {5, 5}},
VertexColors -> {GrayLevel[0], GrayLevel[.9]}]]}
Replace GrayLevel[0] with Purple and GrayLevel[.9] with Lighter[Orange, .8] to get:
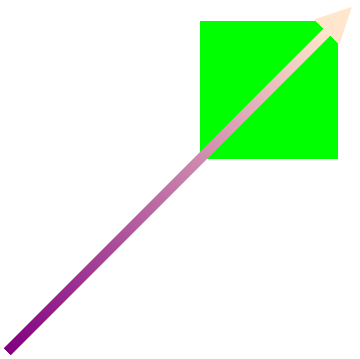
 arrow:
I failed to manage to gradually dims the arrow away to the arrow end.
arrow:
I failed to manage to gradually dims the arrow away to the arrow end.




