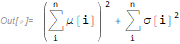Expectation of product of independent random variables (using Expectation function)

Question

I'm trying with no luck to get Expectation to calculate the expectation of expressions containing sums of products of independent random variables.

For instance, take $\mathbb{E}[\sum_{i=1}^n\sum_{j=1}^n X_iX_j]$, where $(X_1,\ldots,X_n)$ are mutually independent with $X_i \sim \mathcal{N}(a_i,b_i) $. Can Expectation perform this calculation?

EDIT

Could get the expectation of a product right by using Piecewise:

f[i_, j_] := 
 Piecewise[{{Expectation[
     x[i] x[j], {x[j] \[Distributed] 
       NormalDistribution[\[Mu][j], \[Sigma][j]], 
      x[i] \[Distributed] NormalDistribution[\[Mu][i], \[Sigma][i]]}],
     i != j}, {Expectation[x[i]^2, 
     x[i] \[Distributed] NormalDistribution[\[Mu][i], \[Sigma][i]]], 
    i == j}}]

But still couldn't obtain $\sum_{i,j}f_{i,j}$.

Answer 1

I'm pretty sure this doesn't answer the question the way you want (and I would want also) but using Expectation works if you specify n:

n = 5;
Expectation[Sum[x[i] x[j], {i, n}, {j, n}], 
  Table[x[i] \[Distributed] NormalDistribution[μ[i], σ[i]], {i, n}]] // FullSimplify
(* (μ[1] + μ[2] + μ[3] + μ[4] + μ[5])^2 + σ[1]^2 + σ[2]^2 + σ[3]^2 + σ[4]^2 + σ[5]^2 *)

From that results one can see the general form:

n =.;
Sum[\[Mu][i], {i, n}]^2 + Sum[\[Sigma][i]^2, {i, n}]

Answer 2

Same setup as in the answer by @JimB (fixed n) - just for fun.

 proc = ItoProcess[{
   \[DifferentialD]x1[t] == \[Sigma]1 \[DifferentialD]w1[t], 
   \[DifferentialD]x2[t] == \[Sigma]2 \[DifferentialD]w2[t], 
   \[DifferentialD]x3[t] == \[Sigma]3 \[DifferentialD]w3[t]}, 
    (x1[t] + x2[t] + x3[t])^2, {{x1, x2, x3}, {\[Mu]1, \[Mu]2, \[Mu]3}}, {t, 0}, 
    {w1 \[Distributed] WienerProcess[], 
     w2 \[Distributed] WienerProcess[],
     w3 \[Distributed] WienerProcess[]}];

Mean[proc[1]]
(* (\[Mu]1+\[Mu]2+\[Mu]3)^2+\[Sigma]1^2+\[Sigma]2^2+\[Sigma]3^2 *)