When I'm trying to calculate the value of DP, it subtracts the PCurrent matrix from each element of PGoal. What am I doing wrong?
OP4 = MatrixForm[{Cos[
T1] (L1 + L2 Cos[T2] + L3 Cos[T2 + T3]), (L1 + L2 Cos[T2] +
L3 Cos[T2 + T3]) Sin[T1], L2 Sin[T2] + L3 Sin[T2 + T3]}];
OP4 = OP4 /. {L1 -> 0.3, L2 -> 0.3, L3 -> 0.15}
Print["OP4=", MatrixForm[OP4]];
OJTrans =
MatrixForm[{{(-(L1 + L2 Cos[T2] + L3 Cos[T2 + T3])) Sin[T1],
Cos[T1] ((-L2) Sin[T2] - L3 Sin[T2 + T3]), ((-L3) Cos[T1]) Sin[
T2 + T3]}, {Cos[T1] (L1 + L2 Cos[T2] + L3 Cos[T2 + T3]),
Sin[T1] ((-L2) Sin[T2] - L3 Sin[T2 + T3]), ((-L3) Sin[T1]) Sin[
T2 + T3]}, {0, L2 Cos[T2] + L3 Cos[T2 + T3], L3 Cos[T2 + T3]}}];
OJTrans = OJTrans /. {L1 -> 0.3, L2 -> 0.3, L3 -> 0.15}
ThetaInitial = {{0}, {Pi/4}, {Pi/2}};
PGoal = {{0.35}, {0.05}, {0.35}};
Print["PGO=", MatrixForm[PGoal]];
ThetaEstimate = ThetaInitial;
For[i = 1, i <= 5, i++,
Print["IterationNumber", i];
PCurrent =
OP4 /. {T1 -> ThetaEstimate[[1, 1]], T2 -> ThetaEstimate[[2, 1]],
T3 -> ThetaEstimate[[3, 1]]};
Print["PCurrent=", MatrixForm[PCurrent]];
DP = PGoal - PCurrent;
Print["DP=", MatrixForm[DP]];
];
This is what i'm getting
SequenceForm["DP=",
MatrixForm[{{
0.35 - MatrixForm[{0.4060660171779821, 0., 0.3181980515339463}]}, {
0.05 - MatrixForm[{0.4060660171779821, 0., 0.3181980515339463}]}, {
0.35 - MatrixForm[{0.4060660171779821, 0., 0.3181980515339463}]}}]]


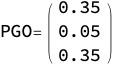

MatrixForm: See mathematica.stackexchange.com/questions/3098/… -- It would be easier to generate interest in other folks to investigate your problem with "a minimal working code example of your problem or your efforts". $\endgroup$