Suppose the oriented surface is described as, the outside of an upper hemisphere $S:x^2+y^2+z^2=1$ inside the cylinder $x^2-x+y^2=0$
The vector field is : ${\vec F}=<x^2,y^2,z^2>$
How to calculate the surface integral of the vector field: $$\iint\limits_{S^+} \vec F\cdot \vec n {\rm d}S $$
Is it the same thing to:
$$\iint\limits_{S^+}x^2{\rm d}y{\rm d}z+y^2{\rm d}x{\rm d}z+z^2{\rm d}x{\rm d}y$$
There is another post here with an answer by@MichaelE2 for the cases when the surface is easily described in parametric form. How to handle this case?

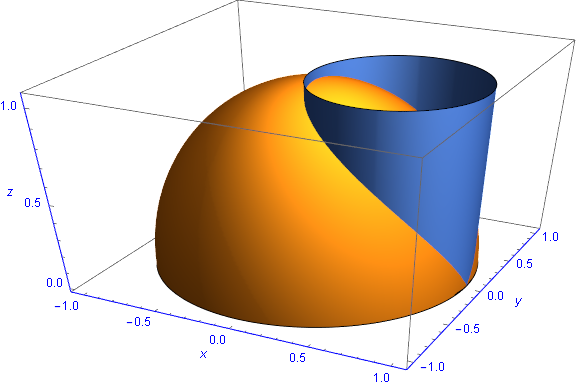
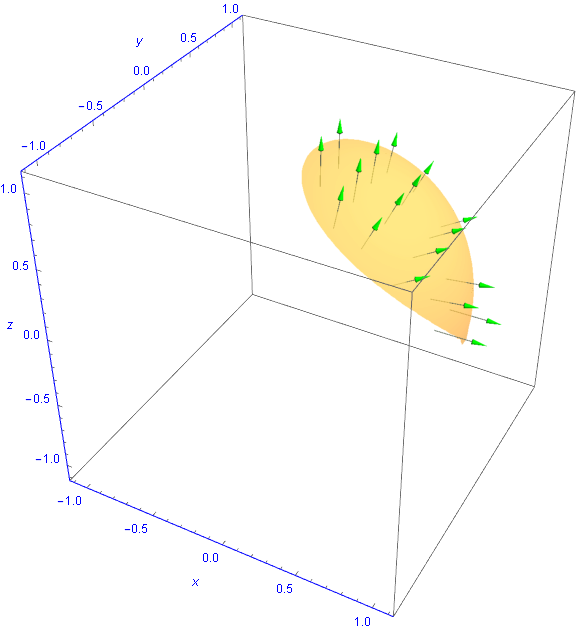


{x, y, Sqrt[1 - x^2 - y^2]}is one parameterization of the upper hemisphere. There's another in spherical coordinates, and so forth. $\endgroup$S = {{x, y, z} -> {Cos[u] v/2 +1/2, Sin[u] v/2, Sqrt[ 1 - (Cos[u] v/2 +1/2)^2 - (Sin[u] v/2)^2]}, {u, 0, 2 Pi}, {v, 0, 1}}; F = {x^2, y^2, z^2};$\endgroup$