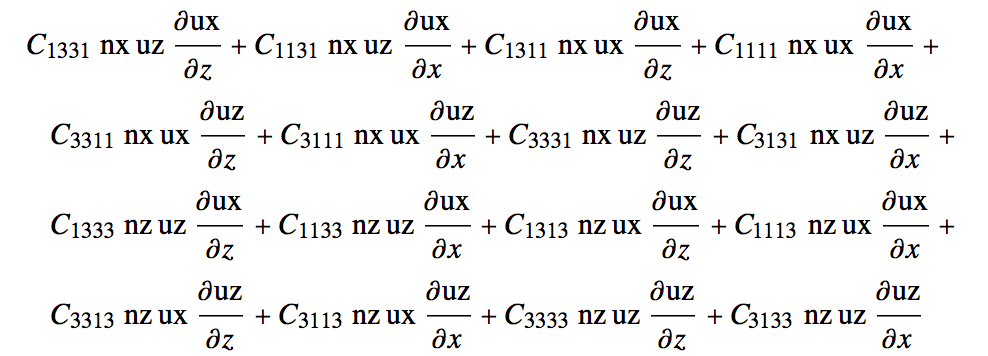Einstein summation and traditional form of partial derivative of implicit function

Question

I am quite new in Mathematica (1 week new) and after many trials I did not manage to make my partial derivatives to look traditional way.

There are few topics such as How to make traditional output for derivatives and Customizing display of partial differential equations answering this question, but in my case all proposed methods does not give desirable result.

Want this: $\frac{\partial u_x}{\partial x} + \frac{\partial u_x}{\partial z} + \frac{\partial u_z}{\partial x} + \frac{\partial u_z}{\partial z}$

Have this: $u_x^{(0,0,1)}(x,0,z) + ...$ or $\frac{\partial uv[[1]]} {\partial xz[[1]]}+...$

I would like to expand equation $n_l u_k C_{ijkl} u_{i,j}$. For this purpose I am doing sum over all 4 $ijkl$ indices and result looks as I want but partial derivatives

Code that I am using:

nv = {nx, 0, nz};
uv = {ux[x, 0, z], 0, uz[x, 0, z]};
xz = {x, 0, z};
CC = Array[C_{ToExpression@StringJoin@IntegerString@ {#1, #2, #3, #4}} &, {3, 3, 3, 3}];

(* _{ToExpression@StringJoin@IntegerString@ {#1, #2, #3, #4}} stands for a subscript of C *)

ux /: Format[ux[___]] := ux;
uz /: Format[uz[___]] := uz;

TraditionalForm[Sum[Part[nv, l] Part[uv, k] Part[CC, i, j, k, l] D[Part[uv, i], Part[xz, j]], {i, {1, 3}}, {j, {1, 3}}, {k, {1, 3}}, {l, {1, 3}}]]

If you have any ideas I am grateful in advance

Thanks!

Answer 1

I've edited my answer in the linked thread so that it can now be used without modification. Previously, you had to define the functions uv that you wish to differentiate in a more general way, replacing the explicit 0 in their argument with y. The reason is that my earlier answer assumed differentiations are performed on the given function, not on the new function obtained by setting one function argument equal to a constant.

However, now it works with the unchanged definitions in the question (except that I corrected a formatting error in your CC):

nv = {nx, 0, nz};
uv = {ux[x, 0, z], 0, uz[x, 0, z]};
xz = {x, 0, z};
CC = Array[
   Subscript[C, 
     ToExpression@StringJoin@IntegerString@{#1, #2, #3, #4}] &, {3, 3,
     3, 3}];

ux /: Format[ux[___]] := ux;
uz /: Format[uz[___]] := uz;

TraditionalForm[
 Sum[Part[nv, l] Part[uv, k] Part[CC, i, j, k, l] D[Part[uv, i], 
    Part[xz, j]], {i, {1, 3}}, {j, {1, 3}}, {k, {1, 3}}, {l, {1, 3}}]]