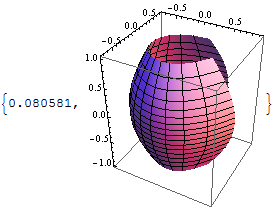
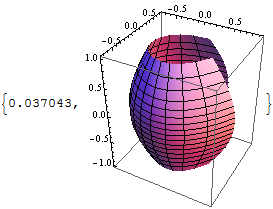
In my CAGD package, I implemented a CAGDBSplineFunction[] like built-in BSplineFunction[]. Here is a performance comparison:
pts = Table[{ Cos[2 Pi u/6] Cos[v], Sin[2 Pi u/6] Cos[v], v}, {u, 6}, {v, -1, 1, 1/2}];
f = BSplineFunction[pts]
g = CAGDBSplineFunction[pts]
ParametricPlot3D[f[u, v], {u, 0, 1}, {v, 0, 1}] // AbsoluteTiming
ParametricPlot3D[g[u, v], {u, 0, 1}, {v, 0, 1}] // AbsoluteTiming
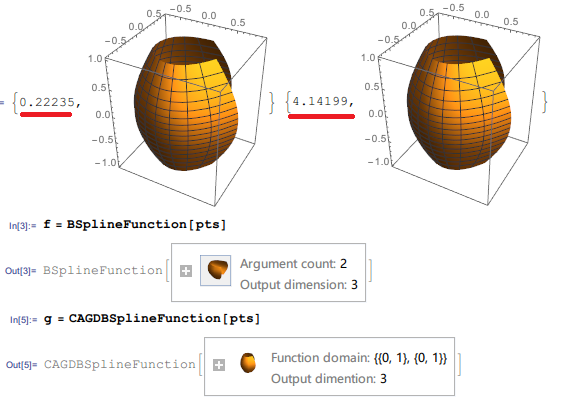
Obviously, my CAGDBSplineFunction[] is far slower(about 20X) than the built-in BSplineFunction[]
The CAGDBSplineFunction[] mainly uses an auxiliary function functionalNonzeroBasis[] to compute the coordinate of a parameter pair $(u,v)$.
To improve the performance, I refactor the functionalNonzeroBasis[] to compiledNonzeroBasis[]
searchSpan[{deg_, knots_}, u_] :=
With[{un = knots[[-(deg + 1)]]},
If[u == un,
Position[knots, un][[1, 1]] - 2,
Ordering[UnitStep[u - knots], 1][[1]] - 2
]
]
coeff[u_, U_, i_, p_] :=
If[U[[i + p + 1]] != U[[i + 1]],
(u - U[[i + 1]])/(U[[i + p + 1]] - U[[i + 1]]), 0]
compiledNonzeroBasis=
ReleaseHold[
Hold@Compile[{{i, _Integer}, {p, _Integer}, {u, _Real, 1}, {u0, _Real}},
Module[
{lst = Table[0., {p + 2}, {p + 1}], cc = i - 2},
lst[[2, 1]] = 1.0;
Do[
lst[[m + n - cc, n + 1]] =
coeff[u0, u, m, n] lst[[m + n - 1 - cc, n]] + (1 -
coeff[u0, u, m + 1, n]) lst[[m + n - cc, n]],
{n, 1, p}, {m, i - n, i}
];
lst // Transpose // Last // Rest
], CompilationTarget -> "C", RuntimeOptions -> "Speed"
] /. DownValues[coeff]
]
compiledBSplineSurf[
ctrlnets_, {{deg1_, knots1_}, {deg2_, knots2_}},
u_?NumericQ, v_?NumericQ] :=
Module[{i, j, validnets, row, col},
i = searchSpan[{deg1, knots1}, u];
j = searchSpan[{deg2, knots2}, v];
validnets =
Take[ctrlnets, {i - deg1 + 1, i + 1}, {j - deg2 + 1, j + 1}];
row = compiledNonzeroBasis[i, deg1, knots1, u];
col = compiledNonzeroBasis[j, deg2, knots2, v];
row.Transpose[validnets, {1, 3, 2}].col
]
Test
(*knot vector*)
k1 = {0., 0., 0., 0., 0.333333, 0.666667, 1., 1., 1., 1.};
k2 = {0., 0., 0., 0., 0.5, 1., 1., 1., 1.};
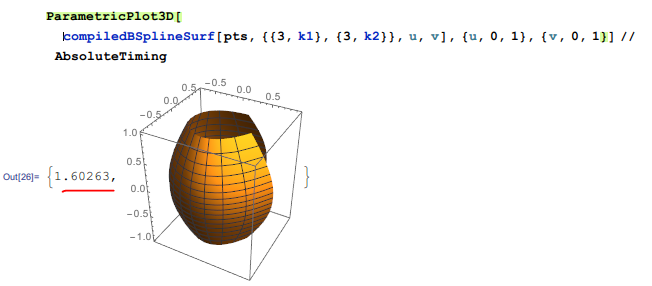
ParametricPlot3D[
compiledBSplineSurf[pts, {{3, k1}, {3, k2}}, u, v],
{u, 0, 1}, {v, 0, 1}] // AbsoluteTiming
This time the performance improves about 2X
Another trial is trying Compile`GetElement
With[{get = Compile`GetElement},
basisCoeff[deg_, U_, i_, u_] :=
If[U[[i + deg + 1]] != U[[i + 1]],
(u - get[U, i + 1])/(get[U, i + deg + 1] - get[U, i + 1]),
(*case for ui=uj*)
0.0
];
optimizedNonzeroBasis =
ReleaseHold[
Hold@Compile[{{i, _Integer}, {deg, _Integer}, {knots, _Real, 1}, {u0, _Real}},
Module[{lst = Table[0., {deg + 2}, {deg + 1}], c = i - 2},
lst[[2, 1]] = 1.0;
Do[
With[{mn = m + n},
lst[[mn - c, n + 1]] =
basisCoeff[n, knots, m, u0] get[lst, mn - c - 1, n] +
(1 - basisCoeff[n, knots, m + 1, u0]) get[lst,
mn - c, n]
],
{n, 1, deg}, {m, i - n, i}
];
Rest@Last[Transpose[lst]
]
], CompilationTarget -> "C", RuntimeOptions -> "Speed"
] /. DownValues[basisCoeff]]
]
ParametricPlot3D[
optimizedBSplineSurf[pts, {{3, k1}, {3, k2}}, u, v],
{u, 0, 1}, {v, 0, 1}] // AbsoluteTiming
So I would like to know:
- Is it possible to further improve/boost the performance of
compiledBSplineSurf[]?
Update:
For the optimizedBSplineSurf[], just replace compiledNonzeroBasis[] with optimizedNonzeroBasis[]