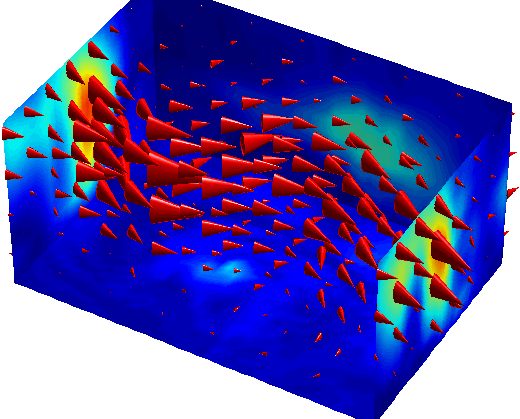
Does Mathematica have a function, similar to the one called coneplot in MATLAB? Given some spatial coordinates $x, y, z$ and velocity components $v_x,v_y,v_z$, it is able to produce the following graphs:
When I plot my data with ListVectorPlot3D, I don't get any arrows shown. Here is a sample data, in the form $((x,y,z),(v_x,v_y,v_z))$:
data={{{0., 0.847912, 9.48902}, {0., 0., 0.}}, {{0.00773322, -0.0110065,
9.09927}, {0., 0., 0.}}, {{-1.00008, -0.0623481, 9.49984}, {0., 0.,
0.}}, {{0., 0.847912, 10.8969}, {0., 0., 0.}}, {{0., 0.847912,
12.5007}, {0., 0., 0.}}, {{0., 0.847912, 14.1046}, {0., 0.,
0.}}, {{0., 0.847912, 15.5729}, {0., 0., 0.}}, {{0., 0.847912,
16.6107}, {0., 0., 0.}}, {{0., 0.847912, 17.3334}, {0., 0.,
0.}}, {{0., 0.847912, 18.0983}, {0., 0., 0.}}, {{0., 0.847912,
18.8639}, {0., 0., 0.}}, {{0., 0.847912, 19.9409}, {0., 0.,
0.}}, {{0., 0.847912, 21.3189}, {0., 0., 0.}}, {{0., 0.847912,
22.822}, {0., 0., 0.}}, {{0., 0.847912, 24.6036}, {0., 0.,
0.}}, {{0., 0.847912, 26.2343}, {0., 0.,
0.}}, {{-1.08457, -0.0217237, 10.6779}, {0., 0.,
0.}}, {{-1.11085, -0.0731095, 12.19}, {0., 0.,
0.}}, {{-1.1131, -0.0651947, 13.7893}, {0., 0.,
0.}}, {{-1.10496, -0.0720509, 15.108}, {0., 0.,
0.}}, {{-1.12255, -0.0365039, 16.0888}, {0., 0.,
0.}}, {{-1.12699, -0.00227834, 16.8818}, {0., 0., 0.}}, {{-1.15057,
0.0126093, 17.6497}, {0., 0., 0.}}, {{-1.13931, -0.015588,
18.4211}, {0., 0., 0.}}, {{-1.03192, -0.0742988, 19.1016}, {0., 0.,
0.}}, {{-1.24186, -0.0317904, 19.9972}, {0., 0.,
0.}}, {{-1.14942, -0.0360112, 20.9677}, {0., 0.,
0.}}, {{-1.09896, -0.0167395, 21.9511}, {0., 0.,
0.}}, {{-1.117, -0.0452791, 23.2337}, {0., 0.,
0.}}, {{-1.1522, -0.056303, 24.8936}, {0., 0.,
0.}}, {{-1.09607, -0.110654, 26.195}, {0., 0.,
0.}}, {{0.103537, -0.0647147, 26.6637}, {0., 0., 0.}}, {{0.,
0.847912, 41.3051}, {0., 0., 0.}}, {{-0.012139, -0.0295375,
40.9221}, {0., 0., 0.}}, {{-0.857944, -0.0671514, 41.3642}, {0.,
0., 0.}}, {{0., 0.847912, 42.4678}, {0., 4.67035*10^-7, 0.}}, {{0.,
0.847912, 43.5378}, {0., 0., 0.}}, {{-0.979967, -0.033538,
42.2371}, {0., 0., 0.}}, {{-1.14769, -0.0351505, 43.0033}, {0., 0.,
0.}}, {{-0.979351, -0.0342237, 43.8374}, {0., 0.,
0.}}, {{-1.23767, -0.0499179, 44.5971}, {0., 0.,
0.}}, {{-1.13972, -0.0000850668, 45.3128}, {0., 0.,
0.}}, {{-1.12545, -0.00826265, 46.0778}, {0., 0.,
0.}}, {{-1.1223, -0.0359113, 46.8929}, {0., 0.,
0.}}, {{-1.12465, -0.055138, 47.8623}, {0., 0.,
0.}}, {{-1.09846, -0.0144483, 49.0776}, {0., 0.,
0.}}, {{-1.10899, -0.0625359, 50.4507}, {0., 0.,
0.}}, {{-1.14579, -0.108309, 52.0417}, {0., 0.,
0.}}, {{-1.12848, -0.01126, 54.0108}, {0., 0.,
0.}}, {{-1.11299, -0.0970175, 55.2159}, {0., 0., 0.}}, {{0.,
0.847912, 44.6206}, {0., 0., 0.}}, {{0., 0.847912, 45.3961}, {0.,
0., 0.}}, {{0., 0.847912, 46.2075}, {0., 0., 0.}}, {{0., 0.847912,
47.3219}, {0., 0., 0.}}, {{0., 0.847912, 48.6172}, {0., 0.,
0.}}, {{0., 0.847912, 50.0586}, {0., 0., 0.}}, {{0., 0.847912,
51.6502}, {0., 0., 0.}}, {{0., 0.847912, 53.7857}, {0., 0.,
0.}}, {{0., 0.847912, 55.2595}, {0., 0.,
0.}}, {{0.119852, -0.0804816, 55.6719}, {0., 0.,
0.}}, {{1.38231, -0.0646805, 9.63683}, {0., 0.,
0.}}, {{3.08676, -0.0356642, 10.2185}, {0., 0., 0.}}, {{3.98277,
0.0329428, 10.6312}, {0., 0., 0.}}, {{1.64587, 0.847912,
10.1128}, {0., 0., 0.}}, {{2.94532, 0.847912, 10.6054}, {0., 0.,
0.}}, {{3.0844, 0.847912, 26.2343}, {0., 0., 0.}}, {{6.37314,
0.847912, 26.2343}, {0., 0., 0.}}, {{8.83598, 0.847912,
26.2343}, {0., 0., 0.}}, {{2.68672, -0.0254293, 26.6613}, {0., 0.,
0.}}, {{5.13989, -0.0481681, 26.6588}, {0., 0.,
0.}}, {{7.07074, -0.0263853, 26.6521}, {0., 0., 0.}}, {{8.87196,
0.0308362, 26.6941}, {0., 0., 0.}}, {{0.908045, -0.0256653,
41.2951}, {0., 0., 0.}}, {{0.90812, -0.0305627, 42.1266}, {0., 0.,
0.}}, {{1.09331, -0.0723344, 42.9721}, {0., 0.,
0.}}, {{1.03446, -0.06788, 43.5403}, {0., 0.,
0.}}, {{1.9443, -0.0212229, 43.8278}, {0., 0., 0.}}, {{2.52323,
0.00655421, 44.1426}, {0., 0., 0.}}, {{1.47266, 0.847912,
44.0959}, {0., 0., 0.}}, {{1.47266, 0.847912, 44.6541}, {0., 0.,
0.}}, {{3.48462, 0.847912, 55.2595}, {0., 0., 0.}}, {{9.45979,
0.847912, 55.2595}, {0., 0., 0.}}, {{15.9244, 0.847912,
55.2595}, {0., 0., 0.}}, {{20.8183, 0.847912, 55.2595}, {0., 0.,
0.}}, {{24.5798, 0.847912, 55.2595}, {0., 0., 0.}}, {{27.9807,
0.847912, 55.2595}, {0., 0., 0.}}, {{4.19646, -0.079758,
55.6822}, {0., 0., 0.}}, {{9.8436, -0.111618, 55.6824}, {0., 0.,
0.}}, {{17.6324, -0.0328765, 55.6789}, {0., 0.,
0.}}, {{23.6589, -0.044735, 55.6773}, {0., 0.,
0.}}, {{27.8005, -0.0722351, 55.6708}, {0., 0., 0.}}, {{2.94532,
0.847912, 11.1635}, {0., 0., 0.}}, {{2.62106, -0.0129568,
44.6594}, {0., 0., 0.}}, {{3.34577, -0.0199615, 44.9502}, {0., 0.,
0.}}, {{3.9942, 0.0362858, 45.2367}, {0., 0., 0.}}, {{2.27968,
0.847912, 44.96}, {0., 0., 0.}}, {{2.94532, 0.847912,
45.2124}, {0., 0., 0.}}, {{2.94532, 0.847912, 45.7705}, {0., 0.,
0.}}, {{4.30519, -0.0509312, 11.19}, {0., 0., 0.}}, {{5.04605,
0.847912, 11.9598}, {0., 0., 0.}}}

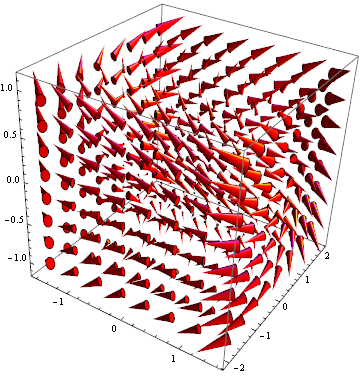
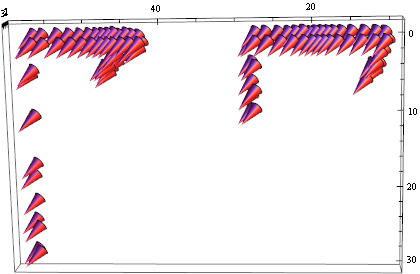
VectorPlot3DorListVectorPlot3Dwith specyficVectorStylewill give you cones. About the box: something like DensityPlot3D $\endgroup$Last /@ Last /@ datais all zeros? $\endgroup$VectorColorFunction->Huewhat you are after? $\endgroup$