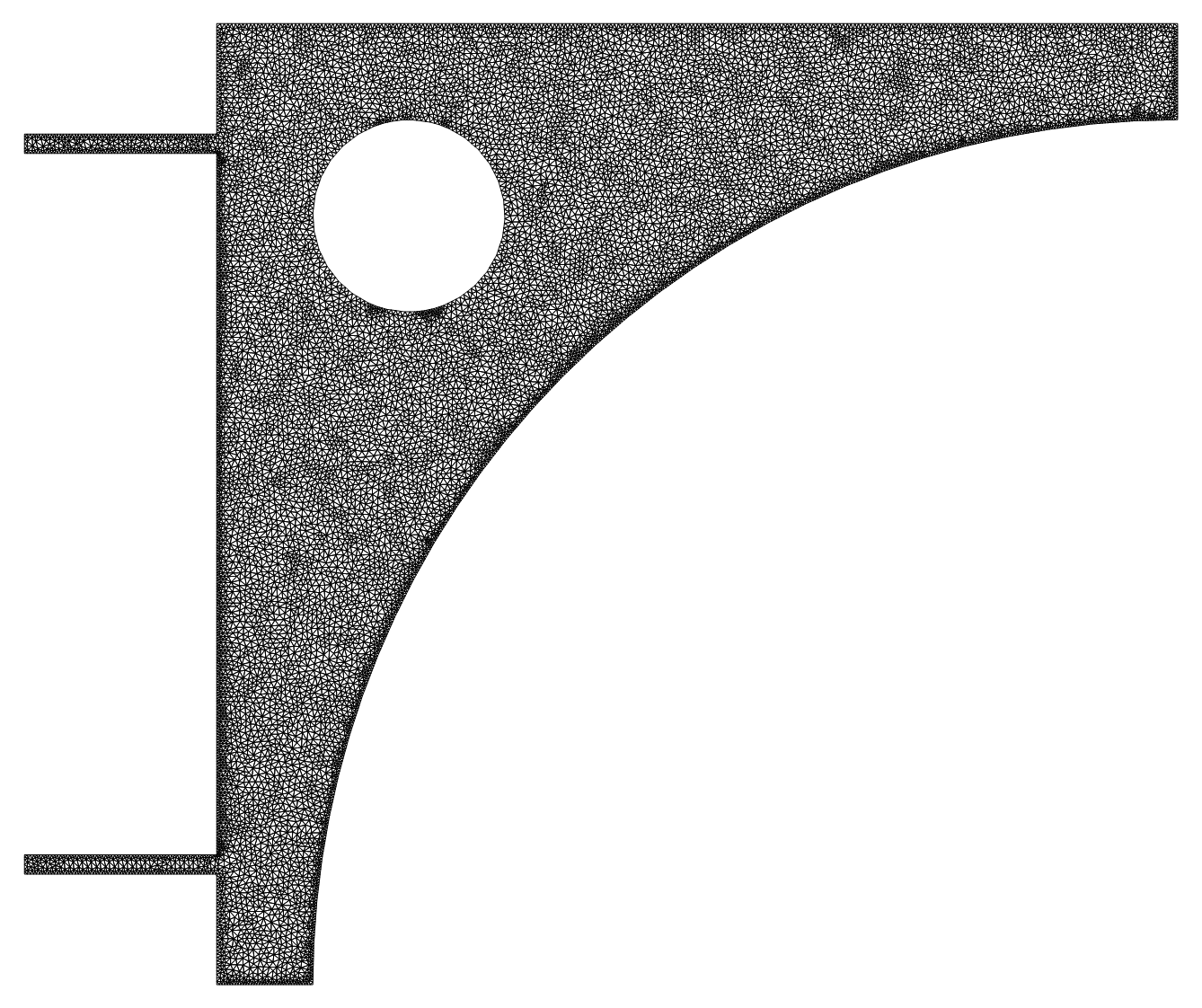
I am trying to make a finite element mesh in 2D. The features are not found by `ToElementMesh`. This is a shelf bracket with nails. I build the region using `RegionDifference` and `RegionUnion` and the result is clear.
Needs["NDSolve`FEM`"]
L = 0.2; (* Bracket side length *)
d = 0.02; (* End edge lenght *)
r = L - d; (* radius of curved edge *)
r1 = d; (* radius of hole *)
L2 = 0.004; (* nail thickness *)
L3 = 0.04; (* nail length *)
L4 = L/8; (* location of nail from bottom *)
Y = 10^3; (* modulus of elasticity *)
ν = 33/100; (* Poisson ratio *)
reg = RegionDifference[
RegionUnion[
Rectangle[{0, 0}, {L, L}],
Rectangle[{-L3, L4 - L2/2}, {0, L4 + L2/2}],
Rectangle[{-L3, (L - L4) - L2/2}, {0, (L - L4) + L2/2}]
],
RegionUnion[
Disk[{L, 0}, r],
Disk[{2 d, L - 2 d}, r1]]
];
Show[Region[reg], PlotRange -> All, Frame -> True]
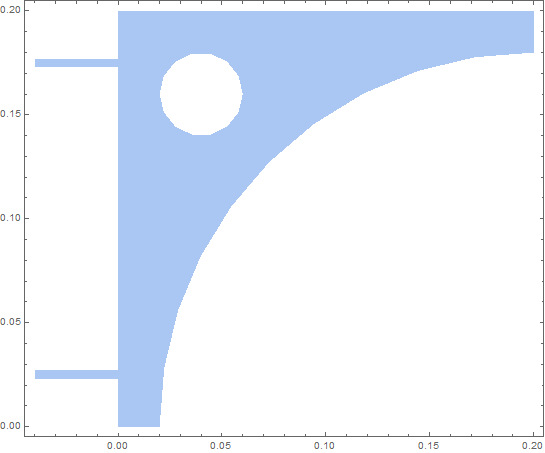
This region looks good. Now I try to mesh.
mesh = ToElementMesh[reg, "BoundaryMeshGenerator" -> "Continuation",
"MaxBoundaryCellMeasure" -> 0.001, "MaxCellMeasure" -> 0.0001];
Show[mesh["Wireframe"], PlotRange -> All]
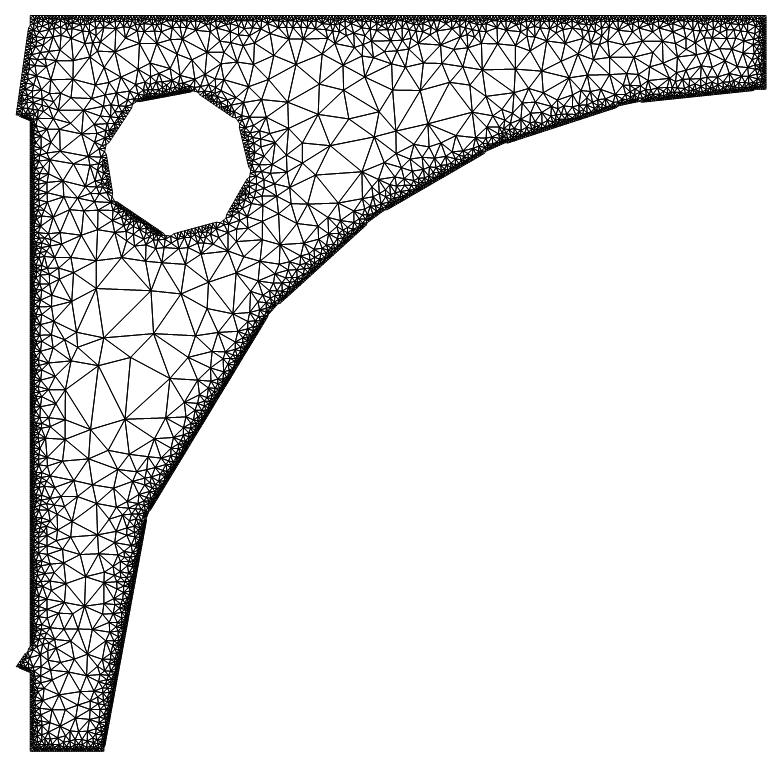
The nails have been lost and the edges are very poorly represented. I have tried different options for `ToElementMesh` but have not hit on one that works. What is happening? Version 11.1 for Windows. Thanks
**Edit**
Helpful user21 gave a solution below which works for the resolution in his example. I have tried to increase the resolution and this is what happens.
mesh = ToElementMesh[reg, {{-0.04`, 0.2`}, {0.`, 0.2`}},
"MaxBoundaryCellMeasure" -> 0.001,
"BoundaryMeshGenerator" -> {"RegionPlot", "SamplePoints" -> 200} ];
Show[mesh["Wireframe"], PlotRange -> All]
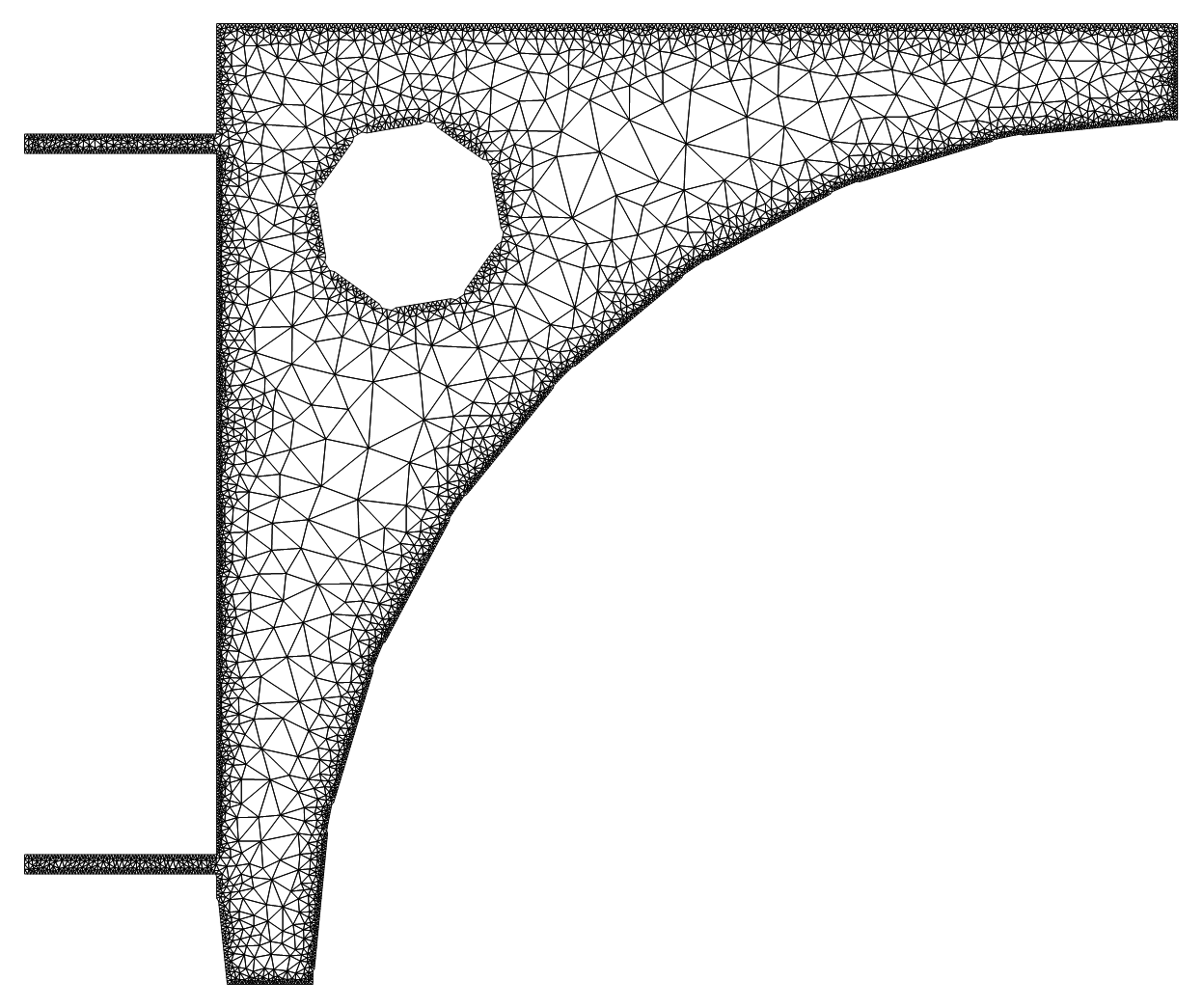
The curved boundaries and the lower part of the vertical straight boundary are badly formed. I would like a high resolution because when doing stress calculations gradients of the solution are needed. Any more thoughts?
Combining the suggestions from the answers below is successful.
**Further edit**
If you use this mesh you will need to use the workaround from [this question](https://mathematica.stackexchange.com/q/152455/12558). Add the option `"ImproveBoundaryPosition" -> False` to `ToElementMesh`. The mesh coordinates are not all contained within the solution interpolation function. So the final `ToElementMesh` expression was
mesh = ToElementMesh[reg, {{-L3, L}, {0, L}},
"MaxBoundaryCellMeasure" -> 0.001, MaxCellMeasure -> 1.1*^-6,
AccuracyGoal -> 8, MeshQualityGoal -> 1,
"BoundaryMeshGenerator" -> {"RegionPlot", "SamplePoints" -> 200},
"ImproveBoundaryPosition" -> False];
and it worked to give this mesh